homework 3 solving quadratics by square roots answers

• HW Guidelines
• Study Skills Quiz
• Find Local Tutors
• Demo MathHelp.com
• Join MathHelp.com

Select a Course Below

• ACCUPLACER Math
• Math Placement Test
• PRAXIS Math
• + more tests
• 5th Grade Math
• 6th Grade Math
• Pre-Algebra
• College Pre-Algebra
• Introductory Algebra
• Intermediate Algebra
• College Algebra

Solving Quadratic Equations by Taking Square Roots

Factoring Roots Completing the Square Formula Graphing Examples

Let's take another look at that last problem on the previous page:

Solve x 2 − 4 = 0

On the previous page, I'd solved this quadratic equation by factoring the difference of squares on the left-hand side of the equation, and then setting each factor equal to zero, etc, etc. The solution was " x  = ± 2 ". However—

I can also try isolating the squared-variable term on the left-hand side of the equation (that is, I can try getting the x 2 term by itself on one side of the "equals" sign), by moving the numerical part (that is, the 4 ) over to the right-hand side, like this:

Content Continues Below

MathHelp.com

Solving by Taking Square Roots

Advertisement

x 2 − 4 = 0

When I'm solving an equation, I know that I can do whatever I like to that equation as long as I do the exact same thing to both sides of that equation . On the left-hand side of this particular equation, I have an x 2 , and I want a plain old x . To turn the x 2 into an x , I can take the square root of each side of the equation, like this:

x = ± 2

Then the solution is x  = ±2 , just like it was when I solved by factoring the difference of squares.

Why did I need the " ± " (that is, the "plus-minus") sign on the 2 when I took the square root of the 4 ? Because I'm trying to find all values of the variable which make the original statement true, and it could have been either a positive 2 or a negative 2 that was squared to get that 4 in the original equation.

This duality is similar to how I'd had two factors, one "plus" and one "minus", when I used the difference-of-squares formula to solve this same equation on the previous page.

"Finding the solution to an equation" is a very different process from "evaluating the square root of a number". When finding "the" square root of a number, we're dealing exclusively with a positive value. Why? Because that is how the square root of a number is defined . The value of the square root of a number can only be positive, because that's how "the square root of a number" is defined.

Solving an equation, on the other hand — that is, finding all of the possible values of the variable that could work in an equation — is different from simply evaluating an expression that is already defined as having only one value.

Keep these two straight! A square-rooted number has only one value, but a square-rooted equation has two, because of the variable .

In mathematics, we need to be able to get the same answer, no matter which valid method we happen to have used in order to arrive at that answer. So, comparing the answer I got above with the answer I got one the previous page confirms that we must use the " ± " when taking square roots to solve.

(You may be doubting my work above in the step where I took the square root of either side, because I put a " ± " sign on only one side of the equation. Shouldn't I add this character to both sides of the equation? Kind of, yes. But if I'd put it on both sides of the equation, would anything really have changed? No. Try all the cases, if you're not sure.)

A benefit of this square-rooting process is that it allows us to solve some quadratics that we could not have solved before when using only factoring. For instance:

Solve x 2 − 50 = 0 .

This quadratic has a squared part and a numerical part. I'll start by adding the numerical term to the other side of the equaion (so the squared part is by itself), and then I'll square-root both sides. I'll need to remember to simplify the square root:

x 2 − 50 = 0

Then my solution is:

While we could have gotten the previous integer solution by factoring, we could never have gotten this radical solution by factoring. Factoring is clearly useful for solving some quadratic equations, but additional sorts of techniques allow us to find solutions to additional sorts of equations.

Solve ( x − 5) 2 − 100 = 0 .

This quadratic has a squared part and a numerical part. I'll start by adding the strictly-numerical term to the right-hand side of the equation, so that the squared binomial expression, containing the variable, is by itself on the left-hand side. Then I'll square-root both sides, remembering the " ± " on the numerical side, and then I'll simplify:

( x − 5) 2 − 100 = 0

( x − 5) 2 = 100

x − 5 = ±10

x = 5 ± 10

x = 5 − 10   or   x = 5 + 10

x = −5   or   x = 15

This equation, after taking the square root of either side, did not contain any radcials. Because of this, I was able to simplify my results, all the way down to simple values. My answer is:

x = −5, 15

The previous equation is an example of a equation where the careless student will omit the " ± " while solving, and will then have no clue as to how the book got the answer " x  = −5, 15 ".

These students get in the bad habit of not bothering to write the " ± " sign until they check their answers in the back of the book and suddenly "remember" that they "meant" to put the " ± " in there when they'd taken the square root of either side of the equation.

But this "magic" only works when you have the answer in the back (to remind you) and when the solution contains radicals (which doesn't always happen). In other cases, there will be no "reminder". Especially on tests, making the mistake of omitting the " ± " can be deadly. Don't be that student. Always remember to insert the " ± ".

By the way, since the solution to the previous equation consisted of integers, this quadratic could also have been solved by multiplying out the square, factoring, etc:

x 2 − 10 x + 25 − 100 = 0

x 2 − 10 x − 75 = 0

( x − 15)( x + 5) = 0

x − 15 = 0, x + 5 = 0

x = 15, −5

Solve ( x − 2) 2 − 12 = 0

This quadratic has a squared part and a numerical part. I'll add the numerical part over to the other side, so the squared part with the variable is by itself. Then I'll square-root both sides, remembering to add a " ± " to the numerical side, and then I'll simplify:

( x − 2) 2 − 12 = 0

( x − 2) 2 = 12

I can't simplify this any more. My answer is going to have radicals in it. My solution is:

This quadratic equation, unlike the one before it, could not have also been solved by factoring. But how would I have solved it, if they had not given me the quadratic already put into "(squared part) minus (a number part)" form? This concern leads to the next topic: solving by completing the square.

URL: https://www.purplemath.com/modules/solvquad2.htm

Page 1 Page 2 Page 3 Page 4 Page 5 Page 6

Standardized Test Prep

College math, homeschool math, share this page.

• Terms of Use
• About Purplemath
• About the Author
• Tutoring from PM
• Advertising
• Linking to PM
• Site licencing

Quadratic Equations Square Root Worksheets - Download free PDFs

Quadratic equations, square root, square: standard form, square: non-standard form, square: advanced form.

Please add a message.

Message received. Thanks for the feedback.

Child Login

• Kindergarten
• Number charts
• Skip Counting
• Place Value
• Number Lines
• Subtraction
• Multiplication
• Word Problems
• Comparing Numbers
• Ordering Numbers
• Odd and Even
• Prime and Composite
• Roman Numerals
• Ordinal Numbers
• In and Out Boxes
• Number System Conversions
• More Number Sense Worksheets
• Size Comparison
• Measuring Length
• Metric Unit Conversion
• Customary Unit Conversion
• Temperature
• More Measurement Worksheets
• Writing Checks
• Profit and Loss
• Simple Interest
• Compound Interest
• Tally Marks
• Mean, Median, Mode, Range
• Mean Absolute Deviation
• Stem-and-leaf Plot
• Box-and-whisker Plot
• Permutation and Combination
• Probability
• Venn Diagram
• More Statistics Worksheets
• Shapes - 2D
• Shapes - 3D
• Lines, Rays and Line Segments
• Points, Lines and Planes
• Transformation
• Quadrilateral
• Ordered Pairs
• Midpoint Formula
• Distance Formula
• Parallel, Perpendicular and Intersecting Lines
• Scale Factor
• Surface Area
• Pythagorean Theorem
• More Geometry Worksheets
• Converting between Fractions and Decimals
• Significant Figures
• Convert between Fractions, Decimals, and Percents
• Proportions
• Direct and Inverse Variation
• Order of Operations
• Squaring Numbers
• Square Roots
• Scientific Notations
• Speed, Distance, and Time
• Absolute Value
• More Pre-Algebra Worksheets
• Translating Algebraic Phrases
• Evaluating Algebraic Expressions
• Simplifying Algebraic Expressions
• Algebraic Identities
• Quadratic Equations
• Systems of Equations
• Polynomials
• Inequalities
• Sequence and Series
• Complex Numbers
• More Algebra Worksheets
• Trigonometry
• Math Workbooks
• English Language Arts
• Summer Review Packets
• Social Studies
• Holidays and Events
• Worksheets >
• Algebra >
• Equations >
• Quadratic Equations >
• Square Root Method

Solving Quadratic Equations by Taking Square Roots Worksheets

Unlike the standard form: ax 2 + bx + c = 0, most of the quadratic equations offered in this pack of printable high school worksheets have no middle term. Such equations are known as pure quadratic equations and are of the form ax 2 - c = 0. Solving these quadratic equations is made a lot easier by by taking square roots. Let's now get into the process! Here’s all you have to do. Isolate the leading term on the left-hand side of the equation and the constant term on the right-hand side, take square roots on both sides, and simplify both sides for the values of x. The equations that have the middle term can also be solved by finding square roots. Yet again, the middle term is excluded by using the appropriate algebraic identities. Get started with our free worksheet!

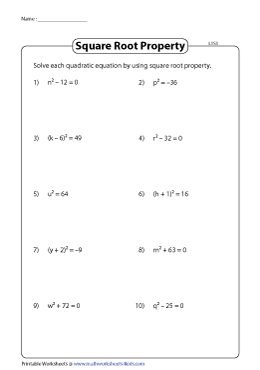
Solve Quadratic Equations by Taking Square Roots - Level 1

Push-start your practice of finding the real and complex roots of quadratic equations with this set of pdf worksheets presenting 30 pure quadratic equations. Note that the coefficient of the leading term is 1 in every equation. Hence, simply rewrite the given equation in the form of x 2 = c, and proceed to solve for x.

• Download the set

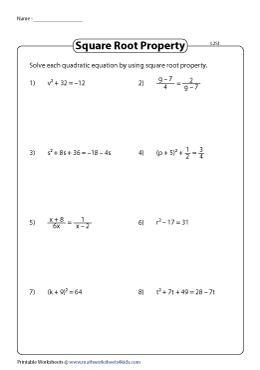
Solve Quadratic Equations by Taking Square Roots - Level 2

Keep one jump ahead of your peers with this printable practice set perfect for high school students! The quadratic equations here involve integers and fractions. You need to rewrite the equation to the desired form, isolate the x 2 term, take square roots, and perform simplification on both sides.

Related Worksheets

» Solving Equations | Completing the Squares

» Solving Quadratic Equations | Factoring

» Solving Equations | Quadratic Formula

» Sum and Product of the Roots

Become a Member

Membership Information

Privacy Policy

What's New?

Printing Help

Testimonial

Copyright © 2024 - Math Worksheets 4 Kids

This is a members-only feature!

If you're seeing this message, it means we're having trouble loading external resources on our website.

If you're behind a web filter, please make sure that the domains *.kastatic.org and *.kasandbox.org are unblocked.

To log in and use all the features of Khan Academy, please enable JavaScript in your browser.

Course: Algebra 1   >   Unit 14

• Solving quadratics by taking square roots
• Quadratics by taking square roots (intro)

Solving quadratics by taking square roots examples

• Quadratics by taking square roots
• Quadratics by taking square roots: strategy
• Solving quadratics by taking square roots: with steps
• Quadratics by taking square roots: with steps
• Solving simple quadratics review

Want to join the conversation?

• Upvote Button navigates to signup page
• Downvote Button navigates to signup page
• Flag Button navigates to signup page

Video transcript

• school Campus Bookshelves
• menu_book Bookshelves
• perm_media Learning Objects
• login Login
• how_to_reg Request Instructor Account
• hub Instructor Commons

Margin Size

• Download Page (PDF)
• Download Full Book (PDF)
• Periodic Table
• Physics Constants
• Scientific Calculator
• Reference & Cite
• Tools expand_more
• Readability

selected template will load here

This action is not available.

7.3: Solve Quadratic Equations Using the Quadratic Formula

• Last updated
• Save as PDF
• Page ID 56818

\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)

\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)

\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)

( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)

\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)

\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)

\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)

\( \newcommand{\Span}{\mathrm{span}}\)

\( \newcommand{\id}{\mathrm{id}}\)

\( \newcommand{\kernel}{\mathrm{null}\,}\)

\( \newcommand{\range}{\mathrm{range}\,}\)

\( \newcommand{\RealPart}{\mathrm{Re}}\)

\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)

\( \newcommand{\Argument}{\mathrm{Arg}}\)

\( \newcommand{\norm}[1]{\| #1 \|}\)

\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)

\( \newcommand{\vectorA}[1]{\vec{#1}}      % arrow\)

\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}}      % arrow\)

\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)

\( \newcommand{\vectorC}[1]{\textbf{#1}} \)

\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)

\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)

\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)

Learning Objectives

By the end of this section, you will be able to:

• Solve quadratic equations using the Quadratic Formula
• Use the discriminant to predict the number and type of solutions of a quadratic equation
• Identify the most appropriate method to use to solve a quadratic equation

Before you get started, take this readiness quiz.

• Evaluate \(b^{2}-4 a b\) when \(a=3\) and \(b=−2\).
• Simplify \(\sqrt{108}\).
• Simplify \(\sqrt{50}\).

Solve Quadratic Equations Using the Quadratic Formula

When we solved quadratic equations in the last section by completing the square, we took the same steps every time. By the end of the exercise set, you may have been wondering ‘isn’t there an easier way to do this?’ The answer is ‘yes’. Mathematicians look for patterns when they do things over and over in order to make their work easier. In this section we will derive and use a formula to find the solution of a quadratic equation.

We have already seen how to solve a formula for a specific variable ‘in general’, so that we would do the algebraic steps only once, and then use the new formula to find the value of the specific variable. Now we will go through the steps of completing the square using the general form of a quadratic equation to solve a quadratic equation for \(x\) .

We start with the standard form of a quadratic equation and solve it for \(x\) by completing the square.

The final equation is called the "Quadratic Formula."

Definition \(\PageIndex{1}\): Quadratic Formula

The solutions to a quadratic equation of the form \(a x^{2}+b x+c=0\), where \(a≠0\) are given by the formula:

\[x=\dfrac{-b \pm \sqrt{b^{2}-4 a c}}{2 a} \label{quad}\]

To use the Quadratic Formula , we substitute the values of \(a,b\), and \(c\) from the standard form into the expression on the right side of the formula. Then we simplify the expression. The result is the pair of solutions to the quadratic equation.

Notice the Quadratic Formula (Equation \ref{quad}) is an equation. Make sure you use both sides of the equation.

Example \(\PageIndex{1}\) How to Solve a Quadratic Equation Using the Quadratic Formula

Solve by using the Quadratic Formula: \(2 x^{2}+9 x-5=0\).

Exercise \(\PageIndex{1}\)

Solve by using the Quadratic Formula: \(3 y^{2}-5 y+2=0\).

\(y=1, y=\dfrac{2}{3}\)

Exercise \(\PageIndex{2}\)

Solve by using the Quadratic Formula: \(4 z^{2}+2 z-6=0\).

\(z=1, z=-\dfrac{3}{2}\)

HowTo: Solve a Quadratic Equation Using the Quadratic Formula

• Write the quadratic equation in standard form, \(a x^{2}+b x+c=0\). Identify the values of \(a,b\), and \(c\).
• Write the Quadratic Formula. Then substitute in the values of \(a,b\), and \(c\).
• Check the solutions.

If you say the formula as you write it in each problem, you’ll have it memorized in no time! And remember, the Quadratic Formula is an EQUATION. Be sure you start with “\(x=\)”.

Example \(\PageIndex{2}\)

Solve by using the Quadratic Formula: \(x^{2}-6 x=-5\).

Exercise \(\PageIndex{3}\)

Solve by using the Quadratic Formula: \(a^{2}-2 a=15\).

\(a=-3, a=5\)

Exercise \(\PageIndex{4}\)

Solve by using the Quadratic Formula: \(b^{2}+24=-10 b\).

\(b=-6, b=-4\)

When we solved quadratic equations by using the Square Root Property, we sometimes got answers that had radicals. That can happen, too, when using the Quadratic Formula . If we get a radical as a solution, the final answer must have the radical in its simplified form.

Example \(\PageIndex{3}\)

Solve by using the Quadratic Formula: \(2 x^{2}+10 x+11=0\).

Exercise \(\PageIndex{5}\)

Solve by using the Quadratic Formula: \(3 m^{2}+12 m+7=0\).

\(m=\dfrac{-6+\sqrt{15}}{3}, m=\dfrac{-6-\sqrt{15}}{3}\)

Exercise \(\PageIndex{6}\)

Solve by using the Quadratic Formula: \(5 n^{2}+4 n-4=0\).

\(n=\dfrac{-2+2 \sqrt{6}}{5}, n=\dfrac{-2-2 \sqrt{6}}{5}\)

When we substitute \(a, b\), and \(c\) into the Quadratic Formula and the radicand is negative, the quadratic equation will have imaginary or complex solutions. We will see this in the next example.

Example \(\PageIndex{4}\)

Solve by using the Quadratic Formula: \(3 p^{2}+2 p+9=0\).

Exercise \(\PageIndex{7}\)

Solve by using the Quadratic Formula: \(4 a^{2}-2 a+8=0\).

\(a=\dfrac{1}{4}+\dfrac{\sqrt{31}}{4} i, \quad a=\dfrac{1}{4}-\dfrac{\sqrt{31}}{4} i\)

Exercise \(\PageIndex{8}\)

Solve by using the Quadratic Formula: \(5 b^{2}+2 b+4=0\).

\(b=-\dfrac{1}{5}+\dfrac{\sqrt{19}}{5} i, \quad b=-\dfrac{1}{5}-\dfrac{\sqrt{19}}{5} i\)

Remember, to use the Quadratic Formula, the equation must be written in standard form, \(a x^{2}+b x+c=0\). Sometimes, we will need to do some algebra to get the equation into standard form before we can use the Quadratic Formula.

Example \(\PageIndex{5}\)

Solve by using the Quadratic Formula: \(x(x+6)+4=0\).

Our first step is to get the equation in standard form.

Exercise \(\PageIndex{9}\)

Solve by using the Quadratic Formula: \(x(x+2)−5=0\).

\(x=-1+\sqrt{6}, x=-1-\sqrt{6}\)

Exercise \(\PageIndex{10}\)

Solve by using the Quadratic Formula: \(3y(y−2)−3=0\).

\(y=1+\sqrt{2}, y=1-\sqrt{2}\)

When we solved linear equations, if an equation had too many fractions we cleared the fractions by multiplying both sides of the equation by the LCD. This gave us an equivalent equation—without fractions— to solve. We can use the same strategy with quadratic equations.

Example \(\PageIndex{6}\)

Solve by using the Quadratic Formula: \(\dfrac{1}{2} u^{2}+\dfrac{2}{3} u=\dfrac{1}{3}\).

Our first step is to clear the fractions.

Exercise \(\PageIndex{11}\)

Solve by using the Quadratic Formula: \(\dfrac{1}{4} c^{2}-\dfrac{1}{3} c=\dfrac{1}{12}\).

\(c=\dfrac{2+\sqrt{7}}{3}, \quad c=\dfrac{2-\sqrt{7}}{3}\)

Exercise \(\PageIndex{12}\)

Solve by using the Quadratic Formula: \(\dfrac{1}{9} d^{2}-\dfrac{1}{2} d=-\dfrac{1}{3}\).

\(d=\dfrac{9+\sqrt{33}}{4}, d=\dfrac{9-\sqrt{33}}{4}\)

Think about the equation \((x-3)^{2}=0\). We know from the Zero Product Property that this equation has only one solution, \(x=3\).

We will see in the next example how using the Quadratic Formula to solve an equation whose standard form is a perfect square trinomial equal to \(0\) gives just one solution. Notice that once the radicand is simplified it becomes \(0\), which leads to only one solution.

Example \(\PageIndex{7}\)

Solve by using the Quadratic Formula: \(4 x^{2}-20 x=-25\).

Did you recognize that \(4 x^{2}-20 x+25\) is a perfect square trinomial. It is equivalent to \((2 x-5)^{2}\)? If you solve \(4 x^{2}-20 x+25=0\) by factoring and then using the Square Root Property, do you get the same result?

Exercise \(\PageIndex{13}\)

Solve by using the Quadratic Formula: \(r^{2}+10 r+25=0\).

Exercise \(\PageIndex{14}\)

Solve by using the Quadratic Formula: \(25 t^{2}-40 t=-16\).

\(t=\dfrac{4}{5}\)

Use the Discriminant to Predict the Number and Type of Solutions of a Quadratic Equation

When we solved the quadratic equations in the previous examples, sometimes we got two real solutions, one real solution, and sometimes two complex solutions. Is there a way to predict the number and type of solutions to a quadratic equation without actually solving the equation?

Yes, the expression under the radical of the Quadratic Formula makes it easy for us to determine the number and type of solutions. This expression is called the discriminant .

Definition \(\PageIndex{2}\)

Discriminant

Let’s look at the discriminant of the equations in some of the examples and the number and type of solutions to those quadratic equations.

Using the Discriminant \(b^{2}-4ac\), to Determine the Number and Type of Solutions of a Quadratic Equation

For a quadratic equation of the form \(ax^{2}+bx+c=0\), \(a \neq 0\),

• If \(b^{2}-4 a c>0\), the equation has \(2\) real solutions.
• if \(b^{2}-4 a c=0\), the equation has \(1\) real solution.
• if \(b^{2}-4 a c<0\), the equation has \(2\) complex solutions.

Example \(\PageIndex{8}\)

Determine the number of solutions to each quadratic equation.

\(3 x^{2}+7 x-9=0\)

\(5 n^{2}+n+4=0\)

\(9 y^{2}-6 y+1=0\)

To determine the number of solutions of each quadratic equation, we will look at its discriminant.

The equation is in standard form, identify \(a, b\), and \(c\).

\(a=3, \quad b=7, \quad c=-9\)

Write the discriminant.

\(b^{2}-4 a c\)

Substitute in the values of \(a, b\), and \(c\).

\((7)^{2}-4 \cdot 3 \cdot(-9)\)

\(49+108\) \(157\)

Since the discriminant is positive, there are \(2\) real solutions to the equation.

\(a=5, \quad b=1, \quad c=4\)

\((1)^{2}-4 \cdot 5 \cdot 4\)

\(1-80\) \(-79\)

Since the discriminant is negative, there are \(2\) complex solutions to the equation.

\(a=9, \quad b=-6, \quad c=1\)

\((-6)^{2}-4 \cdot 9 \cdot 1\)

\(36-36\) \(0\)

Since the discriminant is \(0\), there is \(1\) real solution to the equation.

Exercise \(\PageIndex{15}\)

Determine the number and type of solutions to each quadratic equation.

• \(8 m^{2}-3 m+6=0\)
• \(5 z^{2}+6 z-2=0\)
• \(9 w^{2}+24 w+16=0\)
• \(2\) complex solutions
• \(2\) real solutions
• \(1\) real solution

Exercise \(\PageIndex{16}\)

• \(b^{2}+7 b-13=0\)
• \(5 a^{2}-6 a+10=0\)
• \(4 r^{2}-20 r+25=0\)

Identify the Most Appropriate Method to Use to Solve a Quadratic Equation

We summarize the four methods that we have used to solve quadratic equations below.

Methods for Solving Quadratic Equations

• Square Root Property
• Completing the Square
• Quadratic Formula

Given that we have four methods to use to solve a quadratic equation, how do you decide which one to use? Factoring is often the quickest method and so we try it first. If the equation is \(ax^{2}=k\) or \(a(x−h)^{2}=k\) we use the Square Root Property. For any other equation, it is probably best to use the Quadratic Formula. Remember, you can solve any quadratic equation by using the Quadratic Formula, but that is not always the easiest method.

What about the method of Completing the Square? Most people find that method cumbersome and prefer not to use it. We needed to include it in the list of methods because we completed the square in general to derive the Quadratic Formula. You will also use the process of Completing the Square in other areas of algebra.

Identify the Most Appropriate Method to Solve a Quadratic Equation

• Try Factoring first. If the quadratic factors easily, this method is very quick.
• Try the Square Root Property next. If the equation fits the form \(ax^{2}=k\) or \(a(x−h)^{2}=k\), it can easily be solved by using the Square Root Property.
• Use the Quadratic Formula . Any other quadratic equation is best solved by using the Quadratic Formula.

The next example uses this strategy to decide how to solve each quadratic equation.

Example \(\PageIndex{9}\)

Identify the most appropriate method to use to solve each quadratic equation.

• \(5 z^{2}=17\)

\(4 x^{2}-12 x+9=0\)

\(8 u^{2}+6 u=11\)

\(5z^{2}=17\)

Since the equation is in the \(ax^{2}=k\), the most appropriate method is to use the Square Root Property.

We recognize that the left side of the equation is a perfect square trinomial, and so factoring will be the most appropriate method.

Put the equation in standard form.

\(8 u^{2}+6 u-11=0\)

While our first thought may be to try factoring, thinking about all the possibilities for trial and error method leads us to choose the Quadratic Formula as the most appropriate method.

Exercise \(\PageIndex{17}\)

• \(x^{2}+6 x+8=0\)
• \((n-3)^{2}=16\)
• \(5 p^{2}-6 p=9\)

Exercise \(\PageIndex{18}\)

• \(8 a^{2}+3 a-9=0\)
• \(4 b^{2}+4 b+1=0\)
• \(5 c^{2}=125\)
• Factoring or Square Root Property

Access these online resources for additional instruction and practice with using the Quadratic Formula.

• Using the Quadratic Formula
• Solve a Quadratic Equation Using the Quadratic Formula with Complex Solutions
• Discriminant in Quadratic Formula

Key Concepts

\(x=\dfrac{-b \pm \sqrt{b^{2}-4 a c}}{2 a}\)

• Write the quadratic equation in standard form, \(a x^{2}+b x+c=0\). Identify the values of \(a, b, c\).
• Write the Quadratic Formula. Then substitute in the values of \(a, b, c\).
• If \(b^{2}-4 a c=0\), the equation has \(1\) real solution.
• If \(b^{2}-4 a c<0\), the equation has \(2\) complex solutions.
• Try the Square Root Property next. If the equation fits the form \(a x^{2}=k\) or \(a(x-h)^{2}=k\), it can easily be solved by using the Square Root Property.
• Use the Quadratic Formula. Any other quadratic equation is best solved by using the Quadratic Formula.

Quadratic Equation Solver

What do you want to calculate.

• Solve for Variable
• Practice Mode
• Step-By-Step

Step-By-Step Example

Example (click to try), choose your method, solve by factoring.

Example: 3x^2-2x-1=0

Complete The Square

Example: 3x^2-2x-1=0 (After you click the example, change the Method to 'Solve By Completing the Square'.)

Take the Square Root

Example: 2x^2=18

Quadratic Formula

Example: 4x^2-2x-1=0

About quadratic equations

Need more problem types? Try MathPapa Algebra Calculator

Clear Quadratic Equation Solver »