- Skip to secondary menu
- Skip to main content
- Skip to primary sidebar
Statistics By Jim
Making statistics intuitive

Null Hypothesis: Definition, Rejecting & Examples
By Jim Frost 6 Comments
What is a Null Hypothesis?
The null hypothesis in statistics states that there is no difference between groups or no relationship between variables. It is one of two mutually exclusive hypotheses about a population in a hypothesis test.

- Null Hypothesis H 0 : No effect exists in the population.
- Alternative Hypothesis H A : The effect exists in the population.
In every study or experiment, researchers assess an effect or relationship. This effect can be the effectiveness of a new drug, building material, or other intervention that has benefits. There is a benefit or connection that the researchers hope to identify. Unfortunately, no effect may exist. In statistics, we call this lack of an effect the null hypothesis. Researchers assume that this notion of no effect is correct until they have enough evidence to suggest otherwise, similar to how a trial presumes innocence.
In this context, the analysts don’t necessarily believe the null hypothesis is correct. In fact, they typically want to reject it because that leads to more exciting finds about an effect or relationship. The new vaccine works!
You can think of it as the default theory that requires sufficiently strong evidence to reject. Like a prosecutor, researchers must collect sufficient evidence to overturn the presumption of no effect. Investigators must work hard to set up a study and a data collection system to obtain evidence that can reject the null hypothesis.
Related post : What is an Effect in Statistics?
Null Hypothesis Examples
Null hypotheses start as research questions that the investigator rephrases as a statement indicating there is no effect or relationship.
| Does the vaccine prevent infections? | The vaccine does not affect the infection rate. |
| Does the new additive increase product strength? | The additive does not affect mean product strength. |
| Does the exercise intervention increase bone mineral density? | The intervention does not affect bone mineral density. |
| As screen time increases, does test performance decrease? | There is no relationship between screen time and test performance. |
After reading these examples, you might think they’re a bit boring and pointless. However, the key is to remember that the null hypothesis defines the condition that the researchers need to discredit before suggesting an effect exists.
Let’s see how you reject the null hypothesis and get to those more exciting findings!
When to Reject the Null Hypothesis
So, you want to reject the null hypothesis, but how and when can you do that? To start, you’ll need to perform a statistical test on your data. The following is an overview of performing a study that uses a hypothesis test.
The first step is to devise a research question and the appropriate null hypothesis. After that, the investigators need to formulate an experimental design and data collection procedures that will allow them to gather data that can answer the research question. Then they collect the data. For more information about designing a scientific study that uses statistics, read my post 5 Steps for Conducting Studies with Statistics .
After data collection is complete, statistics and hypothesis testing enter the picture. Hypothesis testing takes your sample data and evaluates how consistent they are with the null hypothesis. The p-value is a crucial part of the statistical results because it quantifies how strongly the sample data contradict the null hypothesis.
When the sample data provide sufficient evidence, you can reject the null hypothesis. In a hypothesis test, this process involves comparing the p-value to your significance level .
Rejecting the Null Hypothesis
Reject the null hypothesis when the p-value is less than or equal to your significance level. Your sample data favor the alternative hypothesis, which suggests that the effect exists in the population. For a mnemonic device, remember—when the p-value is low, the null must go!
When you can reject the null hypothesis, your results are statistically significant. Learn more about Statistical Significance: Definition & Meaning .
Failing to Reject the Null Hypothesis
Conversely, when the p-value is greater than your significance level, you fail to reject the null hypothesis. The sample data provides insufficient data to conclude that the effect exists in the population. When the p-value is high, the null must fly!
Note that failing to reject the null is not the same as proving it. For more information about the difference, read my post about Failing to Reject the Null .
That’s a very general look at the process. But I hope you can see how the path to more exciting findings depends on being able to rule out the less exciting null hypothesis that states there’s nothing to see here!
Let’s move on to learning how to write the null hypothesis for different types of effects, relationships, and tests.
Related posts : How Hypothesis Tests Work and Interpreting P-values
How to Write a Null Hypothesis
The null hypothesis varies by the type of statistic and hypothesis test. Remember that inferential statistics use samples to draw conclusions about populations. Consequently, when you write a null hypothesis, it must make a claim about the relevant population parameter . Further, that claim usually indicates that the effect does not exist in the population. Below are typical examples of writing a null hypothesis for various parameters and hypothesis tests.
Related posts : Descriptive vs. Inferential Statistics and Populations, Parameters, and Samples in Inferential Statistics
Group Means
T-tests and ANOVA assess the differences between group means. For these tests, the null hypothesis states that there is no difference between group means in the population. In other words, the experimental conditions that define the groups do not affect the mean outcome. Mu (µ) is the population parameter for the mean, and you’ll need to include it in the statement for this type of study.
For example, an experiment compares the mean bone density changes for a new osteoporosis medication. The control group does not receive the medicine, while the treatment group does. The null states that the mean bone density changes for the control and treatment groups are equal.
- Null Hypothesis H 0 : Group means are equal in the population: µ 1 = µ 2 , or µ 1 – µ 2 = 0
- Alternative Hypothesis H A : Group means are not equal in the population: µ 1 ≠ µ 2 , or µ 1 – µ 2 ≠ 0.
Group Proportions
Proportions tests assess the differences between group proportions. For these tests, the null hypothesis states that there is no difference between group proportions. Again, the experimental conditions did not affect the proportion of events in the groups. P is the population proportion parameter that you’ll need to include.
For example, a vaccine experiment compares the infection rate in the treatment group to the control group. The treatment group receives the vaccine, while the control group does not. The null states that the infection rates for the control and treatment groups are equal.
- Null Hypothesis H 0 : Group proportions are equal in the population: p 1 = p 2 .
- Alternative Hypothesis H A : Group proportions are not equal in the population: p 1 ≠ p 2 .
Correlation and Regression Coefficients
Some studies assess the relationship between two continuous variables rather than differences between groups.
In these studies, analysts often use either correlation or regression analysis . For these tests, the null states that there is no relationship between the variables. Specifically, it says that the correlation or regression coefficient is zero. As one variable increases, there is no tendency for the other variable to increase or decrease. Rho (ρ) is the population correlation parameter and beta (β) is the regression coefficient parameter.
For example, a study assesses the relationship between screen time and test performance. The null states that there is no correlation between this pair of variables. As screen time increases, test performance does not tend to increase or decrease.
- Null Hypothesis H 0 : The correlation in the population is zero: ρ = 0.
- Alternative Hypothesis H A : The correlation in the population is not zero: ρ ≠ 0.
For all these cases, the analysts define the hypotheses before the study. After collecting the data, they perform a hypothesis test to determine whether they can reject the null hypothesis.
The preceding examples are all for two-tailed hypothesis tests. To learn about one-tailed tests and how to write a null hypothesis for them, read my post One-Tailed vs. Two-Tailed Tests .
Related post : Understanding Correlation
Neyman, J; Pearson, E. S. (January 1, 1933). On the Problem of the most Efficient Tests of Statistical Hypotheses . Philosophical Transactions of the Royal Society A . 231 (694–706): 289–337.
Share this:

Reader Interactions
January 11, 2024 at 2:57 pm
Thanks for the reply.
January 10, 2024 at 1:23 pm
Hi Jim, In your comment you state that equivalence test null and alternate hypotheses are reversed. For hypothesis tests of data fits to a probability distribution, the null hypothesis is that the probability distribution fits the data. Is this correct?
January 10, 2024 at 2:15 pm
Those two separate things, equivalence testing and normality tests. But, yes, you’re correct for both.
Hypotheses are switched for equivalence testing. You need to “work” (i.e., collect a large sample of good quality data) to be able to reject the null that the groups are different to be able to conclude they’re the same.
With typical hypothesis tests, if you have low quality data and a low sample size, you’ll fail to reject the null that they’re the same, concluding they’re equivalent. But that’s more a statement about the low quality and small sample size than anything to do with the groups being equal.
So, equivalence testing make you work to obtain a finding that the groups are the same (at least within some amount you define as a trivial difference).
For normality testing, and other distribution tests, the null states that the data follow the distribution (normal or whatever). If you reject the null, you have sufficient evidence to conclude that your sample data don’t follow the probability distribution. That’s a rare case where you hope to fail to reject the null. And it suffers from the problem I describe above where you might fail to reject the null simply because you have a small sample size. In that case, you’d conclude the data follow the probability distribution but it’s more that you don’t have enough data for the test to register the deviation. In this scenario, if you had a larger sample size, you’d reject the null and conclude it doesn’t follow that distribution.
I don’t know of any equivalence testing type approach for distribution fit tests where you’d need to work to show the data follow a distribution, although I haven’t looked for one either!
February 20, 2022 at 9:26 pm
Is a null hypothesis regularly (always) stated in the negative? “there is no” or “does not”
February 23, 2022 at 9:21 pm
Typically, the null hypothesis includes an equal sign. The null hypothesis states that the population parameter equals a particular value. That value is usually one that represents no effect. In the case of a one-sided hypothesis test, the null still contains an equal sign but it’s “greater than or equal to” or “less than or equal to.” If you wanted to translate the null hypothesis from its native mathematical expression, you could use the expression “there is no effect.” But the mathematical form more specifically states what it’s testing.
It’s the alternative hypothesis that typically contains does not equal.
There are some exceptions. For example, in an equivalence test where the researchers want to show that two things are equal, the null hypothesis states that they’re not equal.
In short, the null hypothesis states the condition that the researchers hope to reject. They need to work hard to set up an experiment and data collection that’ll gather enough evidence to be able to reject the null condition.
February 15, 2022 at 9:32 am
Dear sir I always read your notes on Research methods.. Kindly tell is there any available Book on all these..wonderfull Urgent
Comments and Questions Cancel reply
- Science, Tech, Math ›
- Chemistry ›
- Chemical Laws ›
Null Hypothesis Definition and Examples
PM Images / Getty Images
- Chemical Laws
- Periodic Table
- Projects & Experiments
- Scientific Method
- Biochemistry
- Physical Chemistry
- Medical Chemistry
- Chemistry In Everyday Life
- Famous Chemists
- Activities for Kids
- Abbreviations & Acronyms
- Weather & Climate
- Ph.D., Biomedical Sciences, University of Tennessee at Knoxville
- B.A., Physics and Mathematics, Hastings College
In a scientific experiment, the null hypothesis is the proposition that there is no effect or no relationship between phenomena or populations. If the null hypothesis is true, any observed difference in phenomena or populations would be due to sampling error (random chance) or experimental error. The null hypothesis is useful because it can be tested and found to be false, which then implies that there is a relationship between the observed data. It may be easier to think of it as a nullifiable hypothesis or one that the researcher seeks to nullify. The null hypothesis is also known as the H 0, or no-difference hypothesis.
The alternate hypothesis, H A or H 1 , proposes that observations are influenced by a non-random factor. In an experiment, the alternate hypothesis suggests that the experimental or independent variable has an effect on the dependent variable .
How to State a Null Hypothesis
There are two ways to state a null hypothesis. One is to state it as a declarative sentence, and the other is to present it as a mathematical statement.
For example, say a researcher suspects that exercise is correlated to weight loss, assuming diet remains unchanged. The average length of time to achieve a certain amount of weight loss is six weeks when a person works out five times a week. The researcher wants to test whether weight loss takes longer to occur if the number of workouts is reduced to three times a week.
The first step to writing the null hypothesis is to find the (alternate) hypothesis. In a word problem like this, you're looking for what you expect to be the outcome of the experiment. In this case, the hypothesis is "I expect weight loss to take longer than six weeks."
This can be written mathematically as: H 1 : μ > 6
In this example, μ is the average.
Now, the null hypothesis is what you expect if this hypothesis does not happen. In this case, if weight loss isn't achieved in greater than six weeks, then it must occur at a time equal to or less than six weeks. This can be written mathematically as:
H 0 : μ ≤ 6
The other way to state the null hypothesis is to make no assumption about the outcome of the experiment. In this case, the null hypothesis is simply that the treatment or change will have no effect on the outcome of the experiment. For this example, it would be that reducing the number of workouts would not affect the time needed to achieve weight loss:
H 0 : μ = 6
Null Hypothesis Examples
"Hyperactivity is unrelated to eating sugar " is an example of a null hypothesis. If the hypothesis is tested and found to be false, using statistics, then a connection between hyperactivity and sugar ingestion may be indicated. A significance test is the most common statistical test used to establish confidence in a null hypothesis.
Another example of a null hypothesis is "Plant growth rate is unaffected by the presence of cadmium in the soil ." A researcher could test the hypothesis by measuring the growth rate of plants grown in a medium lacking cadmium, compared with the growth rate of plants grown in mediums containing different amounts of cadmium. Disproving the null hypothesis would set the groundwork for further research into the effects of different concentrations of the element in soil.
Why Test a Null Hypothesis?
You may be wondering why you would want to test a hypothesis just to find it false. Why not just test an alternate hypothesis and find it true? The short answer is that it is part of the scientific method. In science, propositions are not explicitly "proven." Rather, science uses math to determine the probability that a statement is true or false. It turns out it's much easier to disprove a hypothesis than to positively prove one. Also, while the null hypothesis may be simply stated, there's a good chance the alternate hypothesis is incorrect.
For example, if your null hypothesis is that plant growth is unaffected by duration of sunlight, you could state the alternate hypothesis in several different ways. Some of these statements might be incorrect. You could say plants are harmed by more than 12 hours of sunlight or that plants need at least three hours of sunlight, etc. There are clear exceptions to those alternate hypotheses, so if you test the wrong plants, you could reach the wrong conclusion. The null hypothesis is a general statement that can be used to develop an alternate hypothesis, which may or may not be correct.
- Kelvin Temperature Scale Definition
- Independent Variable Definition and Examples
- Theory Definition in Science
- Hypothesis Definition (Science)
- de Broglie Equation Definition
- Law of Combining Volumes Definition
- Chemical Definition
- Pure Substance Definition in Chemistry
- Acid Definition and Examples
- Extensive Property Definition (Chemistry)
- Radiation Definition and Examples
- Valence Definition in Chemistry
- Atomic Solid Definition
- Weak Base Definition and Examples
- Oxidation Definition and Example in Chemistry
- Definition of Binary Compound
- Math Article
Null Hypothesis

In mathematics, Statistics deals with the study of research and surveys on the numerical data. For taking surveys, we have to define the hypothesis. Generally, there are two types of hypothesis. One is a null hypothesis, and another is an alternative hypothesis .
In probability and statistics, the null hypothesis is a comprehensive statement or default status that there is zero happening or nothing happening. For example, there is no connection among groups or no association between two measured events. It is generally assumed here that the hypothesis is true until any other proof has been brought into the light to deny the hypothesis. Let us learn more here with definition, symbol, principle, types and example, in this article.
Table of contents:
- Comparison with Alternative Hypothesis
Null Hypothesis Definition
The null hypothesis is a kind of hypothesis which explains the population parameter whose purpose is to test the validity of the given experimental data. This hypothesis is either rejected or not rejected based on the viability of the given population or sample . In other words, the null hypothesis is a hypothesis in which the sample observations results from the chance. It is said to be a statement in which the surveyors wants to examine the data. It is denoted by H 0 .
Null Hypothesis Symbol
In statistics, the null hypothesis is usually denoted by letter H with subscript ‘0’ (zero), such that H 0 . It is pronounced as H-null or H-zero or H-nought. At the same time, the alternative hypothesis expresses the observations determined by the non-random cause. It is represented by H 1 or H a .
Null Hypothesis Principle
The principle followed for null hypothesis testing is, collecting the data and determining the chances of a given set of data during the study on some random sample, assuming that the null hypothesis is true. In case if the given data does not face the expected null hypothesis, then the outcome will be quite weaker, and they conclude by saying that the given set of data does not provide strong evidence against the null hypothesis because of insufficient evidence. Finally, the researchers tend to reject that.
Null Hypothesis Formula
Here, the hypothesis test formulas are given below for reference.
The formula for the null hypothesis is:
H 0 : p = p 0
The formula for the alternative hypothesis is:
H a = p >p 0 , < p 0 ≠ p 0
The formula for the test static is:
Remember that, p 0 is the null hypothesis and p – hat is the sample proportion.
Also, read:
Types of Null Hypothesis
There are different types of hypothesis. They are:
Simple Hypothesis
It completely specifies the population distribution. In this method, the sampling distribution is the function of the sample size.
Composite Hypothesis
The composite hypothesis is one that does not completely specify the population distribution.
Exact Hypothesis
Exact hypothesis defines the exact value of the parameter. For example μ= 50
Inexact Hypothesis
This type of hypothesis does not define the exact value of the parameter. But it denotes a specific range or interval. For example 45< μ <60
Null Hypothesis Rejection
Sometimes the null hypothesis is rejected too. If this hypothesis is rejected means, that research could be invalid. Many researchers will neglect this hypothesis as it is merely opposite to the alternate hypothesis. It is a better practice to create a hypothesis and test it. The goal of researchers is not to reject the hypothesis. But it is evident that a perfect statistical model is always associated with the failure to reject the null hypothesis.
How do you Find the Null Hypothesis?
The null hypothesis says there is no correlation between the measured event (the dependent variable) and the independent variable. We don’t have to believe that the null hypothesis is true to test it. On the contrast, you will possibly assume that there is a connection between a set of variables ( dependent and independent).
When is Null Hypothesis Rejected?
The null hypothesis is rejected using the P-value approach. If the P-value is less than or equal to the α, there should be a rejection of the null hypothesis in favour of the alternate hypothesis. In case, if P-value is greater than α, the null hypothesis is not rejected.
Null Hypothesis and Alternative Hypothesis
Now, let us discuss the difference between the null hypothesis and the alternative hypothesis.
|
|
| |
| 1 | The null hypothesis is a statement. There exists no relation between two variables | Alternative hypothesis a statement, there exists some relationship between two measured phenomenon |
| 2 | Denoted by H | Denoted by H |
| 3 | The observations of this hypothesis are the result of chance | The observations of this hypothesis are the result of real effect |
| 4 | The mathematical formulation of the null hypothesis is an equal sign | The mathematical formulation alternative hypothesis is an inequality sign such as greater than, less than, etc. |
Null Hypothesis Examples
Here, some of the examples of the null hypothesis are given below. Go through the below ones to understand the concept of the null hypothesis in a better way.
If a medicine reduces the risk of cardiac stroke, then the null hypothesis should be “the medicine does not reduce the chance of cardiac stroke”. This testing can be performed by the administration of a drug to a certain group of people in a controlled way. If the survey shows that there is a significant change in the people, then the hypothesis is rejected.
Few more examples are:
1). Are there is 100% chance of getting affected by dengue?
Ans: There could be chances of getting affected by dengue but not 100%.
2). Do teenagers are using mobile phones more than grown-ups to access the internet?
Ans: Age has no limit on using mobile phones to access the internet.
3). Does having apple daily will not cause fever?
Ans: Having apple daily does not assure of not having fever, but increases the immunity to fight against such diseases.
4). Do the children more good in doing mathematical calculations than grown-ups?
Ans: Age has no effect on Mathematical skills.
In many common applications, the choice of the null hypothesis is not automated, but the testing and calculations may be automated. Also, the choice of the null hypothesis is completely based on previous experiences and inconsistent advice. The choice can be more complicated and based on the variety of applications and the diversity of the objectives.
The main limitation for the choice of the null hypothesis is that the hypothesis suggested by the data is based on the reasoning which proves nothing. It means that if some hypothesis provides a summary of the data set, then there would be no value in the testing of the hypothesis on the particular set of data.
Frequently Asked Questions on Null Hypothesis
What is meant by the null hypothesis.
In Statistics, a null hypothesis is a type of hypothesis which explains the population parameter whose purpose is to test the validity of the given experimental data.
What are the benefits of hypothesis testing?
Hypothesis testing is defined as a form of inferential statistics, which allows making conclusions from the entire population based on the sample representative.
When a null hypothesis is accepted and rejected?
The null hypothesis is either accepted or rejected in terms of the given data. If P-value is less than α, then the null hypothesis is rejected in favor of the alternative hypothesis, and if the P-value is greater than α, then the null hypothesis is accepted in favor of the alternative hypothesis.
Why is the null hypothesis important?
The importance of the null hypothesis is that it provides an approximate description of the phenomena of the given data. It allows the investigators to directly test the relational statement in a research study.
How to accept or reject the null hypothesis in the chi-square test?
If the result of the chi-square test is bigger than the critical value in the table, then the data does not fit the model, which represents the rejection of the null hypothesis.

Put your understanding of this concept to test by answering a few MCQs. Click ‘Start Quiz’ to begin!
Select the correct answer and click on the “Finish” button Check your score and answers at the end of the quiz
Visit BYJU’S for all Maths related queries and study materials
Your result is as below
Request OTP on Voice Call
| MATHS Related Links | |
Register with BYJU'S & Download Free PDFs
Register with byju's & watch live videos.
Null Hypothesis Definition and Examples, How to State
What is the null hypothesis, how to state the null hypothesis, null hypothesis overview.
Why is it Called the “Null”?
The word “null” in this context means that it’s a commonly accepted fact that researchers work to nullify . It doesn’t mean that the statement is null (i.e. amounts to nothing) itself! (Perhaps the term should be called the “nullifiable hypothesis” as that might cause less confusion).
Why Do I need to Test it? Why not just prove an alternate one?
The short answer is, as a scientist, you are required to ; It’s part of the scientific process. Science uses a battery of processes to prove or disprove theories, making sure than any new hypothesis has no flaws. Including both a null and an alternate hypothesis is one safeguard to ensure your research isn’t flawed. Not including the null hypothesis in your research is considered very bad practice by the scientific community. If you set out to prove an alternate hypothesis without considering it, you are likely setting yourself up for failure. At a minimum, your experiment will likely not be taken seriously.
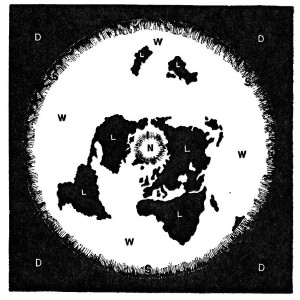
- Null hypothesis : H 0 : The world is flat.
- Alternate hypothesis: The world is round.
Several scientists, including Copernicus , set out to disprove the null hypothesis. This eventually led to the rejection of the null and the acceptance of the alternate. Most people accepted it — the ones that didn’t created the Flat Earth Society !. What would have happened if Copernicus had not disproved the it and merely proved the alternate? No one would have listened to him. In order to change people’s thinking, he first had to prove that their thinking was wrong .
How to State the Null Hypothesis from a Word Problem
You’ll be asked to convert a word problem into a hypothesis statement in statistics that will include a null hypothesis and an alternate hypothesis . Breaking your problem into a few small steps makes these problems much easier to handle.

Step 2: Convert the hypothesis to math . Remember that the average is sometimes written as μ.
H 1 : μ > 8.2
Broken down into (somewhat) English, that’s H 1 (The hypothesis): μ (the average) > (is greater than) 8.2
Step 3: State what will happen if the hypothesis doesn’t come true. If the recovery time isn’t greater than 8.2 weeks, there are only two possibilities, that the recovery time is equal to 8.2 weeks or less than 8.2 weeks.
H 0 : μ ≤ 8.2
Broken down again into English, that’s H 0 (The null hypothesis): μ (the average) ≤ (is less than or equal to) 8.2
How to State the Null Hypothesis: Part Two
But what if the researcher doesn’t have any idea what will happen.
Example Problem: A researcher is studying the effects of radical exercise program on knee surgery patients. There is a good chance the therapy will improve recovery time, but there’s also the possibility it will make it worse. Average recovery times for knee surgery patients is 8.2 weeks.
Step 1: State what will happen if the experiment doesn’t make any difference. That’s the null hypothesis–that nothing will happen. In this experiment, if nothing happens, then the recovery time will stay at 8.2 weeks.
H 0 : μ = 8.2
Broken down into English, that’s H 0 (The null hypothesis): μ (the average) = (is equal to) 8.2
Step 2: Figure out the alternate hypothesis . The alternate hypothesis is the opposite of the null hypothesis. In other words, what happens if our experiment makes a difference?
H 1 : μ ≠ 8.2
In English again, that’s H 1 (The alternate hypothesis): μ (the average) ≠ (is not equal to) 8.2
That’s How to State the Null Hypothesis!
Check out our Youtube channel for more stats tips!
Gonick, L. (1993). The Cartoon Guide to Statistics . HarperPerennial. Kotz, S.; et al., eds. (2006), Encyclopedia of Statistical Sciences , Wiley.
9.1 Null and Alternative Hypotheses
The actual test begins by considering two hypotheses . They are called the null hypothesis and the alternative hypothesis . These hypotheses contain opposing viewpoints.
H 0 , the — null hypothesis: a statement of no difference between sample means or proportions or no difference between a sample mean or proportion and a population mean or proportion. In other words, the difference equals 0.
H a —, the alternative hypothesis: a claim about the population that is contradictory to H 0 and what we conclude when we reject H 0 .
Since the null and alternative hypotheses are contradictory, you must examine evidence to decide if you have enough evidence to reject the null hypothesis or not. The evidence is in the form of sample data.
After you have determined which hypothesis the sample supports, you make a decision. There are two options for a decision. They are reject H 0 if the sample information favors the alternative hypothesis or do not reject H 0 or decline to reject H 0 if the sample information is insufficient to reject the null hypothesis.
Mathematical Symbols Used in H 0 and H a :
| equal (=) | not equal (≠) greater than (>) less than (<) |
| greater than or equal to (≥) | less than (<) |
| less than or equal to (≤) | more than (>) |
H 0 always has a symbol with an equal in it. H a never has a symbol with an equal in it. The choice of symbol depends on the wording of the hypothesis test. However, be aware that many researchers use = in the null hypothesis, even with > or < as the symbol in the alternative hypothesis. This practice is acceptable because we only make the decision to reject or not reject the null hypothesis.
Example 9.1
H 0 : No more than 30 percent of the registered voters in Santa Clara County voted in the primary election. p ≤ 30 H a : More than 30 percent of the registered voters in Santa Clara County voted in the primary election. p > 30
A medical trial is conducted to test whether or not a new medicine reduces cholesterol by 25 percent. State the null and alternative hypotheses.
Example 9.2
We want to test whether the mean GPA of students in American colleges is different from 2.0 (out of 4.0). The null and alternative hypotheses are the following: H 0 : μ = 2.0 H a : μ ≠ 2.0
We want to test whether the mean height of eighth graders is 66 inches. State the null and alternative hypotheses. Fill in the correct symbol (=, ≠, ≥, <, ≤, >) for the null and alternative hypotheses.
- H 0 : μ __ 66
- H a : μ __ 66
Example 9.3
We want to test if college students take fewer than five years to graduate from college, on the average. The null and alternative hypotheses are the following: H 0 : μ ≥ 5 H a : μ < 5
We want to test if it takes fewer than 45 minutes to teach a lesson plan. State the null and alternative hypotheses. Fill in the correct symbol ( =, ≠, ≥, <, ≤, >) for the null and alternative hypotheses.
- H 0 : μ __ 45
- H a : μ __ 45
Example 9.4
An article on school standards stated that about half of all students in France, Germany, and Israel take advanced placement exams and a third of the students pass. The same article stated that 6.6 percent of U.S. students take advanced placement exams and 4.4 percent pass. Test if the percentage of U.S. students who take advanced placement exams is more than 6.6 percent. State the null and alternative hypotheses. H 0 : p ≤ 0.066 H a : p > 0.066
On a state driver’s test, about 40 percent pass the test on the first try. We want to test if more than 40 percent pass on the first try. Fill in the correct symbol (=, ≠, ≥, <, ≤, >) for the null and alternative hypotheses.
- H 0 : p __ 0.40
- H a : p __ 0.40
Collaborative Exercise
Bring to class a newspaper, some news magazines, and some internet articles. In groups, find articles from which your group can write null and alternative hypotheses. Discuss your hypotheses with the rest of the class.
This book may not be used in the training of large language models or otherwise be ingested into large language models or generative AI offerings without OpenStax's permission.
Want to cite, share, or modify this book? This book uses the Creative Commons Attribution License and you must attribute Texas Education Agency (TEA). The original material is available at: https://www.texasgateway.org/book/tea-statistics . Changes were made to the original material, including updates to art, structure, and other content updates.
Access for free at https://openstax.org/books/statistics/pages/1-introduction
- Authors: Barbara Illowsky, Susan Dean
- Publisher/website: OpenStax
- Book title: Statistics
- Publication date: Mar 27, 2020
- Location: Houston, Texas
- Book URL: https://openstax.org/books/statistics/pages/1-introduction
- Section URL: https://openstax.org/books/statistics/pages/9-1-null-and-alternative-hypotheses
© Apr 16, 2024 Texas Education Agency (TEA). The OpenStax name, OpenStax logo, OpenStax book covers, OpenStax CNX name, and OpenStax CNX logo are not subject to the Creative Commons license and may not be reproduced without the prior and express written consent of Rice University.
Have a thesis expert improve your writing
Check your thesis for plagiarism in 10 minutes, generate your apa citations for free.
- Knowledge Base
- Null and Alternative Hypotheses | Definitions & Examples
Null and Alternative Hypotheses | Definitions & Examples
Published on 5 October 2022 by Shaun Turney . Revised on 6 December 2022.
The null and alternative hypotheses are two competing claims that researchers weigh evidence for and against using a statistical test :
- Null hypothesis (H 0 ): There’s no effect in the population .
- Alternative hypothesis (H A ): There’s an effect in the population.
The effect is usually the effect of the independent variable on the dependent variable .
Table of contents
Answering your research question with hypotheses, what is a null hypothesis, what is an alternative hypothesis, differences between null and alternative hypotheses, how to write null and alternative hypotheses, frequently asked questions about null and alternative hypotheses.
The null and alternative hypotheses offer competing answers to your research question . When the research question asks “Does the independent variable affect the dependent variable?”, the null hypothesis (H 0 ) answers “No, there’s no effect in the population.” On the other hand, the alternative hypothesis (H A ) answers “Yes, there is an effect in the population.”
The null and alternative are always claims about the population. That’s because the goal of hypothesis testing is to make inferences about a population based on a sample . Often, we infer whether there’s an effect in the population by looking at differences between groups or relationships between variables in the sample.
You can use a statistical test to decide whether the evidence favors the null or alternative hypothesis. Each type of statistical test comes with a specific way of phrasing the null and alternative hypothesis. However, the hypotheses can also be phrased in a general way that applies to any test.
The null hypothesis is the claim that there’s no effect in the population.
If the sample provides enough evidence against the claim that there’s no effect in the population ( p ≤ α), then we can reject the null hypothesis . Otherwise, we fail to reject the null hypothesis.
Although “fail to reject” may sound awkward, it’s the only wording that statisticians accept. Be careful not to say you “prove” or “accept” the null hypothesis.
Null hypotheses often include phrases such as “no effect”, “no difference”, or “no relationship”. When written in mathematical terms, they always include an equality (usually =, but sometimes ≥ or ≤).
Examples of null hypotheses
The table below gives examples of research questions and null hypotheses. There’s always more than one way to answer a research question, but these null hypotheses can help you get started.
| ( ) | ||
| Does tooth flossing affect the number of cavities? | Tooth flossing has on the number of cavities. | test: The mean number of cavities per person does not differ between the flossing group (µ ) and the non-flossing group (µ ) in the population; µ = µ . |
| Does the amount of text highlighted in the textbook affect exam scores? | The amount of text highlighted in the textbook has on exam scores. | : There is no relationship between the amount of text highlighted and exam scores in the population; β = 0. |
| Does daily meditation decrease the incidence of depression? | Daily meditation the incidence of depression.* | test: The proportion of people with depression in the daily-meditation group ( ) is greater than or equal to the no-meditation group ( ) in the population; ≥ . |
*Note that some researchers prefer to always write the null hypothesis in terms of “no effect” and “=”. It would be fine to say that daily meditation has no effect on the incidence of depression and p 1 = p 2 .
The alternative hypothesis (H A ) is the other answer to your research question . It claims that there’s an effect in the population.
Often, your alternative hypothesis is the same as your research hypothesis. In other words, it’s the claim that you expect or hope will be true.
The alternative hypothesis is the complement to the null hypothesis. Null and alternative hypotheses are exhaustive, meaning that together they cover every possible outcome. They are also mutually exclusive, meaning that only one can be true at a time.
Alternative hypotheses often include phrases such as “an effect”, “a difference”, or “a relationship”. When alternative hypotheses are written in mathematical terms, they always include an inequality (usually ≠, but sometimes > or <). As with null hypotheses, there are many acceptable ways to phrase an alternative hypothesis.
Examples of alternative hypotheses
The table below gives examples of research questions and alternative hypotheses to help you get started with formulating your own.
| Does tooth flossing affect the number of cavities? | Tooth flossing has an on the number of cavities. | test: The mean number of cavities per person differs between the flossing group (µ ) and the non-flossing group (µ ) in the population; µ ≠ µ . |
| Does the amount of text highlighted in a textbook affect exam scores? | The amount of text highlighted in the textbook has an on exam scores. | : There is a relationship between the amount of text highlighted and exam scores in the population; β ≠ 0. |
| Does daily meditation decrease the incidence of depression? | Daily meditation the incidence of depression. | test: The proportion of people with depression in the daily-meditation group ( ) is less than the no-meditation group ( ) in the population; < . |
Null and alternative hypotheses are similar in some ways:
- They’re both answers to the research question
- They both make claims about the population
- They’re both evaluated by statistical tests.
However, there are important differences between the two types of hypotheses, summarized in the following table.
| A claim that there is in the population. | A claim that there is in the population. | |
|
| ||
| Equality symbol (=, ≥, or ≤) | Inequality symbol (≠, <, or >) | |
| Rejected | Supported | |
| Failed to reject | Not supported |
To help you write your hypotheses, you can use the template sentences below. If you know which statistical test you’re going to use, you can use the test-specific template sentences. Otherwise, you can use the general template sentences.
The only thing you need to know to use these general template sentences are your dependent and independent variables. To write your research question, null hypothesis, and alternative hypothesis, fill in the following sentences with your variables:
Does independent variable affect dependent variable ?
- Null hypothesis (H 0 ): Independent variable does not affect dependent variable .
- Alternative hypothesis (H A ): Independent variable affects dependent variable .
Test-specific
Once you know the statistical test you’ll be using, you can write your hypotheses in a more precise and mathematical way specific to the test you chose. The table below provides template sentences for common statistical tests.
| ( ) | ||
| test
with two groups | The mean dependent variable does not differ between group 1 (µ ) and group 2 (µ ) in the population; µ = µ . | The mean dependent variable differs between group 1 (µ ) and group 2 (µ ) in the population; µ ≠ µ . |
| with three groups | The mean dependent variable does not differ between group 1 (µ ), group 2 (µ ), and group 3 (µ ) in the population; µ = µ = µ . | The mean dependent variable of group 1 (µ ), group 2 (µ ), and group 3 (µ ) are not all equal in the population. |
| There is no correlation between independent variable and dependent variable in the population; ρ = 0. | There is a correlation between independent variable and dependent variable in the population; ρ ≠ 0. | |
| There is no relationship between independent variable and dependent variable in the population; β = 0. | There is a relationship between independent variable and dependent variable in the population; β ≠ 0. | |
| Two-proportions test | The dependent variable expressed as a proportion does not differ between group 1 ( ) and group 2 ( ) in the population; = . | The dependent variable expressed as a proportion differs between group 1 ( ) and group 2 ( ) in the population; ≠ . |
Note: The template sentences above assume that you’re performing one-tailed tests . One-tailed tests are appropriate for most studies.
The null hypothesis is often abbreviated as H 0 . When the null hypothesis is written using mathematical symbols, it always includes an equality symbol (usually =, but sometimes ≥ or ≤).
The alternative hypothesis is often abbreviated as H a or H 1 . When the alternative hypothesis is written using mathematical symbols, it always includes an inequality symbol (usually ≠, but sometimes < or >).
A research hypothesis is your proposed answer to your research question. The research hypothesis usually includes an explanation (‘ x affects y because …’).
A statistical hypothesis, on the other hand, is a mathematical statement about a population parameter. Statistical hypotheses always come in pairs: the null and alternative hypotheses. In a well-designed study , the statistical hypotheses correspond logically to the research hypothesis.
Cite this Scribbr article
If you want to cite this source, you can copy and paste the citation or click the ‘Cite this Scribbr article’ button to automatically add the citation to our free Reference Generator.
Turney, S. (2022, December 06). Null and Alternative Hypotheses | Definitions & Examples. Scribbr. Retrieved 15 October 2024, from https://www.scribbr.co.uk/stats/null-and-alternative-hypothesis/
Is this article helpful?

Shaun Turney
Other students also liked, levels of measurement: nominal, ordinal, interval, ratio, the standard normal distribution | calculator, examples & uses, types of variables in research | definitions & examples.

- Science Notes Posts
- Contact Science Notes
- Todd Helmenstine Biography
- Anne Helmenstine Biography
- Free Printable Periodic Tables (PDF and PNG)
- Periodic Table Wallpapers
- Interactive Periodic Table
- Periodic Table Posters
- Science Experiments for Kids
- How to Grow Crystals
- Chemistry Projects
- Fire and Flames Projects
- Holiday Science
- Chemistry Problems With Answers
- Physics Problems
- Unit Conversion Example Problems
- Chemistry Worksheets
- Biology Worksheets
- Periodic Table Worksheets
- Physical Science Worksheets
- Science Lab Worksheets
- My Amazon Books
Null Hypothesis Examples

The null hypothesis (H 0 ) is the hypothesis that states there is no statistical difference between two sample sets. In other words, it assumes the independent variable does not have an effect on the dependent variable in a scientific experiment .
The null hypothesis is the most powerful type of hypothesis in the scientific method because it’s the easiest one to test with a high confidence level using statistics. If the null hypothesis is accepted, then it’s evidence any observed differences between two experiment groups are due to random chance. If the null hypothesis is rejected, then it’s strong evidence there is a true difference between test sets or that the independent variable affects the dependent variable.
- The null hypothesis is a nullifiable hypothesis. A researcher seeks to reject it because this result strongly indicates observed differences are real and not just due to chance.
- The null hypothesis may be accepted or rejected, but not proven. There is always a level of confidence in the outcome.
What Is the Null Hypothesis?
The null hypothesis is written as H 0 , which is read as H-zero, H-nought, or H-null. It is associated with another hypothesis, called the alternate or alternative hypothesis H A or H 1 . When the null hypothesis and alternate hypothesis are written mathematically, they cover all possible outcomes of an experiment.
An experimenter tests the null hypothesis with a statistical analysis called a significance test. The significance test determines the likelihood that the results of the test are not due to chance. Usually, a researcher uses a confidence level of 95% or 99% (p-value of 0.05 or 0.01). But, even if the confidence in the test is high, there is always a small chance the outcome is incorrect. This means you can’t prove a null hypothesis. It’s also a good reason why it’s important to repeat experiments.
Exact and Inexact Null Hypothesis
The most common type of null hypothesis assumes no difference between two samples or groups or no measurable effect of a treatment. This is the exact hypothesis . If you’re asked to state a null hypothesis for a science class, this is the one to write. It is the easiest type of hypothesis to test and is the only one accepted for certain types of analysis. Examples include:
There is no difference between two groups H 0 : μ 1 = μ 2 (where H 0 = the null hypothesis, μ 1 = the mean of population 1, and μ 2 = the mean of population 2)
Both groups have value of 100 (or any number or quality) H 0 : μ = 100
However, sometimes a researcher may test an inexact hypothesis . This type of hypothesis specifies ranges or intervals. Examples include:
Recovery time from a treatment is the same or worse than a placebo: H 0 : μ ≥ placebo time
There is a 5% or less difference between two groups: H 0 : 95 ≤ μ ≤ 105
An inexact hypothesis offers “directionality” about a phenomenon. For example, an exact hypothesis can indicate whether or not a treatment has an effect, while an inexact hypothesis can tell whether an effect is positive of negative. However, an inexact hypothesis may be harder to test and some scientists and statisticians disagree about whether it’s a true null hypothesis .
How to State the Null Hypothesis
To state the null hypothesis, first state what you expect the experiment to show. Then, rephrase the statement in a form that assumes there is no relationship between the variables or that a treatment has no effect.
Example: A researcher tests whether a new drug speeds recovery time from a certain disease. The average recovery time without treatment is 3 weeks.
- State the goal of the experiment: “I hope the average recovery time with the new drug will be less than 3 weeks.”
- Rephrase the hypothesis to assume the treatment has no effect: “If the drug doesn’t shorten recovery time, then the average time will be 3 weeks or longer.” Mathematically: H 0 : μ ≥ 3
This null hypothesis (inexact hypothesis) covers both the scenario in which the drug has no effect and the one in which the drugs makes the recovery time longer. The alternate hypothesis is that average recovery time will be less than three weeks:
H A : μ < 3
Of course, the researcher could test the no-effect hypothesis (exact null hypothesis): H 0 : μ = 3
The danger of testing this hypothesis is that rejecting it only implies the drug affected recovery time (not whether it made it better or worse). This is because the alternate hypothesis is:
H A : μ ≠ 3 (which includes μ <3 and μ >3)
Even though the no-effect null hypothesis yields less information, it’s used because it’s easier to test using statistics. Basically, testing whether something is unchanged/changed is easier than trying to quantify the nature of the change.
Remember, a researcher hopes to reject the null hypothesis because this supports the alternate hypothesis. Also, be sure the null and alternate hypothesis cover all outcomes. Finally, remember a simple true/false, equal/unequal, yes/no exact hypothesis is easier to test than a more complex inexact hypothesis.
| Does chewing willow bark relieve pain? | Pain relief is the same compared with a . (exact) Pain relief after chewing willow bark is the same or worse versus taking a placebo. (inexact) | Pain relief is different compared with a placebo. (exact) Pain relief is better compared to a placebo. (inexact) |
| Do cats care about the shape of their food? | Cats show no food preference based on shape. (exact) | Cat show a food preference based on shape. (exact) |
| Do teens use mobile devices more than adults? | Teens and adults use mobile devices the same amount. (exact) Teens use mobile devices less than or equal to adults. (inexact) | Teens and adults used mobile devices different amounts. (exact) Teens use mobile devices more than adults. (inexact) |
| Does the color of light influence plant growth? | The color of light has no effect on plant growth. (exact) | The color of light affects plant growth. (exact) |
- Adèr, H. J.; Mellenbergh, G. J. & Hand, D. J. (2007). Advising on Research Methods: A Consultant’s Companion . Huizen, The Netherlands: Johannes van Kessel Publishing. ISBN 978-90-79418-01-5 .
- Cox, D. R. (2006). Principles of Statistical Inference . Cambridge University Press. ISBN 978-0-521-68567-2 .
- Everitt, Brian (1998). The Cambridge Dictionary of Statistics . Cambridge, UK New York: Cambridge University Press. ISBN 978-0521593465.
- Weiss, Neil A. (1999). Introductory Statistics (5th ed.). ISBN 9780201598773.
Related Posts
Have a language expert improve your writing
Run a free plagiarism check in 10 minutes, generate accurate citations for free.
- Knowledge Base
Hypothesis Testing | A Step-by-Step Guide with Easy Examples
Published on November 8, 2019 by Rebecca Bevans . Revised on June 22, 2023.
Hypothesis testing is a formal procedure for investigating our ideas about the world using statistics . It is most often used by scientists to test specific predictions, called hypotheses, that arise from theories.
There are 5 main steps in hypothesis testing:
- State your research hypothesis as a null hypothesis and alternate hypothesis (H o ) and (H a or H 1 ).
- Collect data in a way designed to test the hypothesis.
- Perform an appropriate statistical test .
- Decide whether to reject or fail to reject your null hypothesis.
- Present the findings in your results and discussion section.
Though the specific details might vary, the procedure you will use when testing a hypothesis will always follow some version of these steps.
Table of contents
Step 1: state your null and alternate hypothesis, step 2: collect data, step 3: perform a statistical test, step 4: decide whether to reject or fail to reject your null hypothesis, step 5: present your findings, other interesting articles, frequently asked questions about hypothesis testing.
After developing your initial research hypothesis (the prediction that you want to investigate), it is important to restate it as a null (H o ) and alternate (H a ) hypothesis so that you can test it mathematically.
The alternate hypothesis is usually your initial hypothesis that predicts a relationship between variables. The null hypothesis is a prediction of no relationship between the variables you are interested in.
- H 0 : Men are, on average, not taller than women. H a : Men are, on average, taller than women.
Prevent plagiarism. Run a free check.
For a statistical test to be valid , it is important to perform sampling and collect data in a way that is designed to test your hypothesis. If your data are not representative, then you cannot make statistical inferences about the population you are interested in.
There are a variety of statistical tests available, but they are all based on the comparison of within-group variance (how spread out the data is within a category) versus between-group variance (how different the categories are from one another).
If the between-group variance is large enough that there is little or no overlap between groups, then your statistical test will reflect that by showing a low p -value . This means it is unlikely that the differences between these groups came about by chance.
Alternatively, if there is high within-group variance and low between-group variance, then your statistical test will reflect that with a high p -value. This means it is likely that any difference you measure between groups is due to chance.
Your choice of statistical test will be based on the type of variables and the level of measurement of your collected data .
- an estimate of the difference in average height between the two groups.
- a p -value showing how likely you are to see this difference if the null hypothesis of no difference is true.
Based on the outcome of your statistical test, you will have to decide whether to reject or fail to reject your null hypothesis.
In most cases you will use the p -value generated by your statistical test to guide your decision. And in most cases, your predetermined level of significance for rejecting the null hypothesis will be 0.05 – that is, when there is a less than 5% chance that you would see these results if the null hypothesis were true.
In some cases, researchers choose a more conservative level of significance, such as 0.01 (1%). This minimizes the risk of incorrectly rejecting the null hypothesis ( Type I error ).
Receive feedback on language, structure, and formatting
Professional editors proofread and edit your paper by focusing on:
- Academic style
- Vague sentences
- Style consistency
See an example
The results of hypothesis testing will be presented in the results and discussion sections of your research paper , dissertation or thesis .
In the results section you should give a brief summary of the data and a summary of the results of your statistical test (for example, the estimated difference between group means and associated p -value). In the discussion , you can discuss whether your initial hypothesis was supported by your results or not.
In the formal language of hypothesis testing, we talk about rejecting or failing to reject the null hypothesis. You will probably be asked to do this in your statistics assignments.
However, when presenting research results in academic papers we rarely talk this way. Instead, we go back to our alternate hypothesis (in this case, the hypothesis that men are on average taller than women) and state whether the result of our test did or did not support the alternate hypothesis.
If your null hypothesis was rejected, this result is interpreted as “supported the alternate hypothesis.”
These are superficial differences; you can see that they mean the same thing.
You might notice that we don’t say that we reject or fail to reject the alternate hypothesis . This is because hypothesis testing is not designed to prove or disprove anything. It is only designed to test whether a pattern we measure could have arisen spuriously, or by chance.
If we reject the null hypothesis based on our research (i.e., we find that it is unlikely that the pattern arose by chance), then we can say our test lends support to our hypothesis . But if the pattern does not pass our decision rule, meaning that it could have arisen by chance, then we say the test is inconsistent with our hypothesis .
If you want to know more about statistics , methodology , or research bias , make sure to check out some of our other articles with explanations and examples.
- Normal distribution
- Descriptive statistics
- Measures of central tendency
- Correlation coefficient
Methodology
- Cluster sampling
- Stratified sampling
- Types of interviews
- Cohort study
- Thematic analysis
Research bias
- Implicit bias
- Cognitive bias
- Survivorship bias
- Availability heuristic
- Nonresponse bias
- Regression to the mean
Hypothesis testing is a formal procedure for investigating our ideas about the world using statistics. It is used by scientists to test specific predictions, called hypotheses , by calculating how likely it is that a pattern or relationship between variables could have arisen by chance.
A hypothesis states your predictions about what your research will find. It is a tentative answer to your research question that has not yet been tested. For some research projects, you might have to write several hypotheses that address different aspects of your research question.
A hypothesis is not just a guess — it should be based on existing theories and knowledge. It also has to be testable, which means you can support or refute it through scientific research methods (such as experiments, observations and statistical analysis of data).
Null and alternative hypotheses are used in statistical hypothesis testing . The null hypothesis of a test always predicts no effect or no relationship between variables, while the alternative hypothesis states your research prediction of an effect or relationship.
Cite this Scribbr article
If you want to cite this source, you can copy and paste the citation or click the “Cite this Scribbr article” button to automatically add the citation to our free Citation Generator.
Bevans, R. (2023, June 22). Hypothesis Testing | A Step-by-Step Guide with Easy Examples. Scribbr. Retrieved October 16, 2024, from https://www.scribbr.com/statistics/hypothesis-testing/
Is this article helpful?
Rebecca Bevans
Other students also liked, choosing the right statistical test | types & examples, understanding p values | definition and examples, what is your plagiarism score.
Mastering Python’s Set Difference: A Game-Changer for Data Wrangling
Reading list
Basics of machine learning, machine learning lifecycle, importance of stats and eda, understanding data, probability, exploring continuous variable, exploring categorical variables, missing values and outliers, central limit theorem, bivariate analysis introduction, continuous - continuous variables, continuous categorical, categorical categorical, multivariate analysis, different tasks in machine learning, build your first predictive model, evaluation metrics, preprocessing data, linear models, selecting the right model, feature selection techniques, decision tree, feature engineering, naive bayes, multiclass and multilabel, basics of ensemble techniques, advance ensemble techniques, hyperparameter tuning, support vector machine, advance dimensionality reduction, unsupervised machine learning methods, recommendation engines, improving ml models, working with large datasets, interpretability of machine learning models, automated machine learning, model deployment, deploying ml models, embedded devices, a simple guide to hypothesis testing for dummies.
This article was published as a part of the Data Science Blogathon
Introduction
Statistics is the science of analyzing huge amounts of data. In the real world, it is nearly impossible to deduce statistics about the entire population. And this huge amount of data needs interpretation to draw meaningful conclusions. Hence, we take some random samples from the population, derive some statistical measures (e.g. mean, standard deviation, variance), and draw conclusions about relationships from the data collected.
Data can be interpreted by assuming a specific outcome and use statistical methods to confirm or reject the assumption. This assumption is called a hypothesis and the statistical test used for this purpose is called hypothesis testing .
In statistics, a hypothesis is a statement about a population that we want to verify based on information contained in the sample data.
Hypothesis testing quantifies an observation or outcome of an experiment under a given assumption. The result of the test enables us to interpret whether the assumption holds true or false. In other words, it signifies if the hypothesis can be confirmed or rejected for the observation made.
An observation or outcome of an experiment is known as a test statistic , which is a statistic measure or a standardized value that is calculated from sample data of the underlying population.
The assumption of hypothesis testing is called the null hypothesis. A null hypothesis is a type of assumption used in statistics indicating that there is no significant difference between the samples from the underlying population. It is also known as the default hypothesis, represented by H 0 . In contrast, there is a term “Alternative Hypothesis”, represented by H 1 . For every null hypothesis, there is an alternative hypothesis that is opposite to what the null hypothesis states. In other words, if we reject the null hypothesis, we fail to reject the alternative hypothesis.
The decision of confirming or rejecting the null hypothesis is made by interpreting the result of the test. The result of the hypothesis testing can be interpreted using p-values or critical values. The p-value is the probability of deducing the observed value, given the assumption. On the other hand, critical values are cut-off values that define regions where the test statistic is unlikely to lie.
Perform Hypothesis Testing
Hypothesis testing can be performed using these 3 steps:
1. Design a test statistic
2. Design a null hypothesis
3. Compute the p-value or critical values
How about looking at this coin-toss example to see how is hypothetical testing performed?
Given a coin, determine if the coin is biased towards heads or not.
What do you mean by saying a coin is biased towards heads? This means that the probability of getting heads is greater than 0.5 i.e. P(H) > 0.5.
Design an experiment
Flip a coin 5 times, and count the number of heads.
Consider the count of the number of heads as a random variable ‘X’. In the theory of statistical hypothesis testing, it is known as a test statistic i.e. X = 5.
Perform the experiment
We flip the coin 5 times.
There are 32 such observations. Out of these 32 possibilities, there is only one observation that has 5 heads. Hence, the test statistic is X = 5.
Now, compute the probability that X = 5, when the coin is not biased towards Heads.
Here, the assumption is “coin is not biased towards heads”. In hypothetical testing, this assumption is known as the null hypothesis (H 0 ).
Result of the experiment
When a coin is tossed once, the probability of getting Heads, i.e. P(H) = 1/2. Similarly, when a coin is tossed 5 times, the probability of getting heads assuming the coin is not biased is 1/2 5 or 1/32.
P(X = 5 | H 0 ) ≈ 0.03 = 3%
This means that there is a probability of 3% of getting 5 heads in 5 flips if the coin is not biassed towards heads.
While performing this experiment, an observation is made which is X = 5.
In hypothesis testing, the probability of observing the value of the test statistic by experimentation i.e. X = 5 if the null hypothesis is true is 3%.
Here, the test statistic i.e. X = 5 is the observation which is the ground truth. Hence, this fact cannot be revoked.
So, given that this observation has already been made, the probability of this observation if the assumption is also true is only 3% which is very low.
This probability value is called the p-value; the result of the statistical hypothesis testing.
Typically, the p-value is said to be small if it is less than or equal to 5%. This is just a rule of thumb.
If the probability of making the observation that we already made, given the null hypothesis is less than or equal to 5%, then probably the assumption (the coin is not biased towards heads) is incorrect. Hence, it can be concluded that the null hypothesis is incorrect. In this scenario, the assumption that the coin is not biased towards heads is rejected, confirming the idea that the coin is biased. In other words, the alternative hypothesis (H 1 ) is failing to reject.
Here the experiment was designed saying the coin was flipped 5 times. What if the coin was flipped 3 times or 10 times? The probability values will change. So, this experiment is dependent on the number of flips. This is often called a sample size.
Now, let the same experiment be modified as flip a coin 3 times and count the number of heads . Here, the new test statistic is 3 i.e. X = 3. Hence, when the coin is tossed 3 times, the probability of getting heads assuming the coin is not biased is 1/2 3 = 1/8.
P(X = 3 | H 0 ) = 0.125 = 12.5%
This means that there is a probability of 12.5% of getting 3 heads in 3 flips if the coin is not biased towards heads.
This probability value is much greater than 5%. Hence, the assumption is failing to reject i.e. the coin is not biased.
The results of the hypothesis testing must be wisely interpreted to make claims about the data. The results can be interpreted in different ways. They are the p-values and critical values.

How to interpret the p-value?
A statistical hypothesis test may return a p-value. A p-value is defined as the probability of making the observation made, given the null hypothesis is true. It is calculated using the sample distribution of the test statistic, under the assumption i.e. null hypothesis.
The p-value is used to quantify the result of the test given the null hypothesis. This is done by comparing the p-value to the threshold value also known as significance level referred to by the Greek letter alpha .
Typically, the alpha value is 0.05 or 5%.
The p-value is compared to the pre-defined alpha value. The result of the experiment is significant when the p-value is less than, equal to the alpha value signifying that a change was detected, rejecting the null hypothesis.
P(test statistic | H 0 ) > alpha (5%): failed to reject H 0
P(test statistic | H 0 ) <= alpha (5%): reject H 0

Let us assume, we performed a statistical hypothesis test of whether the data sample is normally distributed and calculated a p-value of 0.9, we can say that the hypothesis test found that the sample is normally distributed, failing to reject the null hypothesis at a 5% significance level.
There is one mistake that a lot of people often make. Some people think that it is the probability that the null hypothesis (H 0 ) is true.
This is incorrect! We cannot state anything about the null hypothesis (H 0 ).
Instead, the p-value is the probability of the observation you have made given that the null hypothesis (H 0 ) is true.
How to interpret critical values?
Not all statistical tests return a p-value. Instead, they might return a critical value and associated significance level along with the test statistic.
The interpretation of the results is similar to the p-value results. Instead of comparing the p-value to a pre-defined significance level, the test statistic is compared to the critical value at a chosen significance level.
test statistic < critical value: fail to reject H 0
test statistic >= critical value: reject H 0

Representation of the results using the critical values are in the same way as they are interpreted using the p-value.
While performing a statistical hypothesis test of whether the data sample is normally distributed is calculated and the test statistic was compared to the critical value at the 5% significance level, we can say that the hypothesis test found that the sample is normally distributed, failing to reject the null hypothesis at a 5% significance level.
Errors in Hypothesis Testing
In hypothesis testing, relatively small samples are used to answer questions about population parameters. There is always a chance that the selected sample is not representative of the population; therefore, there is always a chance that the conclusion deduced is wrong.
Hypothesis testing provides confidence in favor of a certain hypothesis. In other words, hypothesis testing refers to the use of statistical analysis to determine if observed differences between two or more data samples are due to random chance or to be true differences in the samples.
Since we are computing the probability of an experiment it can be deduced that interpretation of the hypothetical test is purely probabilistic. This means that the outcome of the experiment can be misunderstood.

If the p-value is small; rejecting the null hypothesis indicates either of these 2 scenarios:
- The null hypothesis is false – we are right
- The null hypothesis is true and some rare and unlikely event occurred – we made a mistake.
This type of error is called False Positive – Type I Error.
On contrary, if the p-value is large; failing to reject the null hypothesis indicates either of these 2 scenarios:
- The null hypothesis is true – we are right
- The null hypothesis is false and some rare and unlikely event occurred – we made a mistake.
This type of error is called False Negative – Type II Error.
There is always a possibility of making these kinds of errors while interpreting the results of hypothesis testing. Hence, we must be cautious of encountering such errors and verify the findings before drawing conclusions.
In machine learning, mostly hypothesis testing is used in a test that assumes that the data has a normal distribution and in a test that assumes that 2 or more sample data are drawn from the same population.
Remember these 2 most important things while performing hypothesis testing:
1. Design the Test statistic
Design the experiment ingeniously.
Here in the above experiment, a coin is flipped 5 and 3 times. The sample sizes have to be carefully chosen while designing the experiment.
2. Design the null hypothesis (H 0 ) carefully
It should be designed in such a manner that it makes the probability computation easy and feasible.
Free Courses

Generative AI - A Way of Life
Explore Generative AI for beginners: create text and images, use top AI tools, learn practical skills, and ethics.

Getting Started with Large Language Models
Master Large Language Models (LLMs) with this course, offering clear guidance in NLP and model training made simple.

Building LLM Applications using Prompt Engineering
This free course guides you on building LLM apps, mastering prompt engineering, and developing chatbots with enterprise data.

Improving Real World RAG Systems: Key Challenges & Practical Solutions
Explore practical solutions, advanced retrieval strategies, and agentic RAG systems to improve context, relevance, and accuracy in AI-driven applications.

Microsoft Excel: Formulas & Functions
Master MS Excel for data analysis with key formulas, functions, and LookUp tools in this comprehensive course.
Recommended Articles
An Introduction to Hypothesis Testing
Hypothesis Testing: A Way to Accept or Reject Y...
The Concept Of Hypothesis Testing in Probabilit...
Hypothesis Testing Made Easy for Data Science B...
Your Guide to Master Hypothesis Testing in Stat...
Everything you need to know about Hypothesis Te...
Understanding The Concept Of Hypothesis In Data...
Creating a Simple Z-test Calculator using Strea...
Quick Guide To Perform Hypothesis Testing
Hypothesis Testing in Inferential Statistics
Responses From Readers
Clear Submit reply
Write for us
Write, captivate, and earn accolades and rewards for your work
- Reach a Global Audience
- Get Expert Feedback
- Build Your Brand & Audience
- Cash In on Your Knowledge
- Join a Thriving Community
- Level Up Your Data Science Game
We use cookies essential for this site to function well. Please click to help us improve its usefulness with additional cookies. Learn about our use of cookies in our Privacy Policy & Cookies Policy .
Show details
This site uses cookies to ensure that you get the best experience possible. To learn more about how we use cookies, please refer to our Privacy Policy & Cookies Policy .
Necessary cookies help make a website usable by enabling basic functions like page navigation and access to secure areas of the website. The website cannot function properly without these cookies.
It is needed for personalizing the website.
Expiry: Session
This cookie is used to prevent Cross-site request forgery (often abbreviated as CSRF) attacks of the website
Type: HTTPS
Preserves the login/logout state of users across the whole site.
Preserves users' states across page requests.
Google One-Tap login adds this g_state cookie to set the user status on how they interact with the One-Tap modal.
Expiry: 365 days
Statistic cookies help website owners to understand how visitors interact with websites by collecting and reporting information anonymously.
Used by Microsoft Clarity, to store and track visits across websites.
Expiry: 1 Year
Used by Microsoft Clarity, Persists the Clarity User ID and preferences, unique to that site, on the browser. This ensures that behavior in subsequent visits to the same site will be attributed to the same user ID.
Used by Microsoft Clarity, Connects multiple page views by a user into a single Clarity session recording.
Expiry: 1 Day
Collects user data is specifically adapted to the user or device. The user can also be followed outside of the loaded website, creating a picture of the visitor's behavior.
Expiry: 2 Years
Use to measure the use of the website for internal analytics
Expiry: 1 Years
The cookie is set by embedded Microsoft Clarity scripts. The purpose of this cookie is for heatmap and session recording.
Collected user data is specifically adapted to the user or device. The user can also be followed outside of the loaded website, creating a picture of the visitor's behavior.
Expiry: 2 Months
This cookie is installed by Google Analytics. The cookie is used to store information of how visitors use a website and helps in creating an analytics report of how the website is doing. The data collected includes the number of visitors, the source where they have come from, and the pages visited in an anonymous form.
Expiry: 399 Days
Used by Google Analytics, to store and count pageviews.
Used by Google Analytics to collect data on the number of times a user has visited the website as well as dates for the first and most recent visit.
Used to send data to Google Analytics about the visitor's device and behavior. Tracks the visitor across devices and marketing channels.
Type: PIXEL
cookies ensure that requests within a browsing session are made by the user, and not by other sites.
Expiry: 6 Months
G_ENABLED_IDPS
use the cookie when customers want to make a referral from their gmail contacts; it helps auth the gmail account.
test_cookie
This cookie is set by DoubleClick (which is owned by Google) to determine if the website visitor's browser supports cookies.
this is used to send push notification using webengage.
WebKlipperAuth
used by webenage to track auth of webenagage.
Linkedin sets this cookie to registers statistical data on users' behavior on the website for internal analytics.
Use to maintain an anonymous user session by the server.
Used as part of the LinkedIn Remember Me feature and is set when a user clicks Remember Me on the device to make it easier for him or her to sign in to that device.
AnalyticsSyncHistory
Used to store information about the time a sync with the lms_analytics cookie took place for users in the Designated Countries.
lms_analytics
Used to store information about the time a sync with the AnalyticsSyncHistory cookie took place for users in the Designated Countries.
Cookie used for Sign-in with Linkedin and/or to allow for the Linkedin follow feature.
allow for the Linkedin follow feature.
often used to identify you, including your name, interests, and previous activity.
Tracks the time that the previous page took to load
Used to remember a user's language setting to ensure LinkedIn.com displays in the language selected by the user in their settings
Tracks percent of page viewed
AMCV_14215E3D5995C57C0A495C55%40AdobeOrg
Indicates the start of a session for Adobe Experience Cloud
Provides page name value (URL) for use by Adobe Analytics
Used to retain and fetch time since last visit in Adobe Analytics
Remembers a user's display preference/theme setting
li_theme_set
Remembers which users have updated their display / theme preferences
Preference cookies enable a website to remember information that changes the way the website behaves or looks, like your preferred language or the region that you are in.
Marketing cookies are used to track visitors across websites. The intention is to display ads that are relevant and engaging for the individual user and thereby more valuable for publishers and third party advertisers.
Used by Google Adsense, to store and track conversions.
Expiry: 3 Months
Save certain preferences, for example the number of search results per page or activation of the SafeSearch Filter. Adjusts the ads that appear in Google Search.
These cookies are used for the purpose of targeted advertising.
Expiry: 6 Hours
Expiry: 1 Month
These cookies are used to gather website statistics, and track conversion rates.
Aggregate analysis of website visitors
This cookie is set by Facebook to deliver advertisements when they are on Facebook or a digital platform powered by Facebook advertising after visiting this website.
Expiry: 4 Months
Contains a unique browser and user ID, used for targeted advertising.
Used by LinkedIn to track the use of embedded services.
Used by LinkedIn for tracking the use of embedded services.
Use these cookies to assign a unique ID when users visit a website.
UserMatchHistory
These cookies are set by LinkedIn for advertising purposes, including: tracking visitors so that more relevant ads can be presented, allowing users to use the 'Apply with LinkedIn' or the 'Sign-in with LinkedIn' functions, collecting information about how visitors use the site, etc.
Used to make a probabilistic match of a user's identity outside the Designated Countries
Expiry: 90 Days
Used to collect information for analytics purposes.
Expiry: 1 year
Used to store session ID for a users session to ensure that clicks from adverts on the Bing search engine are verified for reporting purposes and for personalisation
UnclassNameified cookies are cookies that we are in the process of classNameifying, together with the providers of individual cookies.
Cookie declaration last updated on 24/03/2023 by Analytics Vidhya.
Cookies are small text files that can be used by websites to make a user's experience more efficient. The law states that we can store cookies on your device if they are strictly necessary for the operation of this site. For all other types of cookies, we need your permission. This site uses different types of cookies. Some cookies are placed by third-party services that appear on our pages. Learn more about who we are, how you can contact us, and how we process personal data in our Privacy Policy .
Flagship Courses
Popular categories, generative ai tools and techniques, popular genai models, data science tools and techniques, genai pinnacle program, revolutionizing ai learning & development.
- 1:1 Mentorship with Generative AI experts
- Advanced Curriculum with 200+ Hours of Learning
- Master 26+ GenAI Tools and Libraries
Enroll with us today!
Continue your learning for free, enter email address to continue, enter otp sent to.
Resend OTP in 45s
Research Hypothesis In Psychology: Types, & Examples
Saul McLeod, PhD
Editor-in-Chief for Simply Psychology
BSc (Hons) Psychology, MRes, PhD, University of Manchester
Saul McLeod, PhD., is a qualified psychology teacher with over 18 years of experience in further and higher education. He has been published in peer-reviewed journals, including the Journal of Clinical Psychology.
Learn about our Editorial Process
Olivia Guy-Evans, MSc
Associate Editor for Simply Psychology
BSc (Hons) Psychology, MSc Psychology of Education
Olivia Guy-Evans is a writer and associate editor for Simply Psychology. She has previously worked in healthcare and educational sectors.
On This Page:
A research hypothesis, in its plural form “hypotheses,” is a specific, testable prediction about the anticipated results of a study, established at its outset. It is a key component of the scientific method .
Hypotheses connect theory to data and guide the research process towards expanding scientific understanding
Some key points about hypotheses:
- A hypothesis expresses an expected pattern or relationship. It connects the variables under investigation.
- It is stated in clear, precise terms before any data collection or analysis occurs. This makes the hypothesis testable.
- A hypothesis must be falsifiable. It should be possible, even if unlikely in practice, to collect data that disconfirms rather than supports the hypothesis.
- Hypotheses guide research. Scientists design studies to explicitly evaluate hypotheses about how nature works.
- For a hypothesis to be valid, it must be testable against empirical evidence. The evidence can then confirm or disprove the testable predictions.
- Hypotheses are informed by background knowledge and observation, but go beyond what is already known to propose an explanation of how or why something occurs.
Predictions typically arise from a thorough knowledge of the research literature, curiosity about real-world problems or implications, and integrating this to advance theory. They build on existing literature while providing new insight.
Types of Research Hypotheses
Alternative hypothesis.
The research hypothesis is often called the alternative or experimental hypothesis in experimental research.
It typically suggests a potential relationship between two key variables: the independent variable, which the researcher manipulates, and the dependent variable, which is measured based on those changes.
The alternative hypothesis states a relationship exists between the two variables being studied (one variable affects the other).
A hypothesis is a testable statement or prediction about the relationship between two or more variables. It is a key component of the scientific method. Some key points about hypotheses:
- Important hypotheses lead to predictions that can be tested empirically. The evidence can then confirm or disprove the testable predictions.
In summary, a hypothesis is a precise, testable statement of what researchers expect to happen in a study and why. Hypotheses connect theory to data and guide the research process towards expanding scientific understanding.
An experimental hypothesis predicts what change(s) will occur in the dependent variable when the independent variable is manipulated.
It states that the results are not due to chance and are significant in supporting the theory being investigated.
The alternative hypothesis can be directional, indicating a specific direction of the effect, or non-directional, suggesting a difference without specifying its nature. It’s what researchers aim to support or demonstrate through their study.
Null Hypothesis
The null hypothesis states no relationship exists between the two variables being studied (one variable does not affect the other). There will be no changes in the dependent variable due to manipulating the independent variable.
It states results are due to chance and are not significant in supporting the idea being investigated.
The null hypothesis, positing no effect or relationship, is a foundational contrast to the research hypothesis in scientific inquiry. It establishes a baseline for statistical testing, promoting objectivity by initiating research from a neutral stance.
Many statistical methods are tailored to test the null hypothesis, determining the likelihood of observed results if no true effect exists.
This dual-hypothesis approach provides clarity, ensuring that research intentions are explicit, and fosters consistency across scientific studies, enhancing the standardization and interpretability of research outcomes.
Nondirectional Hypothesis
A non-directional hypothesis, also known as a two-tailed hypothesis, predicts that there is a difference or relationship between two variables but does not specify the direction of this relationship.
It merely indicates that a change or effect will occur without predicting which group will have higher or lower values.
For example, “There is a difference in performance between Group A and Group B” is a non-directional hypothesis.
Directional Hypothesis
A directional (one-tailed) hypothesis predicts the nature of the effect of the independent variable on the dependent variable. It predicts in which direction the change will take place. (i.e., greater, smaller, less, more)
It specifies whether one variable is greater, lesser, or different from another, rather than just indicating that there’s a difference without specifying its nature.
For example, “Exercise increases weight loss” is a directional hypothesis.

Falsifiability
The Falsification Principle, proposed by Karl Popper , is a way of demarcating science from non-science. It suggests that for a theory or hypothesis to be considered scientific, it must be testable and irrefutable.
Falsifiability emphasizes that scientific claims shouldn’t just be confirmable but should also have the potential to be proven wrong.
It means that there should exist some potential evidence or experiment that could prove the proposition false.
However many confirming instances exist for a theory, it only takes one counter observation to falsify it. For example, the hypothesis that “all swans are white,” can be falsified by observing a black swan.
For Popper, science should attempt to disprove a theory rather than attempt to continually provide evidence to support a research hypothesis.
Can a Hypothesis be Proven?
Hypotheses make probabilistic predictions. They state the expected outcome if a particular relationship exists. However, a study result supporting a hypothesis does not definitively prove it is true.
All studies have limitations. There may be unknown confounding factors or issues that limit the certainty of conclusions. Additional studies may yield different results.
In science, hypotheses can realistically only be supported with some degree of confidence, not proven. The process of science is to incrementally accumulate evidence for and against hypothesized relationships in an ongoing pursuit of better models and explanations that best fit the empirical data. But hypotheses remain open to revision and rejection if that is where the evidence leads.
- Disproving a hypothesis is definitive. Solid disconfirmatory evidence will falsify a hypothesis and require altering or discarding it based on the evidence.
- However, confirming evidence is always open to revision. Other explanations may account for the same results, and additional or contradictory evidence may emerge over time.
We can never 100% prove the alternative hypothesis. Instead, we see if we can disprove, or reject the null hypothesis.
If we reject the null hypothesis, this doesn’t mean that our alternative hypothesis is correct but does support the alternative/experimental hypothesis.
Upon analysis of the results, an alternative hypothesis can be rejected or supported, but it can never be proven to be correct. We must avoid any reference to results proving a theory as this implies 100% certainty, and there is always a chance that evidence may exist which could refute a theory.
How to Write a Hypothesis
- Identify variables . The researcher manipulates the independent variable and the dependent variable is the measured outcome.
- Operationalized the variables being investigated . Operationalization of a hypothesis refers to the process of making the variables physically measurable or testable, e.g. if you are about to study aggression, you might count the number of punches given by participants.
- Decide on a direction for your prediction . If there is evidence in the literature to support a specific effect of the independent variable on the dependent variable, write a directional (one-tailed) hypothesis. If there are limited or ambiguous findings in the literature regarding the effect of the independent variable on the dependent variable, write a non-directional (two-tailed) hypothesis.
- Make it Testable : Ensure your hypothesis can be tested through experimentation or observation. It should be possible to prove it false (principle of falsifiability).
- Clear & concise language . A strong hypothesis is concise (typically one to two sentences long), and formulated using clear and straightforward language, ensuring it’s easily understood and testable.
Consider a hypothesis many teachers might subscribe to: students work better on Monday morning than on Friday afternoon (IV=Day, DV= Standard of work).
Now, if we decide to study this by giving the same group of students a lesson on a Monday morning and a Friday afternoon and then measuring their immediate recall of the material covered in each session, we would end up with the following:
- The alternative hypothesis states that students will recall significantly more information on a Monday morning than on a Friday afternoon.
- The null hypothesis states that there will be no significant difference in the amount recalled on a Monday morning compared to a Friday afternoon. Any difference will be due to chance or confounding factors.
More Examples
- Memory : Participants exposed to classical music during study sessions will recall more items from a list than those who studied in silence.
- Social Psychology : Individuals who frequently engage in social media use will report higher levels of perceived social isolation compared to those who use it infrequently.
- Developmental Psychology : Children who engage in regular imaginative play have better problem-solving skills than those who don’t.
- Clinical Psychology : Cognitive-behavioral therapy will be more effective in reducing symptoms of anxiety over a 6-month period compared to traditional talk therapy.
- Cognitive Psychology : Individuals who multitask between various electronic devices will have shorter attention spans on focused tasks than those who single-task.
- Health Psychology : Patients who practice mindfulness meditation will experience lower levels of chronic pain compared to those who don’t meditate.
- Organizational Psychology : Employees in open-plan offices will report higher levels of stress than those in private offices.
- Behavioral Psychology : Rats rewarded with food after pressing a lever will press it more frequently than rats who receive no reward.
- Search Search Please fill out this field.
What Is a Null Hypothesis?
The alternative hypothesis.
- Additional Examples
- Null Hypothesis and Investments
The Bottom Line
- Corporate Finance
- Financial Ratios
Null Hypothesis: What Is It, and How Is It Used in Investing?
Adam Hayes, Ph.D., CFA, is a financial writer with 15+ years Wall Street experience as a derivatives trader. Besides his extensive derivative trading expertise, Adam is an expert in economics and behavioral finance. Adam received his master's in economics from The New School for Social Research and his Ph.D. from the University of Wisconsin-Madison in sociology. He is a CFA charterholder as well as holding FINRA Series 7, 55 & 63 licenses. He currently researches and teaches economic sociology and the social studies of finance at the Hebrew University in Jerusalem.
:max_bytes(150000):strip_icc():format(webp)/adam_hayes-5bfc262a46e0fb005118b414.jpg)
Yarilet Perez is an experienced multimedia journalist and fact-checker with a Master of Science in Journalism. She has worked in multiple cities covering breaking news, politics, education, and more. Her expertise is in personal finance and investing, and real estate.
:max_bytes(150000):strip_icc():format(webp)/YariletPerez-d2289cb01c3c4f2aabf79ce6057e5078.jpg)
A null hypothesis is a type of statistical hypothesis that proposes that no statistical significance exists in a set of given observations. Hypothesis testing is used to assess the credibility of a hypothesis by using sample data. Sometimes referred to simply as the “null,” it is represented as H 0 .
The null hypothesis, also known as “the conjecture,” is used in quantitative analysis to test theories about markets, investing strategies, and economies to decide if an idea is true or false.
Key Takeaways
- A null hypothesis is a type of conjecture in statistics that proposes that there is no difference between certain characteristics of a population or data-generating process.
- The alternative hypothesis proposes that there is a difference.
- Hypothesis testing provides a method to reject a null hypothesis within a certain confidence level.
- If you can reject the null hypothesis, it provides support for the alternative hypothesis.
- Null hypothesis testing is the basis of the principle of falsification in science.
Alex Dos Diaz / Investopedia
Understanding a Null Hypothesis
A gambler may be interested in whether a game of chance is fair. If it is, then the expected earnings per play come to zero for both players. If it is not, then the expected earnings are positive for one player and negative for the other.
To test whether the game is fair, the gambler collects earnings data from many repetitions of the game, calculates the average earnings from these data, then tests the null hypothesis that the expected earnings are not different from zero.
If the average earnings from the sample data are sufficiently far from zero, then the gambler will reject the null hypothesis and conclude the alternative hypothesis—namely, that the expected earnings per play are different from zero. If the average earnings from the sample data are near zero, then the gambler will not reject the null hypothesis, concluding instead that the difference between the average from the data and zero is explainable by chance alone.
A null hypothesis can only be rejected, not proven.
The null hypothesis assumes that any kind of difference between the chosen characteristics that you see in a set of data is due to chance. For example, if the expected earnings for the gambling game are truly equal to zero, then any difference between the average earnings in the data and zero is due to chance.
Analysts look to reject the null hypothesis because doing so is a strong conclusion. This requires evidence in the form of an observed difference that is too large to be explained solely by chance. Failing to reject the null hypothesis—that the results are explainable by chance alone—is a weak conclusion because it allows that while factors other than chance may be at work, they may not be strong enough for the statistical test to detect them.
An important point to note is that we are testing the null hypothesis because there is an element of doubt about its validity. Whatever information that is against the stated null hypothesis is captured in the alternative (alternate) hypothesis (H 1 ).
For the examples below, the alternative hypothesis would be:
- Students score an average that is not equal to seven.
- The mean annual return of a mutual fund is not equal to 8% per year.
In other words, the alternative hypothesis is a direct contradiction of the null hypothesis.
Null Hypothesis Examples
Here is a simple example: A school principal claims that students in their school score an average of seven out of 10 in exams. The null hypothesis is that the population mean is not 7.0. To test this null hypothesis, we record marks of, say, 30 students ( sample ) from the entire student population of the school (say, 300) and calculate the mean of that sample.
We can then compare the (calculated) sample mean to the (hypothesized) population mean of 7.0 and attempt to reject the null hypothesis. (The null hypothesis here—that the population mean is not 7.0—cannot be proved using the sample data. It can only be rejected.)
Take another example: The annual return of a particular mutual fund is claimed to be 8%. Assume that the mutual fund has been in existence for 20 years. The null hypothesis is that the mean return is not 8% for the mutual fund. We take a random sample of annual returns of the mutual fund for, say, five years (sample) and calculate the sample mean. We then compare the (calculated) sample mean to the (claimed) population mean (8%) to test the null hypothesis.
For the above examples, null hypotheses are:
- Example A: Students in the school don’t score an average of seven out of 10 in exams.
- Example B: The mean annual return of the mutual fund is not 8% per year.
For the purposes of determining whether to reject the null hypothesis (abbreviated H0), said hypothesis is assumed, for the sake of argument, to be true. Then the likely range of possible values of the calculated statistic (e.g., the average score on 30 students’ tests) is determined under this presumption (e.g., the range of plausible averages might range from 6.2 to 7.8 if the population mean is 7.0).
If the sample average is outside of this range, the null hypothesis is rejected. Otherwise, the difference is said to be “explainable by chance alone,” being within the range that is determined by chance alone.
Traditional null hypothesis testing, consisting of a comparative statistical test for two competing theories, was suggested by Ronald Fisher in 1925.
How Null Hypothesis Testing Is Used in Investments
As an example related to financial markets, assume Alice sees that her investment strategy produces higher average returns than simply buying and holding a stock . The null hypothesis states that there is no difference between the two average returns, and Alice is inclined to believe this until she can conclude contradictory results.
Refuting the null hypothesis would require showing statistical significance, which can be found by a variety of tests. The alternative hypothesis would state that the investment strategy has a higher average return than a traditional buy-and-hold strategy.
One tool that can determine the statistical significance of the results is the p-value. A p-value represents the probability that a difference as large or larger than the observed difference between the two average returns could occur solely by chance.
A p-value that is less than or equal to 0.05 often indicates whether there is evidence against the null hypothesis. If Alice conducts one of these tests, such as a test using the normal model, resulting in a significant difference between her returns and the buy-and-hold returns (the p-value is less than or equal to 0.05), she can then reject the null hypothesis and conclude the alternative hypothesis.
How Is the Null Hypothesis Identified?
The analyst or researcher establishes a null hypothesis based on the research question or problem they are trying to answer. Depending on the question, the null may be identified differently. For example, if the question is simply whether an effect exists (e.g., does X influence Y?), the null hypothesis could be H 0 : X = 0. If the question is instead, is X the same as Y, the H 0 would be X = Y. If it is that the effect of X on Y is positive, H 0 would be X > 0. If the resulting analysis shows an effect that is statistically significantly different from zero, the null can be rejected.
How Is Null Hypothesis Used in Finance?
In finance , a null hypothesis is used in quantitative analysis. It tests the premise of an investing strategy, the markets, or an economy to determine if it is true or false.
For instance, an analyst may want to see if two stocks, ABC and XYZ, are closely correlated. The null hypothesis would be ABC ≠ XYZ.
How Are Statistical Hypotheses Tested?
Statistical hypotheses are tested in a four-step process . The first is for the analyst to state the two hypotheses so that only one can be right. The second is to formulate an analysis plan, which outlines how the data will be evaluated. The third is to carry out the plan and physically analyze the sample data. The fourth and final step is to analyze the results and either reject the null hypothesis or claim that the observed differences are explainable by chance alone.
What Is an Alternative Hypothesis?
An alternative hypothesis is a direct contradiction of a null hypothesis. This means that if one of the two hypotheses is true, the other is false.
A null hypothesis states there is no difference between groups or relationship between variables. It is a type of statistical hypothesis and proposes that no statistical significance exists in a set of given observations. “Null” means nothing.
The null hypothesis is used in quantitative analysis to test theories about economies, investing strategies, and markets to decide if an idea is true or false. Hypothesis testing assesses the credibility of a hypothesis by using sample data. It is represented as H 0 and is sometimes simply known as “the null.”
Correction—July 23, 2024: This article was corrected to state accurate examples of null hypothesis in the Null Hypothesis Examples section.
National Library of Medicine. " Current Controversies: Null Hypotheses in Statistical Testing ."
:max_bytes(150000):strip_icc():format(webp)/LoanCostRatio_final-868418a3086541ebaee40f1bc15a9c91.png)
- Terms of Service
- Editorial Policy
- Privacy Policy
Talk to our experts
1800-120-456-456
- Null Hypothesis

Before understanding the Null Hypothesis in detail, let us first understand the definition of the Hypothesis.
In Statistics, a hypothesis can be defined as a formal statement, which gives an explanation about the relationship between any two or more variables of the specified population.
Hypothesis helps the researcher to translate any given problem to a clear explanation for the outcome of the study.
Hypothesis clearly explains and predicts the expected outcome and it indicates the types of experimental design and directs the study of any research process.
What Is The Null Hypothesis?
We can define a null hypothesis as a general statement or a default position that says there is no relationship between two measured phenomena or there is no association among groups.
Why is Null Hypothesis Important?
Testing (which involves accepting, approving, rejecting, or disproving) the null hypothesis and thus concluding that there are or we can say that there are no grounds for believing that there is any relationship between two phenomena is basically a central task in the modern practice of science; in the field of statistics.
To be more specific, hypothesis testing gives precise criteria for rejecting or accepting a null hypothesis within a level known as the confidence level.
Null Hypothesis Symbol-
A Null Hypothesis is denoted by the symbol H 0 in statistics. It is usually pronounced as “h-nought” or “H-null”. The Subscript in H is the digit 0.
Null Hypothesis Principle and When is A Null Hypothesis Rejected?
The principle followed for null hypothesis testing is basically collecting the data and determining the chances of a given set of data during the study on any given random sample, assuming that the null hypothesis is true.
Suppose, if the given data does not face the expected null hypothesis, then the outcome we will get will be quite weaker and they conclude that by saying that the given set of data does not provide strong evidence against the null hypothesis which is because of insufficient evidence.
Finally, this leads to null hypothesis rejection.
Null Hypothesis Formula or How Do You Find The Null Hypothesis :
So far, understanding the concept of null hypothesis, let’s now discuss the null hypothesis formula:
Here, the null hypothesis formula is given below.
H 0 : p = p 0
The formula for the alternative hypothesis can be written as: H a = p >p 0 , and < p 0 ≠ p 0
The formula for the test static is denoted by:
\[Z=\frac{P-P_{0}}{\sqrt{\frac{P-P_{0}}{n}}}\]
Remember that, p 0 here is the null hypothesis.
Types of Null Hypothesis.
There are 4 different types of Null hypothesis. Each of them is explained below with examples.
Simple Hypothesis: A simple Hypothesis is the one in which the relationship between two variables is predicted. One is an independent variable and the other is a dependent variable. A simple Hypothesis completely specifies the population distribution. An example of a simple Hypothesis is “Consuming sugar drinks daily leads to being overweight.”
Composite hypothesis. A Composite hypothesis describes the relationship between two or more independent variables and two or more dependent variables. In this hypothesis, the population distribution is not specified. An example of the Composite hypothesis is stated below. “ Individuals who consume sugar drinks daily and have a family history of health issues are more likely to become overweight and develop diabetes.”
Exact hypothesis. In this hypothesis, the exact value of the parameter or variable is defined. All the assumptions made during the derivation of the hypothesis are met in the exact hypothesis. An example of an exact hypothesis is, “ Students in a division score an average 17 out of 25 in exams. Hence, μ=17.”
Inexact hypothesis. Unlike the exact hypothesis, the exact value of the parameter or variable is not defined in the Inexact type of Hypothesis. Instead, a specific range or interval of the parameter is stated. For example, Students in class score an average between 12 to 15 out of 20 in exams. Hence, 12< μ< 15.
Null Hypothesis Problem Problem : A researcher thinks that if the knee surgery patients go to physical therapy twice a week (instead of going 3 times), their recovery period will be longer. Given average recovery time knee surgery patients are given 8.2 weeks.
Step 1: First, we need to figure out the hypothesis from the problem . The hypothesis is usually hidden in a word problem that you need to figure out. The hypothesis that has been given in the above question is “I expect the average recovery period to be greater than 8.2 weeks.”
Step 2: You need to convert the hypothesis to math . Remember that the average can be sometimes written as μ.
H 1 : μ > 8.2(average)
Step 3: Now state what will happen if the hypothesis doesn’t come true. If the recovery time is not greater than the given average that is 8.2 weeks, there are only two possibilities, that the recovery time is equal to 8.2 weeks or it is less than 8.2 weeks.
H 0 : μ ≤ 8.2
H 0 (The null hypothesis): μ (the average) ≤ (is less than or equal to) 8.2
H 0 🡪 μ 1 = μ 2 where
H 0 is the null hypothesis,
μ 1 is the mean of population 1, and
μ 2 is the mean of population 2.
A stronger null hypothesis denotes that if two samples are drawn from the same given population, such that the variances and shapes of the given distributions are also equal.
Examples of situations where hypothesis tests are used in the real world.
Is there a 100% chance of getting affected by dengue?
Answer : There could be chances of getting affected by dengue but the chances of getting affected by dengue are not 100%.
Do teenagers these days use mobile phones more than adults to access the internet?
Answer : Age has no limit and is not a factor that we can use mobile phones to access the internet.
Does having the fruit apple daily will not cause fever?
Answer : Having an apple daily does not assure of not having fever, but on the other hand it does increase the immunity to fight against such diseases.
Are children better at performing mathematical calculations than adults?
Answer : Again in this example too, age has no effect on Mathematical skills.
Hypothesis tests are often conducted in botany to determine whether some new treatment, fertilizer or chemical can cause increased growth, stamina or immunity in plants and animals.
Suppose, a botanist believes that a certain fertilizer will cause plants to grow more during a 1 month period than what they actually grow. Currently, they grow to 20 inches. To test the effectiveness of the fertilizer, the botanist applies the fertilizer to each of the plants in her lab for a month. Then performs a hypothesis test using the following hypothesis: H 0 : μ = 20 inches as the effect is nil on the mean plant growth by the fertilizer.
H A : μ > 20 inches (the fertilizer will cause mean plant growth to increase)
If the p-value of the test is significantly less then it will reject the null hypothesis and as a result, the fertilizer leads to increased plant growth.
In clinical trials, hypothesis tests are often used to determine whether some new treatment, drug, procedure, etc. cause improved outcomes in patients and thus elevate the treatment levels. Suppose a doctor wishes to test a new drug that reduces blood pressure in obese patients. To test its effectiveness, the doctor may measure the blood pressure of 40 patients before and after using the new drug for a month. Then a hypothesis test is performed using the following hypothesis. H 0 : μafter = μbefore means the mean blood pressure is the same before and after using the drug
H A : μafter < μbefore (the mean blood pressure is less after using the drug)
Hypothesis tests are often conducted in business organizations to determine whether a new advertising campaign will get more leads and cause an increase in sales. Suppose, the executive team of an organization believes that spending more on digital advertising leads to increased sales. To test this, the organization may increase money spent on digital advertising during a 3 month period and collect data to see if overall sales have increased or not. A hypothesis test can be performed using the following hypothesis.
H 0 : μafter = μbefore signifies the mean sales is the same before and after spending more on advertising
H A : μafter > μbefore (the mean sales increased spending more on advertising)
Apart from just the above 3 examples, hypothesis testing is widely used in research centers, chemical laboratories, stock market predictions, the Investment industry and several business organizations.
Decision errors.
There are two types of decision errors that can happen when doing a hypothesis test. They are described below.
Type I error: This happens when one rejects the null hypothesis despite the null hypothesis being true. The probability of making a type I error is equal to the alpha level significantly
Type II error. This happens when one fails to reject the null hypothesis when it is actually false. The probability of committing a type II error is the power of the test.
Importance of Hypothesis testing in real life and examples
The main aim of a hypothesis statement in statistics is to determine whether or not some hypothesis of a population parameter is true or false. It is an essential part of the procedures in statistics.
A hypothesis test evaluates two mutually exclusive statements and determines which statement is best supported by sample data. A discovery or a new finding holds a significant value when supported by a hypothesis test. Because the hypothesis test provides sufficient evidence to check the credibility of the given data.
To conduct a hypothesis test in the practical world, researchers obtain a random sample from the population and perform a hypothesis test on the sample data using Null and Alternative hypotheses.
Difference between Null Hypothesis and Alternative Hypothesis.
Parameter | Null Hypothesis | Alternative Hypothesis |
Observation | Concludes that the results are observed as a result of chance. | Concludes that the results are observed due to some real causes. |
Symbol | Denoted by H0 | Denoted by HA |
Definition | States that two factors or groups are unrelated and there is no difference between certain characteristics of a population. | States that there is a relation between the two variables or groups under consideration. |
Nature | Researchers usually try to disprove the null hypothesis. | Researchers try to prove an alternative hypothesis. |
Acceptance | If the obtained p-value is greater than the level of significance, then the null hypothesis is accepted. | If the obtained p-value is smaller than the level of significance, then an alternative hypothesis is accepted. |
FAQs on Null Hypothesis
1. Why is a null hypothesis important and can you accept a null hypothesis?
The purpose and the importance of the null hypothesis and alternative hypothesis are that they provide an approximate description of any given phenomena. The purpose of the null hypothesis is to provide the researcher or an investigator with the relational statement that is directly tested in any research study.
A null hypothesis is a hypothesis that is never acceptable. We either reject them or we fail to reject them. Hence, failing to reject the null hypothesis does not mean that we have shown that there is no difference in accepting the null hypothesis.
2. State one example where a Null hypothesis is applied in a practical life scenario?
The concept of null hypothesis has a variety of practical applications. Let us take the example of stock investment, buying shares of a company. The annual return of company stock when invested for a long duration is assumed to be 7.5%.
Now to test if the assumption is true or false, we consider the null hypothesis to be, ”the mean annual return for XYZ company shares is not 7.5%”. To test this hypothesis, we first accept the null hypothesis.
We then check the history of the stock performance of the last 5 years of XYZ company and then calculate the mean of the annual return. The result is then compared to the assumed annual return of 7.5%.
If it turns out that the average annual return of the last 5 years is 7.5%, then the null hypothesis is rejected. And the alternative hypothesis is accepted.
3. What is the purpose of Hypothesis testing?
Hypothesis testing is a statistical process of testing an assumption regarding a particular phenomenon or parameter. Often a good theory can make accurate predictions. However, for an analyst, hypothesis testing is a rigorous way of backing up his prediction with statistical analysis to support the predictions. It is also helpful to determine sufficient statistical evidence that can favor a certain hypothesis about the specified parameter.
In simple words, hypothesis testing is a systematic approach to assessing theories through observations and then determining whether the stated statement is true or false.

- Trending Now
- Foundational Courses
- Data Science
- Practice Problem
- Machine Learning
- System Design
- DevOps Tutorial
Difference between Null and Alternate Hypothesis
Hypothesis is a statement or an assumption that may be true or false. There are six types of hypotheses mainly the Simple hypothesis, Complex hypothesis, Directional hypothesis, Associative hypothesis, and Null hypothesis. Usually, the hypothesis is the start point of any scientific investigation, It gives the right direction to the process of investigation. It avoids the blind search and gives direction to the search. It acts as a compass in the process.

Null hypothesis suggests that there is no relationship between the two variables. Null hypothesis is also exactly the opposite of the alternative hypothesis. Null hypothesis is generally what researchers or scientists try to disprove and if the null hypothesis gets accepted then we have to make changes in our opinion i.e. we have to make changes in our original opinion or statement in order to match null hypothesis. Null hypothesis is represented as H0. If my alternative hypothesis is that 55% of boys in my town are taller than girls then my alternative hypothesis will be that 55% of boys in my town are not taller than girls.
Alternative hypothesis is a method for reaching a conclusion and making inferences and judgements about certain facts or a statement. This is done on the basis of the data which is available. Usually, the statement which we check regarding the null hypothesis is commonly known as the alternative hypothesis. Most of the times alternative hypothesis is exactly the opposite of the null hypothesis. This is what generally researchers or scientists try to approve. Alternative hypothesis is represented as Ha or H1. If my null hypothesis is that 55% of boys in my town are not taller than girls then my alternative hypothesis will be that 55% of boys in my town are taller than girls.
Following is the difference between the null hypothesis and alternate hypothesis:
|
|
| |
| 1. | In the null hypothesis, there is no relationship between the two variables. | In the alternative hypothesis, there is some relationship between the two variables i.e. They are dependent upon each other. |
| 2. | Generally, researchers and scientists try to reject or disprove the null hypothesis. | Generally, researchers and scientists try to accept or approve the null hypothesis |
| 3. | If the null hypothesis is accepted researchers have to make changes in their opinions and statements. | If the alternative hypothesis gets accepted researchers do not have to make changes in their opinions and statements. |
| 4. | Here no effect can be observed i.e. it does not affect output. | Here effect can be observed i.e. it affects the output. |
| 5. | Here the testing process is implicit and indirect. | Here the testing process is explicit and direct. |
| 6. | This hypothesis is denoted by H0. | This hypothesis is denoted by Ha or H1. |
| 7. | It is generally used when we reject the null hypothesis. | It gets accepted if we fail to reject the null hypothesis. |
| 8. | In this hypothesis, the p-value is smaller than the significance level. | In this hypothesis, the p-value is greater than the significance level. |

Similar Reads
- Difference Between
Please Login to comment...
- Free Music Recording Software in 2024: Top Picks for Windows, Mac, and Linux
- Best Free Music Maker Software in 2024
- What is Quantum AI? The Future of Computing and Artificial Intelligence Explained
- Noel Tata: Ratan Tata's Brother Named as a new Chairman of Tata Trusts
- GeeksforGeeks Practice - Leading Online Coding Platform
Improve your Coding Skills with Practice
What kind of Experience do you want to share?
Stack Exchange Network
Stack Exchange network consists of 183 Q&A communities including Stack Overflow , the largest, most trusted online community for developers to learn, share their knowledge, and build their careers.
Q&A for work
Connect and share knowledge within a single location that is structured and easy to search.
Null hypothesis for linear regression
I am confused about the null hypothesis for linear regression.
If a variable in a linear model has $p < 0.05$ (when R prints out stars), I would say the variable is a statistically significant part of the model.
What does that translate to in terms of null hypothesis?
Am I rejecting the null hypothesis that the coefficient for that variable is $0,$ or am I accepting a null hypothesis that the coefficient is $\ne 0$ ?
Is there a difference between those statements?
- hypothesis-testing
- interpretation
3 Answers 3
The issue applies to null hypotheses more broadly than regression
You should get used to stating nulls before you look at p-values.
Am I rejecting the null hypothesis that the coefficient for that variable is 0
Yes, as long as it's the population coefficient, ($\beta_i$) you're talking about (obviously - with continuous response - the estimate of the coefficient isn't 0).
or am I accepting a null hypothesis that the coefficient is != 0?
Null hypotheses would generally be null - either 'no effect' or some conventionally accepted value. In this case, the population coefficient being 0 is a classical 'no effect' null.
More prosaically, when testing a point hypothesis against a composite alternative (a two-sided alternative in this case), one takes the point hypothesis as the null, because that's the one under which we can compute the distribution of the test statistic (more generally, using an open set for a null presents certain problems, even when both are composite). With a pair of point hypotheses, one is (at least mechanically) free to make either one the null (and even then one still would generally want to make the one that's most clearly "null" the null -- if either of them is; that is to choose the 'no effect' or conventionally-accepted one the null).
- $\begingroup$ Thanks. Now I finally understand why it's called the 'null hypothesis.' Related question: is there a way to accept the null hypothesis - to say you have enough evidence to state there is no relationship between the vars? $\endgroup$ – wrschneider Commented Jan 30, 2015 at 15:37
- $\begingroup$ Well, that could depend on who you ask and partly on the circumstances. At least with a point null vs a composite alternative (the most common situation), I say the answer is no, since failure to reject doesn't indicate the null is actually true, only that the effect is probably quite small (relative to what the sample size could detect). In some of the other cases, more of an argument might be made, but I'd still say 'no'. Of course, ordinary null-hypothesis significance testing isn't the only possible choice, and some of the other choices would give more of a basis to do that. $\endgroup$ – Glen_b Commented Jan 30, 2015 at 15:56
- 1 $\begingroup$ @wrschneider99 Obligatory plug for tests for equivalence as an alternative way of thinking about "accepting the null hypothesis." $\endgroup$ – Alexis Commented Feb 13, 2015 at 3:53
- $\begingroup$ @Alexis +1 definitely worth raising. $\endgroup$ – Glen_b Commented Feb 13, 2015 at 4:24
- $\begingroup$ @Glen_b: I know this is a broad question, but if you could give me a quick answer or ref: How does one go about choosing the right statistic, or any statistic to test a hypothesis? In this case we test wether the slope is 0. How do we choose/find a statistic that would allow us to test this claim? How do we come up with, e.g., the F-test when determining equality of variance? How is the process in general? Appreciate a ref. if question is too broad. $\endgroup$ – MSIS Commented Oct 30, 2020 at 12:53
The P-Value in regression output in R tests the null hypothesis that the coefficient equals 0.
Any regression equation is given by y = a + b*x + u, where 'a' and 'b' are the intercept and slope of the best fit line and 'u' is the disturbance term. Imagine b=0; the equation would then be y = a + 0*x + u = a + u. Notice that the 'x' has disappeared. It simply means that there is no relationship between y and x. Thus are test hypothesis would be, Ho: b=0; Ha: b != 0
Next step is to compare the critical values with the test statistic. If the test stat lies within the Rejection region then we knockout the Null hypothesis.
Alternatively, the p-value also relays this information by pointing out how much probability remains between the test stat and the end tails.
Your Answer
Sign up or log in, post as a guest.
Required, but never shown
By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy .
Not the answer you're looking for? Browse other questions tagged regression hypothesis-testing interpretation or ask your own question .
- Featured on Meta
- Upcoming initiatives on Stack Overflow and across the Stack Exchange network...
- Preventing unauthorized automated access to the network
Hot Network Questions
- What would happen if a natural disaster occurred on election Day?
- Under what circumstances is the observation of X proof of the existence of X?
- The shortcut hint
- How Can I Ethically Adjust Grading Without Violating My 'No Grade Curving' Policy?
- Card design with long and short text options
- Why does Windows 11 display a different ethernet MAC (hardware) address than Linux?
- What was the first game to implement ADS?
- Windows SMB and NIC RSS
- Would Acid Fog dissolve the opponent's equipment?
- Could compressed air be used in a 'whipper'
- How do I draw a single object to print from multiple materials?
- Does a vector change under coordinate transformation?
- What traits can I combine to make a long-lived race that matures at the human norm?
- "Riiiight," he said. What synonym of said can be used here?
- Could there be a legitimate reason for a SSH server to allow null authentication, to anyone?
- Asymptotic behavior of partial sums of Dirichlet series
- Why pick up the arba minim prior to making the bracha?
- Capacitance multiplier as a reservoir capacitor
- Can Congress pass a law that sets an incarcerated person free?
- Roll a die in 3D
- Rename files with non-Latin characters
- How to distinguish contrast from simultaneity when using the connective "while"?
- What expressions (verbs) are used for the actions of adding ingredients (solid, fluid, powdery) into a container, specifically while cooking?
- What Are the Computational Complexity Bounds for Dynamic Programming in Reinforcement Learning?
- More from M-W
- To save this word, you'll need to log in. Log In
null hypothesis
Definition of null hypothesis
Examples of null hypothesis in a sentence.
These examples are programmatically compiled from various online sources to illustrate current usage of the word 'null hypothesis.' Any opinions expressed in the examples do not represent those of Merriam-Webster or its editors. Send us feedback about these examples.
Word History
1935, in the meaning defined above
Dictionary Entries Near null hypothesis
Nullarbor Plain
Cite this Entry
“Null hypothesis.” Merriam-Webster.com Dictionary , Merriam-Webster, https://www.merriam-webster.com/dictionary/null%20hypothesis. Accessed 16 Oct. 2024.
More from Merriam-Webster on null hypothesis
Britannica.com: Encyclopedia article about null hypothesis
Subscribe to America's largest dictionary and get thousands more definitions and advanced search—ad free!

Can you solve 4 words at once?
Word of the day.
See Definitions and Examples »
Get Word of the Day daily email!
Popular in Grammar & Usage
Plural and possessive names: a guide, every letter is silent, sometimes: a-z list of examples, the difference between 'i.e.' and 'e.g.', what's the difference between 'fascism' and 'socialism', more commonly misspelled words, popular in wordplay, weird words for autumn time, 8 words with fascinating histories, 8 words for lesser-known musical instruments, birds say the darndest things, 10 words from taylor swift songs (merriam's version), games & quizzes.


IMAGES
VIDEO
COMMENTS
H 0 (Null Hypothesis): Population parameter =, ≤, ≥ some value. H A (Alternative Hypothesis): Population parameter <, >, ≠ some value. Note that the null hypothesis always contains the equal sign. We interpret the hypotheses as follows: Null hypothesis: The sample data provides no evidence to support some claim being made by an individual.
The null hypothesis (H0) answers "No, there's no effect in the population.". The alternative hypothesis (Ha) answers "Yes, there is an effect in the population.". The null and alternative are always claims about the population. That's because the goal of hypothesis testing is to make inferences about a population based on a sample.
To distinguish it from other hypotheses, the null hypothesis is written as H 0 (which is read as "H-nought," "H-null," or "H-zero"). A significance test is used to determine the likelihood that the results supporting the null hypothesis are not due to chance. A confidence level of 95% or 99% is common. Keep in mind, even if the confidence level is high, there is still a small chance the ...
When your sample contains sufficient evidence, you can reject the null and conclude that the effect is statistically significant. Statisticians often denote the null hypothesis as H 0 or H A.. Null Hypothesis H 0: No effect exists in the population.; Alternative Hypothesis H A: The effect exists in the population.; In every study or experiment, researchers assess an effect or relationship.
Null Hypothesis Examples. "Hyperactivity is unrelated to eating sugar " is an example of a null hypothesis. If the hypothesis is tested and found to be false, using statistics, then a connection between hyperactivity and sugar ingestion may be indicated. A significance test is the most common statistical test used to establish confidence in a ...
The null hypothesis is a hypothesis in which the sample observation results from chance. Learn the definition, principles, and types of the null hypothesis at BYJU'S. ... Simple Hypothesis. It completely specifies the population distribution. In this method, the sampling distribution is the function of the sample size.
Step 1: Figure out the hypothesis from the problem. The hypothesis is usually hidden in a word problem, and is sometimes a statement of what you expect to happen in the experiment. The hypothesis in the above question is "I expect the average recovery period to be greater than 8.2 weeks.". Step 2: Convert the hypothesis to math.
A null hypothesis is a statistical concept suggesting no significant difference or relationship between measured variables. It's the default assumption unless empirical evidence proves otherwise. The null hypothesis states no relationship exists between the two variables being studied (i.e., one variable does not affect the other).
The actual test begins by considering two hypotheses.They are called the null hypothesis and the alternative hypothesis.These hypotheses contain opposing viewpoints. H 0, the —null hypothesis: a statement of no difference between sample means or proportions or no difference between a sample mean or proportion and a population mean or proportion. In other words, the difference equals 0.
The null and alternative hypotheses are two competing claims that researchers weigh evidence for and against using a statistical test: Null hypothesis (H0): There's no effect in the population. Alternative hypothesis (HA): There's an effect in the population. The effect is usually the effect of the independent variable on the dependent ...
The null hypothesis and the alternative hypothesis are types of conjectures used in statistical tests to make statistical inferences, which are formal methods of reaching conclusions and separating scientific claims from statistical noise.. The statement being tested in a test of statistical significance is called the null hypothesis. The test of significance is designed to assess the strength ...
Null Hypothesis Examples. The null hypothesis assumes no difference between two groups or that the independent variable has no effect on the dependent variable. An example of the null hypothesis is that light color has no effect on plant growth. The null hypothesis (H 0) is the hypothesis that states there is no statistical difference between ...
There are 5 main steps in hypothesis testing: State your research hypothesis as a null hypothesis and alternate hypothesis (H o) and (H a or H 1). Collect data in a way designed to test the hypothesis. Perform an appropriate statistical test. Decide whether to reject or fail to reject your null hypothesis.
The null Hypothesis is represented as H 0, the Null Hypothesis symbolizes the absence of a measurable effect or difference in the variables under examination. Certainly, a simple example would be asserting that the mean score of a group is equal to a specified value like stating that the average IQ of a population is 100.
A null hypothesis is a hypothesis that says there is no statistical significance between the two variables in the hypothesis. It is the hypothesis that the researcher is trying to disprove.
A null hypothesis is a type of assumption used in statistics indicating that there is no significant difference between the samples from the underlying population. It is also known as the default hypothesis, represented by H 0. In contrast, there is a term "Alternative Hypothesis", represented by H 1. For every null hypothesis, there is an ...
Examples. A research hypothesis, in its plural form "hypotheses," is a specific, testable prediction about the anticipated results of a study, established at its outset. It is a key component of the scientific method. Hypotheses connect theory to data and guide the research process towards expanding scientific understanding.
One of the most basic concepts in statistics is hypothesis testing and something called The Null Hypothesis. This video breaks these concepts down into easy ...
Null Hypothesis Examples . Here is a simple example: A school principal claims that students in their school score an average of seven out of 10 in exams.
Simple Hypothesis: A simple Hypothesis is the one in which the relationship between two variables is predicted. One is an independent variable and the other is a dependent variable. A simple Hypothesis completely specifies the population distribution. An example of a simple Hypothesis is "Consuming sugar drinks daily leads to being overweight."
There are six types of hypotheses mainly the Simple hypothesis, Complex hypothesis, Directional hypothesis, Associative hypothesis, and Null hypothesis. Usually, the hypothesis is the start point of any scientific investigation, It gives the right direction to the process of investigation. ... Following is the difference between the null ...
If a variable in a linear model has p <0.05 p <0.05 (when R prints out stars), I would say the variable is a statistically significant part of the model. What does that translate to in terms of null hypothesis? Am I rejecting the null hypothesis that the coefficient for that variable is 0, 0, or am I accepting a null hypothesis that the ...
The meaning of NULL HYPOTHESIS is a statistical hypothesis to be tested and accepted or rejected in favor of an alternative; specifically : the hypothesis that an observed difference (as between the means of two samples) is due to chance alone and not due to a systematic cause.