algebra problem solving worksheet

Algebra Worksheets

Welcome to the Algebra worksheets page at Math-Drills.com, where unknowns are common and variables are the norm. On this page, you will find Algebra worksheets for middle school students on topics such as algebraic expressions, equations and graphing functions.

This page starts off with some missing numbers worksheets for younger students. We then get right into algebra by helping students recognize and understand the basic language related to algebra. The rest of the page covers some of the main topics you'll encounter in algebra units. Remember that by teaching students algebra, you are helping to create the future financial whizzes, engineers, and scientists that will solve all of our world's problems.

Algebra is much more interesting when things are more real. Solving linear equations is much more fun with a two pan balance, some mystery bags and a bunch of jelly beans. Algebra tiles are used by many teachers to help students understand a variety of algebra topics. And there is nothing like a set of co-ordinate axes to solve systems of linear equations.

Most Popular Algebra Worksheets this Week

$Rewriting Linear Equations in Standard Form$

Algebraic Properties, Rules and Laws Worksheets

$algebra problem solving worksheet$

The commutative law or commutative property states that you can change the order of the numbers in an arithmetic problem and still get the same results. In the context of arithmetic, it only works with addition or multiplication operations , but not mixed addition and multiplication. For example, 3 + 5 = 5 + 3 and 9 × 5 = 5 × 9. A fun activity that you can use in the classroom is to brainstorm non-numerical things from everyday life that are commutative and non-commutative. Putting on socks, for example, is commutative because you can put on the right sock then the left sock or you can put on the left sock then the right sock and you will end up with the same result. Putting on underwear and pants, however, is non-commutative.

The Commutative Law Worksheets The Commutative Law of Addition (Numbers Only) The Commutative Law of Addition (Some Variables) The Commutative Law of Multiplication (Numbers Only) The Commutative Law of Multiplication (Some Variables)

The associative law or associative property allows you to change the grouping of the operations in an arithmetic problem with two or more steps without changing the result. The order of the numbers stays the same in the associative law. As with the commutative law, it applies to addition-only or multiplication-only problems. It is best thought of in the context of order of operations as it requires that parentheses must be dealt with first. An example of the associative law is: (9 + 5) + 6 = 9 + (5 + 6). In this case, it doesn't matter if you add 9 + 5 first or 5 + 6 first, you will end up with the same result. Students might think of some examples from their experience such as putting items on a tray at lunch. They could put the milk and vegetables on their tray first then the sandwich or they could start with the vegetables and sandwich then put on the milk. If their tray looks the same both times, they will have modeled the associative law. Reading a book could be argued as either associative or nonassociative as one could potentially read the final chapters first and still understand the book as well as someone who read the book the normal way.

The Associative Law Worksheets The Associative Law of Addition (Whole Numbers Only) The Associative Law of Multiplication (Whole Numbers Only)

Inverse relationships worksheets cover a pre-algebra skill meant to help students understand the relationship between multiplication and division and the relationship between addition and subtraction.

Inverse Mathematical Relationships with One Blank Addition and Subtraction Easy Addition and Subtraction Harder All Multiplication and Division Facts 1 to 18 in color (no blanks) Multiplication and Division Range 1 to 9 Multiplication and Division Range 5 to 12 Multiplication and Division All Inverse Relationships Range 2 to 9 Multiplication and Division All Inverse Relationships Range 5 to 12 Multiplication and Division All Inverse Relationships Range 10 to 25
Inverse Mathematical Relationships with Two Blanks Addition and Subtraction (Sums 1-18) Addition and Subtraction Inverse Relationships with 1 Addition and Subtraction Inverse Relationships with 2 Addition and Subtraction Inverse Relationships with 3 Addition and Subtraction Inverse Relationships with 4 Addition and Subtraction Inverse Relationships with 5 Addition and Subtraction Inverse Relationships with 6 Addition and Subtraction Inverse Relationships with 7 Addition and Subtraction Inverse Relationships with 8 Addition and Subtraction Inverse Relationships with 9 Addition and Subtraction Inverse Relationships with 10 Addition and Subtraction Inverse Relationships with 11 Addition and Subtraction Inverse Relationships with 12 Addition and Subtraction Inverse Relationships with 13 Addition and Subtraction Inverse Relationships with 14 Addition and Subtraction Inverse Relationships with 15 Addition and Subtraction Inverse Relationships with 16 Addition and Subtraction Inverse Relationships with 17 Addition and Subtraction Inverse Relationships with 18

The distributive property is an important skill to have in algebra. In simple terms, it means that you can split one of the factors in multiplication into addends, multiply each addend separately, add the results, and you will end up with the same answer. It is also useful in mental math, an example of which should help illustrate the definition. Consider the question, 35 × 12. Splitting the 12 into 10 + 2 gives us an opportunity to complete the question mentally using the distributive property. First multiply 35 × 10 to get 350. Second, multiply 35 × 2 to get 70. Lastly, add 350 + 70 to get 420. In algebra, the distributive property becomes useful in cases where one cannot easily add the other factor before multiplying. For example, in the expression, 3(x + 5), x + 5 cannot be added without knowing the value of x. Instead, the distributive property can be used to multiply 3 × x and 3 × 5 to get 3x + 15.

Distributive Property Worksheets Distributive Property (Answers do not include exponents) Distributive Property (Some answers include exponents) Distributive Property (All answers include exponents)

Students should be able to substitute known values in for an unknown(s) in an expression and evaluate the expression's value.

Evaluating Expressions with Known Values Evaluating Expressions with One Variable, One Step and No Exponents Evaluating Expressions with One Variable and One Step Evaluating Expressions with One Variable and Two Steps Evaluating Expressions with Up to Two Variables and Two Steps Evaluating Expressions with Up to Two Variables and Three Steps Evaluating Expressions with Up to Three Variables and Four Steps Evaluating Expressions with Up to Three Variables and Five Steps

As the title says, these worksheets include only basic exponent rules questions. Each question only has two exponents to deal with; complicated mixed up terms and things that a more advanced student might work out are left alone. For example, 4 2 is (2 2 ) 2 = 2 4 , but these worksheets just leave it as 4 2 , so students can focus on learning how to multiply and divide exponents more or less in isolation.

Exponent Rules for Multiplying, Dividing and Powers Mixed Exponent Rules (All Positive) Mixed Exponent Rules (With Negatives) Multiplying Exponents (All Positive) Multiplying Exponents (With Negatives) Multiplying the Same Exponent with Different Bases (All Positive) Multiplying the Same Exponent with Different Bases (With Negatives) Dividing Exponents with a Greater Exponent in Dividend (All Positive) Dividing Exponents with a Greater Exponent in Dividend (With Negatives) Dividing Exponents with a Greater Exponent in Divisor (All Positive) Dividing Exponents with a Greater Exponent in Divisor (With Negatives) Powers of Exponents (All Positive) Powers of Exponents (With Negatives)

Knowing the language of algebra can help to extract meaning from word problems and to situations outside of school. In these worksheets, students are challenged to convert phrases into algebraic expressions.

Translating Algebraic Phrases into Expressions Translating Algebraic Phrases into Expressions (Simple Version) Translating Algebraic Phrases into Expressions (Complex Version)

Combining like terms is something that happens a lot in algebra. Students can be introduced to the topic and practice a bit with these worksheets. The bar is raised with the adding and subtracting versions that introduce parentheses into the expressions. For students who have a good grasp of fractions, simplifying simple algebraic fractions worksheets present a bit of a challenge over the other worksheets in this section.

Simplifying Expressions by Combining Like Terms Simplifying Linear Expressions with 3 terms Simplifying Linear Expressions with 4 terms Simplifying Linear Expressions with 5 terms Simplifying Linear Expressions with 6 to 10 terms
Simplifying Expressions by Combining Like Terms with Some Arithmetic Adding and simplifying linear expressions Adding and simplifying linear expressions with multipliers Adding and simplifying linear expressions with some multipliers . Subtracting and simplifying linear expressions Subtracting and simplifying linear expressions with multipliers Subtracting and simplifying linear expressions with some multipliers Mixed adding and subtracting and simplifying linear expressions Mixed adding and subtracting and simplifying linear expressions with multipliers Mixed adding and subtracting and simplifying linear expressions with some multipliers Simplify simple algebraic fractions (easier) Simplify simple algebraic fractions (harder)
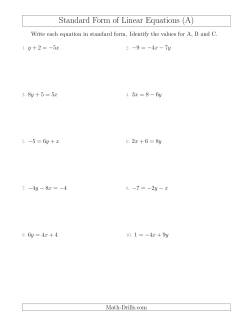
Rewriting Linear Equations Rewrite Linear Equations in Standard Form Convert Linear Equations from Standard to Slope-Intercept Form Convert Linear Equations from Slope-Intercept to Standard Form Convert Linear Equations Between Standard and Slope-Intercept Form
Rewriting Formulas Rewriting Formulas (addition and subtraction; about one step) Rewriting Formulas (addition and subtraction; about two steps) Rewriting Formulas ( multiplication and division ; about one step)

Linear Expressions and Equations

$algebra problem solving worksheet$

In these worksheets, the unknown is limited to the question side of the equation which could be on the left or the right of equal sign.

Missing Numbers in Equations with Blanks as Unknowns Missing Numbers in Equations ( All Operations ; Range 1 to 9 ; Blanks Never in Answer Position ) Missing Numbers in Equations ( All Operations ; Range 1 to 9 ; Blanks in Any Position ) Missing Numbers in Equations ( All Operations ; Range 1 to 20 ; Blanks Never in Answer Position ) Missing Numbers in Equations ( All Operations ; Range 1 to 20 ; Blanks in Any Position ) Missing Numbers in Equations ( Addition Only ; Range 1 to 9 ; Blanks Never in Answer Position ) Missing Numbers in Equations ( Addition Only ; Range 1 to 9 ; Blanks in Any Position ) Missing Numbers in Equations ( Addition Only ; Range 1 to 20 ; Blanks in Any Position ) Missing Numbers in Equations ( Subtraction Only ; Range 1 to 9 ; Blanks Never in Answer Position ) Missing Numbers in Equations ( Subtraction Only ; Range 1 to 9 ; Blanks in Any Position ) Missing Numbers in Equations ( Subtraction Only ; Range 1 to 20 ; Blanks in Any Position ) Missing Numbers in Equations ( Multiplication Only ; Range 1 to 9 ; Blanks Never in Answer Position ) Missing Numbers in Equations ( Multiplication Only ; Range 1 to 9 ; Blanks in Any Position ) Missing Numbers in Equations ( Multiplication Only ; Range 1 to 20 ; Blanks in Any Position ) Missing Numbers in Equations ( Division Only ; Range 1 to 9 ; Blanks Never in Answer Position ) Missing Numbers in Equations ( Division Only ; Range 1 to 9 ; Blanks in Any Position ) Missing Numbers in Equations ( Division Only ; Range 1 to 20 ; Blanks in Any Position )
Missing Numbers in Equations with Symbols as Unknowns Missing Numbers in Equations ( All Operations ; Range 1 to 9 ; Symbols Never in Answer Position ) Missing Numbers in Equations ( All Operations ; Range 1 to 9 ; Symbols in Any Position ) Missing Numbers in Equations ( All Operations ; Range 1 to 20 ; Symbols Never in Answer Position ) Missing Numbers in Equations ( All Operations ; Range 1 to 20 ; Symbols in Any Position ) Missing Numbers in Equations ( Addition Only ; Range 1 to 9 ; Symbols Never in Answer Position ) Missing Numbers in Equations ( Addition Only ; Range 1 to 9 ; Symbols in Any Position ) Missing Numbers in Equations ( Addition Only ; Range 1 to 20 ; Symbols in Any Position ) Missing Numbers in Equations ( Subtraction Only ; Range 1 to 9 ; Symbols Never in Answer Position ) Missing Numbers in Equations ( Subtraction Only ; Range 1 to 9 ; Symbols in Any Position ) Missing Numbers in Equations ( Subtraction Only ; Range 1 to 20 ; Symbols in Any Position ) Missing Numbers in Equations ( Multiplication Only ; Range 1 to 9 ; Symbols Never in Answer Position ) Missing Numbers in Equations ( Multiplication Only ; Range 1 to 9 ; Symbols in Any Position ) Missing Numbers in Equations ( Multiplication Only ; Range 1 to 20 ; Symbols in Any Position ) Missing Numbers in Equations ( Division Only ; Range 1 to 9 ; Symbols Never in Answer Position ) Missing Numbers in Equations ( Division Only ; Range 1 to 9 ; Symbols in Any Position ) Missing Numbers in Equations ( Division Only ; Range 1 to 20 ; Symbols in Any Position )
Solving Equations with Addition and Symbols as Unknowns Equalities with Addition (0 to 9) Symbol Unknowns Equalities with Addition (1 to 12) Symbol Unknowns Equalities with Addition (1 to 15) Symbol Unknowns Equalities with Addition (1 to 25) Symbol Unknowns Equalities with Addition (1 to 99) Symbol Unknowns
Missing Numbers in Equations with Variables as Unknowns Missing Numbers in Equations ( All Operations ; Range 1 to 9 ; Variables Never in Answer Position ) Missing Numbers in Equations ( All Operations ; Range 1 to 9 ; Variables in Any Position ) Missing Numbers in Equations ( All Operations ; Range 1 to 20 ; Variables Never in Answer Position ) Missing Numbers in Equations ( All Operations ; Range 1 to 20 ; Variables in Any Position ) Missing Numbers in Equations ( Addition Only ; Range 1 to 9 ; Variables Never in Answer Position ) Missing Numbers in Equations ( Addition Only ; Range 1 to 9 ; Variables in Any Position ) Missing Numbers in Equations ( Addition Only ; Range 1 to 20 ; Variables in Any Position ) Missing Numbers in Equations ( Subtraction Only ; Range 1 to 9 ; Variables Never in Answer Position ) Missing Numbers in Equations ( Subtraction Only ; Range 1 to 9 ; Variables in Any Position ) Missing Numbers in Equations ( Subtraction Only ; Range 1 to 20 ; Variables in Any Position ) Missing Numbers in Equations ( Multiplication Only ; Range 1 to 9 ; Variables Never in Answer Position ) Missing Numbers in Equations ( Multiplication Only ; Range 1 to 9 ; Variables in Any Position ) Missing Numbers in Equations ( Multiplication Only ; Range 1 to 20 ; Variables in Any Position ) Missing Numbers in Equations ( Division Only ; Range 1 to 9 ; Variables Never in Answer Position ) Missing Numbers in Equations ( Division Only ; Range 1 to 9 ; Variables in Any Position ) Missing Numbers in Equations ( Division Only ; Range 1 to 20 ; Variables in Any Position )
Solving Simple Linear Equations Solving Simple Linear Equations with Values from -9 to 9 (Unknown on Left Side) Solving Simple Linear Equations with Values from -99 to 99 (Unknown on Left Side) Solving Simple Linear Equations with Values from -9 to 9 (Unknown on Right or Left Side) Solving Simple Linear Equations with Values from -99 to 99 (Unknown on Right or Left Side)
Determining Linear Equations from Slopes, y-intercepts and Points Determine a Linear Equation from the Slope and y-intercept Determine a Linear Equation from the Slope and a Point Determine a Linear Equation from Two Points Determine a Linear Equation from Two Points by Graphing

Graphing linear equations and reading existing graphs give students a visual representation that is very useful in understanding the concepts of slope and y-intercept.

Graphing Linear Equations Graph Slope-Intercept Equations
Determining Linear Equations from Graphs Determine the Equation from a Graph Determine the Slope from a Graph Determine the y-intercept from a Graph Determine the x-intercept from a Graph Determine the slope and y-intercept from a Graph Determine the slope and intercepts from a Graph Determine the slope, intercepts and equation from a Graph

Solving linear equations with jelly beans is a fun activity to try with students first learning algebraic concepts. Ideally, you will want some opaque bags with no mass, but since that isn't quite possible (the no mass part), there is a bit of a condition here that will actually help students understand equations better. Any bags that you use have to be balanced on the other side of the equation with empty ones.

Probably the best way to illustrate this is through an example. Let's use 3 x + 2 = 14. You may recognize the x as the unknown which is actually the number of jelly beans we put in each opaque bag. The 3 in the 3 x means that we need three bags. It's best to fill the bags with the required number of jelly beans out of view of the students, so they actually have to solve the equation.

On one side of the two-pan balance, place the three bags with x jelly beans in each one and two loose jelly beans to represent the + 2 part of the equation. On the other side of the balance, place 14 jelly beans and three empty bags which you will note are required to "balance" the equation properly. Now comes the fun part... if students remove the two loose jelly beans from one side of the equation, things become unbalanced, so they need to remove two jelly beans from the other side of the balance to keep things even. Eating the jelly beans is optional. The goal is to isolate the bags on one side of the balance without any loose jelly beans while still balancing the equation.

The last step is to divide the loose jelly beans on one side of the equation into the same number of groups as there are bags. This will probably give you a good indication of how many jelly beans there are in each bag. If not, eat some and try again. Now, we realize this won't work for every linear equation as it is hard to have negative jelly beans, but it is another teaching strategy that you can use for algebra.

Despite all appearances, equations of the type a/ x are not linear. Instead, they belong to a different kind of equations. They are good for combining them with linear equations, since they introduce the concept of valid and invalid answers for an equation (what will be later called the domain of a function). In this case, the invalid answers for equations in the form a/ x , are those that make the denominator become 0.

Solving Linear Equations Combining Like Terms and Solving Simple Linear Equations Solving a x = c Linear Equations Solving a x = c Linear Equations including negatives Solving x /a = c Linear Equations Solving x /a = c Linear Equations including negatives Solving a/ x = c Linear Equations Solving a/ x = c Linear Equations including negatives Solving a x + b = c Linear Equations Solving a x + b = c Linear Equations including negatives Solving a x - b = c Linear Equations Solving a x - b = c Linear Equations including negatives Solving a x ± b = c Linear Equations Solving a x ± b = c Linear Equations including negatives Solving x /a ± b = c Linear Equations Solving x /a ± b = c Linear Equations including negatives Solving a/ x ± b = c Linear Equations Solving a/ x ± b = c Linear Equations including negatives Solving various a/ x ± b = c and x /a ± b = c Linear Equations Solving various a/ x ± b = c and x /a ± b = c Linear Equations including negatives Solving linear equations of all types Solving linear equations of all types including negatives

Algebra rectangles are rectangles that use linear expressions for the side measurements. With a known value (such as the perimeter), students create an algebraic equation that they can solve to determine the value of the unknown (x) and use it to determine the side lengths and area of the rectangle. The terminology in identifying the various options for worksheets use the standard equation y = mx + b where m is the coeffient of x that is generally a known value.

Algebra Rectangles Algebra Rectangles -- Determining the Value of x, Length, Width and Area Using Algebraic Sides and the Perimeter -- m Range [1,1] Algebra Worksheet -{}- Algebra Rectangles -- Determining the Value of x, Length, Width and Area Using Algebraic Sides and the Perimeter -- m Range [2,9] Algebra Worksheet -{}- Algebra Rectangles -- Determining the Value of x, Length, Width and Area Using Algebraic Sides and the Perimeter -- m Range [2,9] or [-9,-2] Algebra Worksheet -{}- Algebra Rectangles -- Determining the Value of x, Length, Width and Area Using Algebraic Sides and the Perimeter -- m Range [2,9] or [-9,-2] -- Inverse m Possible

Linear Systems

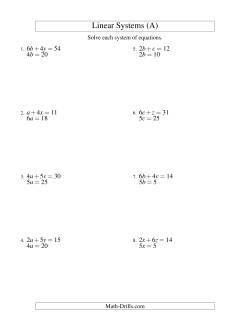
$algebra problem solving worksheet$

Solving Systems of Linear Equations Easy Linear Systems with Two Variables Easy Linear Systems with Two Variables including negative values Linear Systems with Two Variables Linear Systems with Two Variables including negative values Easy Linear Systems with Three Variables; Easy Easy Linear Systems with Three Variables including negative values Linear Systems with Three Variables Linear Systems with Three Variables including negative values
Solving Systems of Linear Equations by Graphing Solve Linear Systems by Graphing (Solutions in first quadrant only) Solve Standard Linear Systems by Graphing Solve Slope-Intercept Linear Systems by Graphing Solve Various Linear Systems by Graphing Identify the Dependent Linear System by Graphing Identify the Inconsistent Linear System by Graphing

Quadratic Expressions and Equations

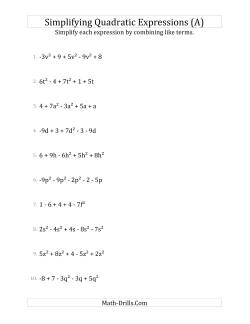
$algebra problem solving worksheet$

Simplifying (Combining Like Terms) Quadratic Expressions Simplifying quadratic expressions with 5 terms Simplifying quadratic expressions with 6 terms Simplifying quadratic expressions with 7 terms Simplifying quadratic expressions with 8 terms Simplifying quadratic expressions with 9 terms Simplifying quadratic expressions with 10 terms Simplifying quadratic expressions with 5 to 10 terms
Adding/Subtracting and Simplifying Quadratic Expressions Adding and simplifying quadratic expressions. Adding and simplifying quadratic expressions with multipliers. Adding and simplifying quadratic expressions with some multipliers. Subtracting and simplifying quadratic expressions. Subtracting and simplifying quadratic expressions with multipliers. Subtracting and simplifying quadratic expressions with some multipliers. Mixed adding and subtracting and simplifying quadratic expressions. Mixed adding and subtracting and simplifying quadratic expressions with multipliers. Mixed adding and subtracting and simplifying quadratic expressions with some multipliers.
Multiplying Factors to Get Quadratic Expressions Multiplying Factors of Quadratics with Coefficients of 1 Multiplying Factors of Quadratics with Coefficients of 1 or -1 Multiplying Factors of Quadratics with Coefficients of 1, or 2 Multiplying Factors of Quadratics with Coefficients of 1, -1, 2 or -2 Multiplying Factors of Quadratics with Coefficients up to 9 Multiplying Factors of Quadratics with Coefficients between -9 and 9

The factoring quadratic expressions worksheets in this section provide many practice questions for students to hone their factoring strategies. If you would rather worksheets with quadratic equations, please see the next section. These worksheets come in a variety of levels with the easier ones are at the beginning. The 'a' coefficients referred to below are the coefficients of the x 2 term as in the general quadratic expression: ax 2 + bx + c. There are also worksheets in this section for calculating sum and product and for determining the operands for sum and product pairs.

Factoring Quadratic Expressions Factoring Quadratic Expressions with Positive 'a' coefficients of 1 Factoring Quadratic Expressions with Positive or Negative 'a' coefficients of 1 Factoring Quadratic Expressions with Positive or Negative 'a' coefficients of 1 with a Common Factor Step Factoring Quadratic Expressions with Positive 'a' coefficients up to 4 Factoring Quadratic Expressions with Positive or Negative 'a' coefficients up to 4 Factoring Quadratic Expressions with Positive or Negative 'a' coefficients up to 4 with a Common Factor Step Factoring Quadratic Expressions with Positive 'a' coefficients up to 5 Factoring Quadratic Expressions with Positive or Negative 'a' coefficients up to 5 Factoring Quadratic Expressions with Positive or Negative 'a' coefficients up to 5 with a Common Factor Step Factoring Quadratic Expressions with Positive 'a' coefficients up to 9 Factoring Quadratic Expressions with Positive or Negative 'a' coefficients up to 9 Factoring Quadratic Expressions with Positive or Negative 'a' coefficients up to 9 with a Common Factor Step Factoring Quadratic Expressions with Positive 'a' coefficients up to 81 Factoring Quadratic Expressions with Positive or Negative 'a' coefficients up to 81 Factoring Quadratic Expressions with Positive or Negative 'a' coefficients up to 81 with a Common Factor Step Calculating Sum and Product (Operand Range 0 to 9 ) ✎ Calculating Sum and Product (Operand Range 1 to 9 ) ✎ Calculating Sum and Product (Operand Range 0 to 9 Including Negatives ) ✎ Calculating Sum and Product (Operand Range 1 to 9 Including Negatives ) ✎ Calculating Sum and Product (Operand Range -20 to 20 ) ✎ Calculating Sum and Product (Operand Range -99 to 99 ) ✎ Determining Operands from Sum and Product Pairs (Operand Range 0 to 9 ) ✎ Determining Operands from Sum and Product Pairs (Operand Range 1 to 9 ) ✎ Determining Operands from Sum and Product Pairs (Operand Range 0 to 12 ) ✎ Determining Operands from Sum and Product Pairs (Operand Range 1 to 12 ) ✎ Determining Operands from Sum and Product Pairs (Operand Range 0 to 9 Including Negatives ) ✎ Determining Operands from Sum and Product Pairs (Operand Range 1 to 9 Including Negatives ) ✎ Determining Operands from Sum and Product Pairs (Operand Range -20 to 20 ) ✎ Determining Operands from Sum and Product Pairs (Operand Range -99 to 99 ) ✎

Whether you use trial and error, completing the square or the general quadratic formula, these worksheets include a plethora of practice questions with answers. In the first section, the worksheets include questions where the quadratic expressions equal 0. This makes the process similar to factoring quadratic expressions, with the additional step of finding the values for x when the expression is equal to 0. In the second section, the expressions are generally equal to something other than x, so there is an additional step at the beginning to make the quadratic expression equal zero.

Solving Quadratic Equations that Equal Zero Solving Quadratic Equations with Positive 'a' coefficients of 1 Solving Quadratic Equations with Positive or Negative 'a' coefficients of 1 Solving Quadratic Equations with Positive or Negative 'a' coefficients of 1 with a Common Factor Step Solving Quadratic Equations with Positive 'a' coefficients up to 4 Solving Quadratic Equations with Positive or Negative 'a' coefficients up to 4 Solving Quadratic Equations with Positive or Negative 'a' coefficients up to 4 with a Common Factor Step Solving Quadratic Equations with Positive 'a' coefficients up to 5 Solving Quadratic Equations with Positive or Negative 'a' coefficients up to 5 Solving Quadratic Equations with Positive or Negative 'a' coefficients up to 5 with a Common Factor Step Solving Quadratic Equations with Positive 'a' coefficients up to 9 Solving Quadratic Equations with Positive or Negative 'a' coefficients up to 9 Solving Quadratic Equations with Positive or Negative 'a' coefficients up to 9 with a Common Factor Step Solving Quadratic Equations with Positive 'a' coefficients up to 81 Solving Quadratic Equations with Positive or Negative 'a' coefficients up to 81 Solving Quadratic Equations with Positive or Negative 'a' coefficients up to 81 with a Common Factor Step
Solving Quadratic Equations that Equal an Integer Solving Quadratic Equations for x ("a" coefficients of 1) Solving Quadratic Equations for x ("a" coefficients of 1 or -1) Solving Quadratic Equations for x ("a" coefficients up to 4) Solving Quadratic Equations for x ("a" coefficients between -4 and 4) Solving Quadratic Equations for x ("a" coefficients up to 81) Solving Quadratic Equations for x ("a" coefficients between -81 and 81)

Other Polynomial and Monomial Expressions & Equations

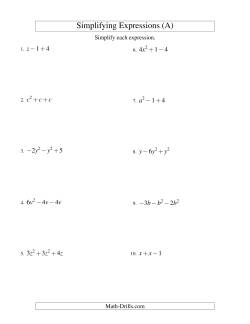
$algebra problem solving worksheet$

Simplifying Polynomials That Involve Addition And Subtraction Addition and Subtraction; 1 variable; 3 terms Addition and Subtraction; 1 variable; 4 terms Addition and Subtraction; 2 variables; 4 terms Addition and Subtraction; 2 variables; 5 terms Addition and Subtraction; 2 variables; 6 terms
Simplifying Polynomials That Involve Multiplication And Division Multiplication and Division; 1 variable; 3 terms Multiplication and Division; 1 variable; 4 terms Multiplication and Division; 2 variables; 4 terms Multiplication and Division; 2 variables; 5 terms
Simplifying Polynomials That Involve Addition, Subtraction, Multiplication And Division All Operations; 1 variable; 3 terms All Operations; 1 variable; 4 terms All Operations; 2 variables; 4 terms All Operations; 2 variables; 5 terms All Operations (Challenge)
Factoring Expressions That Do Not Include A Squared Variable Factoring Non-Quadratic Expressions with No Squares, Simple Coefficients, and Positive Multipliers Factoring Non-Quadratic Expressions with No Squares, Simple Coefficients, and Negative and Positive Multipliers Factoring Non-Quadratic Expressions with No Squares, Compound Coefficients, and Positive Multipliers Factoring Non-Quadratic Expressions with No Squares, Compound Coefficients, and Negative and Positive Multipliers
Factoring Expressions That Always Include A Squared Variable Factoring Non-Quadratic Expressions with All Squares, Simple Coefficients, and Positive Multipliers Factoring Non-Quadratic Expressions with All Squares, Simple Coefficients, and Negative and Positive Multipliers Factoring Non-Quadratic Expressions with All Squares, Compound Coefficients, and Positive Multipliers Factoring Non-Quadratic Expressions with All Squares, Compound Coefficients, and Negative and Positive Multipliers
Factoring Expressions That Sometimes Include Squared Variables Factoring Non-Quadratic Expressions with Some Squares, Simple Coefficients, and Positive Multipliers Factoring Non-Quadratic Expressions with Some Squares, Simple Coefficients, and Negative and Positive Multipliers Factoring Non-Quadratic Expressions with Some Squares, Compound Coefficients, and Positive Multipliers Factoring Non-Quadratic Expressions with Some Squares, Compound Coefficients, and Negative and Positive Multipliers
Multiplying Polynomials With Two Factors Multiplying a monomial by a binomial Multiplying two binomials Multiplying a monomial by a trinomial Multiplying a binomial by a trinomial Multiplying two trinomials Multiplying two random mon/polynomials
Multiplying Polynomials With Three Factors Multiplying a monomial by two binomials Multiplying three binomials Multiplying two binomials by a trinomial Multiplying a binomial by two trinomials Multiplying three trinomials Multiplying three random mon/polynomials

Inequalities

$algebra problem solving worksheet$

Writing The Inequality That Matches The Graph Writing Inequalities for Graphs
Graphing Inequalities On Number Lines Graphing Inequalities (Basic)
Solving Linear Inequalities Solving Inequalities Including a Third Term Solving Inequalities Including a Third Term and Multiplication Solving Inequalities Including a Third Term, Multiplication and Division

Skip to main content
Skip to primary sidebar
Skip to footer

Additional menu

Khan Academy Blog

Free Math Worksheets — Over 100k free practice problems on Khan Academy

Looking for free math worksheets.

You’ve found something even better!

That’s because Khan Academy has over 100,000 free practice questions. And they’re even better than traditional math worksheets – more instantaneous, more interactive, and more fun!

Just choose your grade level or topic to get access to 100% free practice questions:

Kindergarten, basic geometry, pre-algebra, algebra basics, high school geometry.

Trigonometry

Statistics and probability

High school statistics, ap®︎/college statistics, precalculus, differential calculus, integral calculus, ap®︎/college calculus ab, ap®︎/college calculus bc, multivariable calculus, differential equations, linear algebra.

Addition and subtraction
Place value (tens and hundreds)
Addition and subtraction within 20
Addition and subtraction within 100
Addition and subtraction within 1000
Measurement and data
Counting and place value
Measurement and geometry
Place value
Measurement, data, and geometry
Add and subtract within 20
Add and subtract within 100
Add and subtract within 1,000
Money and time
Measurement
Intro to multiplication
1-digit multiplication
Addition, subtraction, and estimation
Intro to division
Understand fractions
Equivalent fractions and comparing fractions
More with multiplication and division
Arithmetic patterns and problem solving
Quadrilaterals
Represent and interpret data
Multiply by 1-digit numbers
Multiply by 2-digit numbers
Factors, multiples and patterns
Add and subtract fractions
Multiply fractions
Understand decimals
Plane figures
Measuring angles
Area and perimeter
Units of measurement
Decimal place value
Add decimals
Subtract decimals
Multi-digit multiplication and division
Divide fractions
Multiply decimals
Divide decimals
Powers of ten
Coordinate plane
Algebraic thinking
Converting units of measure
Properties of shapes
Ratios, rates, & percentages
Arithmetic operations
Negative numbers
Properties of numbers
Variables & expressions
Equations & inequalities introduction
Data and statistics
Negative numbers: addition and subtraction
Negative numbers: multiplication and division
Fractions, decimals, & percentages
Rates & proportional relationships
Expressions, equations, & inequalities
Numbers and operations
Solving equations with one unknown
Linear equations and functions
Systems of equations
Geometric transformations
Data and modeling
Volume and surface area
Pythagorean theorem
Transformations, congruence, and similarity
Arithmetic properties
Factors and multiples
Reading and interpreting data
Negative numbers and coordinate plane
Ratios, rates, proportions
Equations, expressions, and inequalities
Exponents, radicals, and scientific notation
Foundations
Algebraic expressions
Linear equations and inequalities
Graphing lines and slope
Expressions with exponents
Quadratics and polynomials
Equations and geometry
Algebra foundations
Solving equations & inequalities
Working with units
Linear equations & graphs
Forms of linear equations
Inequalities (systems & graphs)
Absolute value & piecewise functions
Exponents & radicals
Exponential growth & decay
Quadratics: Multiplying & factoring
Quadratic functions & equations
Irrational numbers
Performing transformations
Transformation properties and proofs
Right triangles & trigonometry
Non-right triangles & trigonometry (Advanced)
Analytic geometry
Conic sections
Solid geometry
Polynomial arithmetic
Complex numbers
Polynomial factorization
Polynomial division
Polynomial graphs
Rational exponents and radicals
Exponential models
Transformations of functions
Rational functions
Trigonometric functions
Non-right triangles & trigonometry
Trigonometric equations and identities
Analyzing categorical data
Displaying and comparing quantitative data
Summarizing quantitative data
Modeling data distributions
Exploring bivariate numerical data
Study design
Probability
Counting, permutations, and combinations
Random variables
Sampling distributions
Confidence intervals
Significance tests (hypothesis testing)
Two-sample inference for the difference between groups
Inference for categorical data (chi-square tests)
Advanced regression (inference and transforming)
Analysis of variance (ANOVA)
Scatterplots
Data distributions
Two-way tables
Binomial probability
Normal distributions
Displaying and describing quantitative data
Inference comparing two groups or populations
Chi-square tests for categorical data
More on regression
Prepare for the 2020 AP®︎ Statistics Exam
AP®︎ Statistics Standards mappings
Polynomials
Composite functions
Probability and combinatorics
Limits and continuity
Derivatives: definition and basic rules
Derivatives: chain rule and other advanced topics
Applications of derivatives
Analyzing functions
Parametric equations, polar coordinates, and vector-valued functions
Applications of integrals
Differentiation: definition and basic derivative rules
Differentiation: composite, implicit, and inverse functions
Contextual applications of differentiation
Applying derivatives to analyze functions
Integration and accumulation of change
Applications of integration
AP Calculus AB solved free response questions from past exams
AP®︎ Calculus AB Standards mappings
Infinite sequences and series
AP Calculus BC solved exams
AP®︎ Calculus BC Standards mappings
Integrals review
Integration techniques
Thinking about multivariable functions
Derivatives of multivariable functions
Applications of multivariable derivatives
Integrating multivariable functions
Green’s, Stokes’, and the divergence theorems
First order differential equations
Second order linear equations
Laplace transform
Vectors and spaces
Matrix transformations
Alternate coordinate systems (bases)

Frequently Asked Questions about Khan Academy and Math Worksheets

Why is khan academy even better than traditional math worksheets.

Khan Academy’s 100,000+ free practice questions give instant feedback, don’t need to be graded, and don’t require a printer.

What do Khan Academy’s interactive math worksheets look like?

Here’s an example:

What are teachers saying about Khan Academy’s interactive math worksheets?

“My students love Khan Academy because they can immediately learn from their mistakes, unlike traditional worksheets.”

Is Khan Academy free?

Khan Academy’s practice questions are 100% free—with no ads or subscriptions.

What do Khan Academy’s interactive math worksheets cover?

Our 100,000+ practice questions cover every math topic from arithmetic to calculus, as well as ELA, Science, Social Studies, and more.

Is Khan Academy a company?

Khan Academy is a nonprofit with a mission to provide a free, world-class education to anyone, anywhere.

Want to get even more out of Khan Academy?

Then be sure to check out our teacher tools . They’ll help you assign the perfect practice for each student from our full math curriculum and track your students’ progress across the year. Plus, they’re also 100% free — with no subscriptions and no ads.

Get Khanmigo

The best way to learn and teach with AI is here. Ace the school year with our AI-powered guide, Khanmigo.

For learners For teachers For parents

Free Math Printable Worksheets with Answer Keys and Activities

Other free resources.

Feel free to download and enjoy these free worksheets on functions and relations. Each one has model problems worked out step by step, practice problems, as well as challenge questions at the sheets end. Plus each one comes with an answer key.

Long Division with Remainders
Long Division with Remainders #2 (Zeros in the Quotient)
Long Division with 2 Digit Divisors
Whole Number by Unit Fraction
Equation of Circle
Simplify Imaginary Numbers
Adding and Subtracting Complex Numbers
Multiplying Complex Numbers
Dividing Complex Numbers
Dividing Complex Number (Advanced)
End of Unit, Review Sheet
Distance Formula
Simplify Rational Exponents (Algebra 2)
Solve Equations with Rational Exponents (Algebra 2)
Solve Equations with variables in Exponents (Algebra 2)
Exponential Growth (no answer key on this one, sorry)
Compound Interest Worksheet #1 (No logs)
Compound Interest Worksheet (Logarithms required)
Factor Trinomials Worksheet
Factor by Grouping
Domain and Range (Algebra 1)
Functions vs Relations (Distinguish function from relation, state domain etc..) (Algebra 2)
Evaluating Functions (Algebra 2)
1 to 1 Functions (Algebra 2)
Composition of Functions (Algebra 2)
Inverse Functions Worksheet (Algebra 2)
Operations with Functions (Algebra 2)
Functions Review Worksheet (Algebra 2)
Logarithmic Equations
Properties of Logarithms Worksheet
Product Rule of Logarithms
Power Rule of Logarithms
Quotient Rule of Logarithms
Solve Quadratic Equations by Factoring
Quadratic Formula Worksheets (3 different sheets)
Quadratic Formula Worksheet (Real solutions)
Quadratic Formula (Complex solutions)
Quadratic Formula (Both real and complex solutions)
Discriminant and Nature of the Roots
Solve Quadratic Equations by Completing the Square
Sum and Product of Roots
Radical Equations
Mixed Problems on Writing Equations of Lines
Slope Intercept Form Worksheet
Standard Form Worksheet
Point Slope Worksheet
Write Equation of Line from the Slope and 1 Point
Write Equation of Line From Two Points
Equation of Line Parallel to Another Line and Through a Point
Equation of Line Perpendicular to Another Line and Through a Point
Slope of a Line
Perpendicular Bisector of Segment
Write Equation of Line Mixed Review
Word Problems
Multiplying Monomials Worksheet
Multiplying and Dividing Monomials Sheet
Adding and Subtracting Polynomials worksheet
Multiplying Monomials with Polynomials Worksheet
Multiplying Binomials Worksheet
Multiplying Polynomials
Simplifying Polynomials
Factoring Trinomials
Operations with Polynomials Worksheet
Dividing Radicals
Simplify Radicals Worksheet
Adding Radicals
Multiplying Radicals Worksheet
Radicals Review (Mixed review worksheet on radicals and square roots)
Rationalizing the Denominator (Algebra 2)
Radical Equations (Algebra 2)
Solve Systems of Equations Graphically
Solve Systems of Equations by Elimination
Solve by Substitution
Solve Systems of Equations (Mixed Review)
Activity on Systems of Equations (Create an advertisement for your favorite method to Solve Systems of Equations )
Real World Connections (Compare cell phone plans)
Identifying Fractions

Trigonomnetry

Law of Sines and Cosines Worksheet (This sheet is a summative worksheet that focuses on deciding when to use the law of sines or cosines as well as on using both formulas to solve for a single triangle's side or angle)
Law of Sines
Ambiguous Case of the Law of Sines
Law of Cosines
Vector Worksheet
Sine, Cosine, Tangent, to Find Side Length
Sine, Cosine, Tangent Chart
Inverse Trig Functions
Real World Applications of SOHCATOA
Mixed Review
Unit Circle Worksheet
Graphing Sine and Cosine Worksheet
Sine Cosine Graphs with Vertical Translations
Sine, Cosine, Tangent Graphs with Phase Shifts
Sine, Cosine, Tangent Graphs with Change in Period, Amplitude and Phase Shifts (All Translations)
Tangent Equation, Graph Worksheet
Graphing Sine, Cosine, Tangent with Change in Period
Cumulative, Summative Worksheet on Periodic Trig Functions - period, amplitude, phase shift, radians, degrees,unit circle
Ratio and Proportion
Similar Polygons
Area of Triangle
Interior Angles of Polygons
Exterior Angles of Polygons

Identifying Fractions Worksheet
Associated Powerpoint
Simplify Fractions Worksheet (Regular Difficulty)
Associated PowerPoint
Simplify Fractions Worksheet (Challenging Difficulty level for advanced learners)
System of Linear Equations Worksheet
System of Linear Equations - Real World Application
Compositions of Reflections. Reflections Over Intersecting Lines as Rotations

All of these worksheets and activities are available for free so long as they are used solely for educational, noncommercial purposes and are not distributed outside of a specific teacher's classroom.