
- school Campus Bookshelves
- menu_book Bookshelves
- perm_media Learning Objects
- login Login
- how_to_reg Request Instructor Account
- hub Instructor Commons

Margin Size
- Download Page (PDF)
- Download Full Book (PDF)
- Periodic Table
- Physics Constants
- Scientific Calculator
- Reference & Cite
- Tools expand_more
- Readability
selected template will load here
This action is not available.

Module 1: Problem Solving Strategies
- Last updated
- Save as PDF
- Page ID 10352
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
Unlike exercises, there is never a simple recipe for solving a problem. You can get better and better at solving problems, both by building up your background knowledge and by simply practicing. As you solve more problems (and learn how other people solved them), you learn strategies and techniques that can be useful. But no single strategy works every time.
Pólya’s How to Solve It
George Pólya was a great champion in the field of teaching effective problem solving skills. He was born in Hungary in 1887, received his Ph.D. at the University of Budapest, and was a professor at Stanford University (among other universities). He wrote many mathematical papers along with three books, most famously, “How to Solve it.” Pólya died at the age 98 in 1985.1
1. Image of Pólya by Thane Plambeck from Palo Alto, California (Flickr) [CC BY

In 1945, Pólya published the short book How to Solve It , which gave a four-step method for solving mathematical problems:
First, you have to understand the problem.
After understanding, then make a plan.
Carry out the plan.
Look back on your work. How could it be better?
This is all well and good, but how do you actually do these steps?!?! Steps 1. and 2. are particularly mysterious! How do you “make a plan?” That is where you need some tools in your toolbox, and some experience to draw upon.
Much has been written since 1945 to explain these steps in more detail, but the truth is that they are more art than science. This is where math becomes a creative endeavor (and where it becomes so much fun). We will articulate some useful problem solving strategies, but no such list will ever be complete. This is really just a start to help you on your way. The best way to become a skilled problem solver is to learn the background material well, and then to solve a lot of problems!
Problem Solving Strategy 1 (Guess and Test)
Make a guess and test to see if it satisfies the demands of the problem. If it doesn't, alter the guess appropriately and check again. Keep doing this until you find a solution.
Mr. Jones has a total of 25 chickens and cows on his farm. How many of each does he have if all together there are 76 feet?
Step 1: Understanding the problem
We are given in the problem that there are 25 chickens and cows.
All together there are 76 feet.
Chickens have 2 feet and cows have 4 feet.
We are trying to determine how many cows and how many chickens Mr. Jones has on his farm.
Step 2: Devise a plan
Going to use Guess and test along with making a tab
Many times the strategy below is used with guess and test.
Make a table and look for a pattern:
Procedure: Make a table reflecting the data in the problem. If done in an orderly way, such a table will often reveal patterns and relationships that suggest how the problem can be solved.
Step 3: Carry out the plan:
| Chickens | Cows | Number of chicken feet | Number of cow feet | Total number of feet |
| 20 | 5 | 40 | 20 | 60 |
| 21 | 4 | 42 | 16 | 58 |
Notice we are going in the wrong direction! The total number of feet is decreasing!
| 19 | 6 | 38 | 24 | 62 |
Better! The total number of feet are increasing!
| 15 | 10 | 30 | 40 | 70 |
| 12 | 13 | 24 | 52 | 76 |
Step 4: Looking back:
Check: 12 + 13 = 25 heads
24 + 52 = 76 feet.
We have found the solution to this problem. I could use this strategy when there are a limited number of possible answers and when two items are the same but they have one characteristic that is different.
Videos to watch:
1. Click on this link to see an example of “Guess and Test”
http://www.mathstories.com/strategies.htm
2. Click on this link to see another example of Guess and Test.
http://www.mathinaction.org/problem-solving-strategies.html
Check in question 1:

Place the digits 8, 10, 11, 12, and 13 in the circles to make the sums across and vertically equal 31. (5 points)
Check in question 2:
Old McDonald has 250 chickens and goats in the barnyard. Altogether there are 760 feet . How many of each animal does he have? Make sure you use Polya’s 4 problem solving steps. (12 points)
Problem Solving Strategy 2 (Draw a Picture). Some problems are obviously about a geometric situation, and it is clear you want to draw a picture and mark down all of the given information before you try to solve it. But even for a problem that is not geometric thinking visually can help!
Videos to watch demonstrating how to use "Draw a Picture".
1. Click on this link to see an example of “Draw a Picture”
2. Click on this link to see another example of Draw a Picture.
Problem Solving Strategy 3 ( Using a variable to find the sum of a sequence.)
Gauss's strategy for sequences.
last term = fixed number ( n -1) + first term
The fix number is the the amount each term is increasing or decreasing by. "n" is the number of terms you have. You can use this formula to find the last term in the sequence or the number of terms you have in a sequence.
Ex: 2, 5, 8, ... Find the 200th term.
Last term = 3(200-1) +2
Last term is 599.
To find the sum of a sequence: sum = [(first term + last term) (number of terms)]/ 2
Sum = (2 + 599) (200) then divide by 2
Sum = 60,100
Check in question 3: (10 points)
Find the 320 th term of 7, 10, 13, 16 …
Then find the sum of the first 320 terms.
Problem Solving Strategy 4 (Working Backwards)
This is considered a strategy in many schools. If you are given an answer, and the steps that were taken to arrive at that answer, you should be able to determine the starting point.
Videos to watch demonstrating of “Working Backwards”
https://www.youtube.com/watch?v=5FFWTsMEeJw
Karen is thinking of a number. If you double it, and subtract 7, you obtain 11. What is Karen’s number?
1. We start with 11 and work backwards.
2. The opposite of subtraction is addition. We will add 7 to 11. We are now at 18.
3. The opposite of doubling something is dividing by 2. 18/2 = 9
4. This should be our answer. Looking back:
9 x 2 = 18 -7 = 11
5. We have the right answer.
Check in question 4:
Christina is thinking of a number.
If you multiply her number by 93, add 6, and divide by 3, you obtain 436. What is her number? Solve this problem by working backwards. (5 points)
Problem Solving Strategy 5 (Looking for a Pattern)
Definition: A sequence is a pattern involving an ordered arrangement of numbers.
We first need to find a pattern.
Ask yourself as you search for a pattern – are the numbers growing steadily larger? Steadily smaller? How is each number related?
Example 1: 1, 4, 7, 10, 13…
Find the next 2 numbers. The pattern is each number is increasing by 3. The next two numbers would be 16 and 19.
Example 2: 1, 4, 9, 16 … find the next 2 numbers. It looks like each successive number is increase by the next odd number. 1 + 3 = 4.
So the next number would be
25 + 11 = 36
Example 3: 10, 7, 4, 1, -2… find the next 2 numbers.
In this sequence, the numbers are decreasing by 3. So the next 2 numbers would be -2 -3 = -5
-5 – 3 = -8
Example 4: 1, 2, 4, 8 … find the next two numbers.
This example is a little bit harder. The numbers are increasing but not by a constant. Maybe a factor?
So each number is being multiplied by 2.
16 x 2 = 32
1. Click on this link to see an example of “Looking for a Pattern”
2. Click on this link to see another example of Looking for a Pattern.
Problem Solving Strategy 6 (Make a List)
Example 1 : Can perfect squares end in a 2 or a 3?
List all the squares of the numbers 1 to 20.
1 4 9 16 25 36 49 64 81 100 121 144 169 196 225 256 289 324 361 400.
Now look at the number in the ones digits. Notice they are 0, 1, 4, 5, 6, or 9. Notice none of the perfect squares end in 2, 3, 7, or 8. This list suggests that perfect squares cannot end in a 2, 3, 7 or 8.
How many different amounts of money can you have in your pocket if you have only three coins including only dimes and quarters?
Quarter’s dimes
0 3 30 cents
1 2 45 cents
2 1 60 cents
3 0 75 cents
Videos demonstrating "Make a List"
Check in question 5:
How many ways can you make change for 23 cents using only pennies, nickels, and dimes? (10 points)
Problem Solving Strategy 7 (Solve a Simpler Problem)
Geometric Sequences:
How would we find the nth term?
Solve a simpler problem:
1, 3, 9, 27.
1. To get from 1 to 3 what did we do?
2. To get from 3 to 9 what did we do?
Let’s set up a table:
Term Number what did we do
Looking back: How would you find the nth term?
Find the 10 th term of the above sequence.
Let L = the tenth term
Problem Solving Strategy 8 (Process of Elimination)
This strategy can be used when there is only one possible solution.
I’m thinking of a number.
The number is odd.
It is more than 1 but less than 100.
It is greater than 20.
It is less than 5 times 7.
The sum of the digits is 7.
It is evenly divisible by 5.
a. We know it is an odd number between 1 and 100.
b. It is greater than 20 but less than 35
21, 23, 25, 27, 29, 31, 33, 35. These are the possibilities.
c. The sum of the digits is 7
21 (2+1=3) No 23 (2+3 = 5) No 25 (2 + 5= 7) Yes Using the same process we see there are no other numbers that meet this criteria. Also we notice 25 is divisible by 5. By using the strategy elimination, we have found our answer.
Check in question 6: (8 points)
Jose is thinking of a number.
The number is not odd.
The sum of the digits is divisible by 2.
The number is a multiple of 11.
It is greater than 5 times 4.
It is a multiple of 6
It is less than 7 times 8 +23
What is the number?
Click on this link for a quick review of the problem solving strategies.
https://garyhall.org.uk/maths-problem-solving-strategies.html
High Impact Tutoring Built By Math Experts
Personalized standards-aligned one-on-one math tutoring for schools and districts
Free ready-to-use math resources
Hundreds of free math resources created by experienced math teachers to save time, build engagement and accelerate growth

20 Effective Math Strategies To Approach Problem-Solving
Katie Keeton
Math strategies for problem-solving help students use a range of approaches to solve many different types of problems. It involves identifying the problem and carrying out a plan of action to find the answer to mathematical problems.
Problem-solving skills are essential to math in the general classroom and real-life. They require logical reasoning and critical thinking skills. Students must be equipped with strategies to help them find solutions to problems.
This article explores mathematical problem solving strategies, logical reasoning and critical thinking skills to help learners with solving math word problems independently in real-life situations.
What are problem-solving strategies?
Problem-solving strategies in math are methods students can use to figure out solutions to math problems. Some problem-solving strategies:
- Draw a model
- Use different approaches
- Check the inverse to make sure the answer is correct
Students need to have a toolkit of math problem-solving strategies at their disposal to provide different ways to approach math problems. This makes it easier to find solutions and understand math better.
Strategies can help guide students to the solution when it is difficult ot know when to start.

The ultimate guide to problem solving techniques
Download these ready-to-go problem solving techniques that every student should know. Includes printable tasks for students including challenges, short explanations for teachers with questioning prompts.
20 Math Strategies For Problem-Solving
Different problem-solving math strategies are required for different parts of the problem. It is unlikely that students will use the same strategy to understand and solve the problem.
Here are 20 strategies to help students develop their problem-solving skills.
Strategies to understand the problem
Strategies that help students understand the problem before solving it helps ensure they understand:
- The context
- What the key information is
- How to form a plan to solve it
Following these steps leads students to the correct solution and makes the math word problem easier .
Here are five strategies to help students understand the content of the problem and identify key information.
1. Read the problem aloud
Read a word problem aloud to help understand it. Hearing the words engages auditory processing. This can make it easier to process and comprehend the context of the situation.
2. Highlight keywords
When keywords are highlighted in a word problem, it helps the student focus on the essential information needed to solve it. Some important keywords help determine which operation is needed. For example, if the word problem asks how many are left, the problem likely requires subtraction. Ensure students highlight the keywords carefully and do not highlight every number or keyword. There is likely irrelevant information in the word problem.
3. Summarize the information
Read the problem aloud, highlight the key information and then summarize the information. Students can do this in their heads or write down a quick summary. Summaries should include only the important information and be in simple terms that help contextualize the problem.
4. Determine the unknown
A common problem that students have when solving a word problem is misunderstanding what they are solving. Determine what the unknown information is before finding the answer. Often, a word problem contains a question where you can find the unknown information you need to solve. For example, in the question ‘How many apples are left?’ students need to find the number of apples left over.
5. Make a plan
Once students understand the context of the word problem, have dentified the important information and determined the unknown, they can make a plan to solve it. The plan will depend on the type of problem. Some problems involve more than one step to solve them as some require more than one answer. Encourage students to make a list of each step they need to take to solve the problem before getting started.
Strategies for solving the problem
1. draw a model or diagram.
Students may find it useful to draw a model, picture, diagram, or other visual aid to help with the problem solving process. It can help to visualize the problem to understand the relationships between the numbers in the problem. In turn, this helps students see the solution.
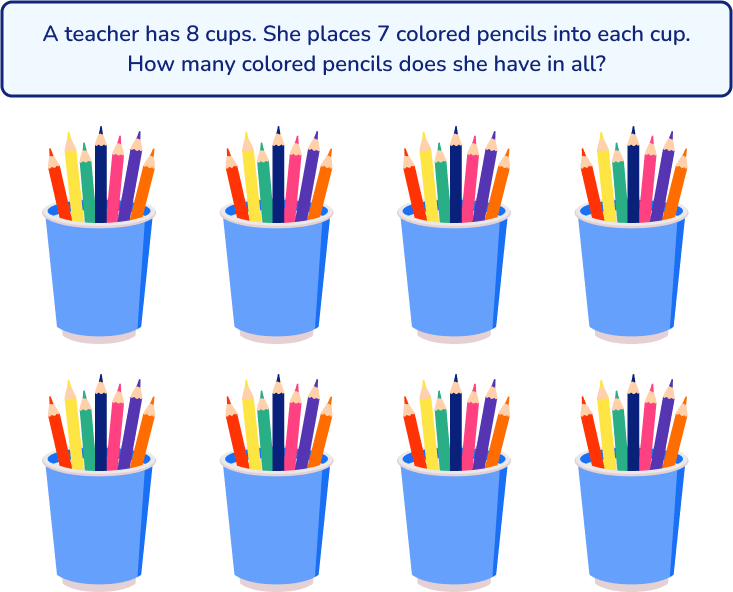
Similarly, you could draw a model to represent the objects in the problem:
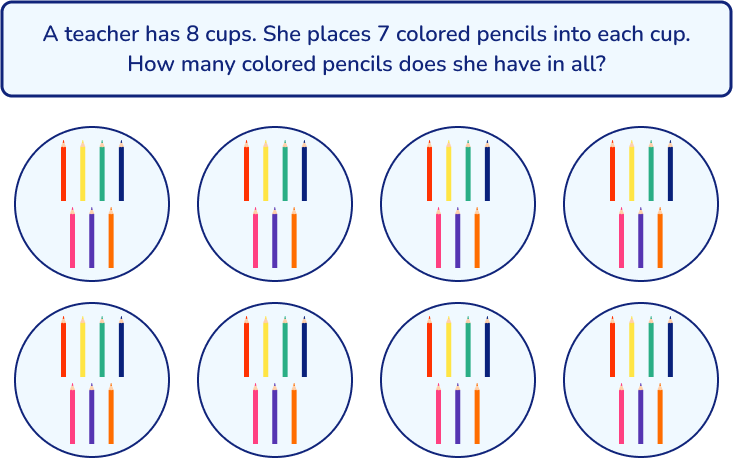
2. Act it out
This particular strategy is applicable at any grade level but is especially helpful in math investigation in elementary school . It involves a physical demonstration or students acting out the problem using movements, concrete resources and math manipulatives . When students act out a problem, they can visualize and contectualize the word problem in another way and secure an understanding of the math concepts. The examples below show how 1st-grade students could “act out” an addition and subtraction problem:
| The problem | How to act out the problem |
| Gia has 6 apples. Jordan has 3 apples. How many apples do they have altogether? | Two students use counters to represent the apples. One student has 6 counters and the other student takes 3. Then, they can combine their “apples” and count the total. |
| Michael has 7 pencils. He gives 2 pencils to Sarah. How many pencils does Michael have now? | One student (“Michael”) holds 7 pencils, the other (“Sarah”) holds 2 pencils. The student playing Michael gives 2 pencils to the student playing Sarah. Then the students count how many pencils Michael is left holding. |
3. Work backwards
Working backwards is a popular problem-solving strategy. It involves starting with a possible solution and deciding what steps to take to arrive at that solution. This strategy can be particularly helpful when students solve math word problems involving multiple steps. They can start at the end and think carefully about each step taken as opposed to jumping to the end of the problem and missing steps in between.
For example,

To solve this problem working backwards, start with the final condition, which is Sam’s grandmother’s age (71) and work backwards to find Sam’s age. Subtract 20 from the grandmother’s age, which is 71. Then, divide the result by 3 to get Sam’s age. 71 – 20 = 51 51 ÷ 3 = 17 Sam is 17 years old.
4. Write a number sentence
When faced with a word problem, encourage students to write a number sentence based on the information. This helps translate the information in the word problem into a math equation or expression, which is more easily solved. It is important to fully understand the context of the word problem and what students need to solve before writing an equation to represent it.
5. Use a formula
Specific formulas help solve many math problems. For example, if a problem asks students to find the area of a rug, they would use the area formula (area = length × width) to solve. Make sure students know the important mathematical formulas they will need in tests and real-life. It can help to display these around the classroom or, for those who need more support, on students’ desks.
Strategies for checking the solution
Once the problem is solved using an appropriate strategy, it is equally important to check the solution to ensure it is correct and makes sense.
There are many strategies to check the solution. The strategy for a specific problem is dependent on the problem type and math content involved.
Here are five strategies to help students check their solutions.
1. Use the Inverse Operation
For simpler problems, a quick and easy problem solving strategy is to use the inverse operation. For example, if the operation to solve a word problem is 56 ÷ 8 = 7 students can check the answer is correct by multiplying 8 × 7. As good practice, encourage students to use the inverse operation routinely to check their work.
2. Estimate to check for reasonableness
Once students reach an answer, they can use estimation or rounding to see if the answer is reasonable. Round each number in the equation to a number that’s close and easy to work with, usually a multiple of ten. For example, if the question was 216 ÷ 18 and the quotient was 12, students might round 216 to 200 and round 18 to 20. Then use mental math to solve 200 ÷ 20, which is 10. When the estimate is clear the two numbers are close. This means your answer is reasonable.
3. Plug-In Method
This method is particularly useful for algebraic equations. Specifically when working with variables. To use the plug-in method, students solve the problem as asked and arrive at an answer. They can then plug the answer into the original equation to see if it works. If it does, the answer is correct.

If students use the equation 20m+80=300 to solve this problem and find that m = 11, they can plug that value back into the equation to see if it is correct. 20m + 80 = 300 20 (11) + 80 = 300 220 + 80 = 300 300 = 300 ✓
4. Peer Review
Peer review is a great tool to use at any grade level as it promotes critical thinking and collaboration between students. The reviewers can look at the problem from a different view as they check to see if the problem was solved correctly. Problem solvers receive immediate feedback and the opportunity to discuss their thinking with their peers. This strategy is effective with mixed-ability partners or similar-ability partners. In mixed-ability groups, the partner with stronger skills provides guidance and support to the partner with weaker skills, while reinforcing their own understanding of the content and communication skills. If partners have comparable ability levels and problem-solving skills, they may find that they approach problems differently or have unique insights to offer each other about the problem-solving process.
5. Use a Calculator
A calculator can be introduced at any grade level but may be best for older students who already have a foundational understanding of basic math operations. Provide students with a calculator to allow them to check their solutions independently, accurately, and quickly. Since calculators are so readily available on smartphones and tablets, they allow students to develop practical skills that apply to real-world situations.
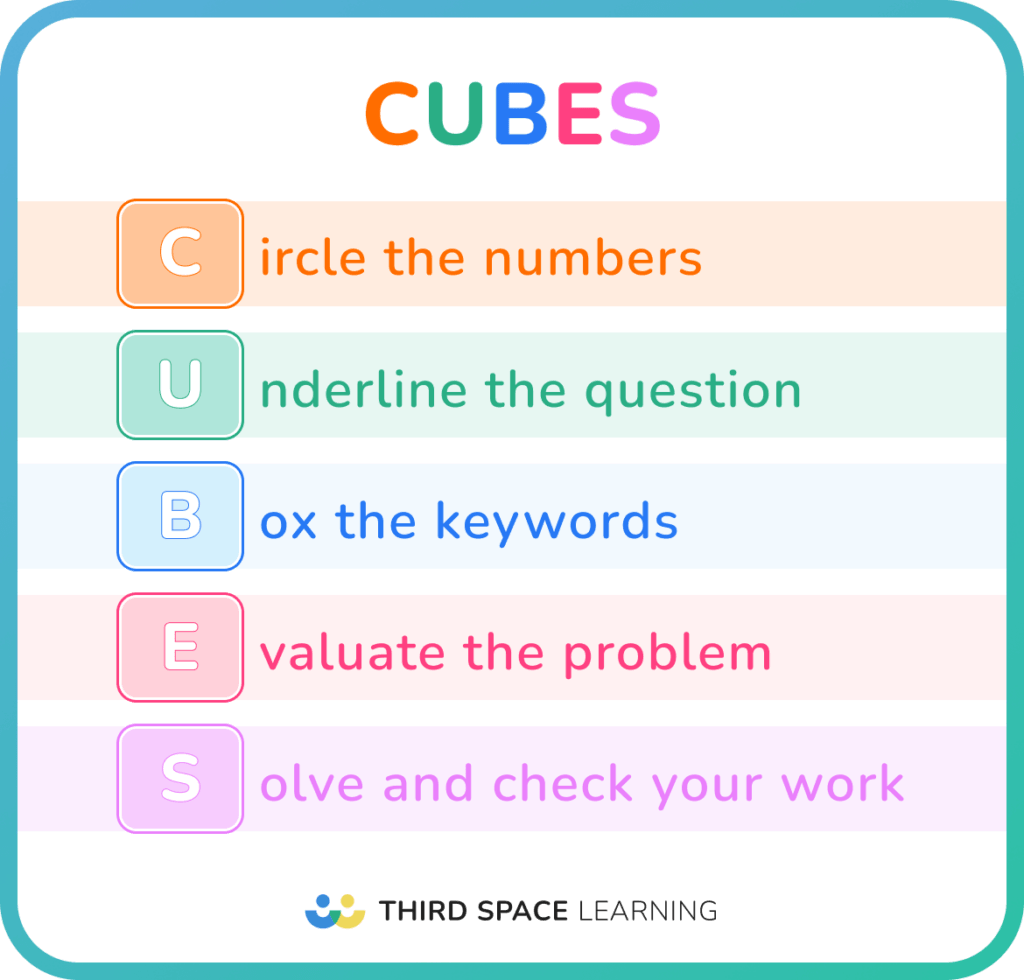
Step-by-step problem-solving processes for your classroom
In his book, How to Solve It , published in 1945, mathematician George Polya introduced a 4-step process to solve problems.
Polya’s 4 steps include:
- Understand the problem
- Devise a plan
- Carry out the plan
Today, in the style of George Polya, many problem-solving strategies use various acronyms and steps to help students recall.
Many teachers create posters and anchor charts of their chosen process to display in their classrooms. They can be implemented in any elementary, middle school or high school classroom.
Here are 5 problem-solving strategies to introduce to students and use in the classroom.
How Third Space Learning improves problem-solving
Resources .
Third Space Learning offers a free resource library is filled with hundreds of high-quality resources. A team of experienced math experts carefully created each resource to develop students mental arithmetic, problem solving and critical thinking.
Explore the range of problem solving resources for 2nd to 8th grade students.
One-on-one tutoring
Third Space Learning offers one-on-one math tutoring to help students improve their math skills. Highly qualified tutors deliver high-quality lessons aligned to state standards.
Former teachers and math experts write all of Third Space Learning’s tutoring lessons. Expertly designed lessons follow a “my turn, follow me, your turn” pedagogy to help students move from guided instruction and problem-solving to independent practice.
Throughout each lesson, tutors ask higher-level thinking questions to promote critical thinking and ensure students are developing a deep understanding of the content and problem-solving skills.
Problem-solving
Educators can use many different strategies to teach problem-solving and help students develop and carry out a plan when solving math problems. Incorporate these math strategies into any math program and use them with a variety of math concepts, from whole numbers and fractions to algebra.
Teaching students how to choose and implement problem-solving strategies helps them develop mathematical reasoning skills and critical thinking they can apply to real-life problem-solving.
READ MORE : 8 Common Core math examples
There are many different strategies for problem-solving; Here are 5 problem-solving strategies: • draw a model • act it out • work backwards • write a number sentence • use a formula
Here are 10 strategies of problem-solving: • Read the problem aloud • Highlight keywords • Summarize the information • Determine the unknown • Make a plan • Draw a model • Act it out • Work backwards • Write a number sentence • Use a formula
1. Understand the problem 2. Devise a plan 3. Carry out the plan 4. Look back
Some strategies you can use to solve challenging math problems are: breaking the problem into smaller parts, using diagrams or models, applying logical reasoning, and trying different approaches.
Related articles

Why Student Centered Learning Is Important: A Guide For Educators

13 Effective Learning Strategies: A Guide to Using them in your Math Classroom

Differentiated Instruction: 9 Differentiated Curriculum And Instruction Strategies For Teachers

5 Math Mastery Strategies To Incorporate Into Your 4th and 5th Grade Classrooms
Ultimate Guide to Metacognition [FREE]
Looking for a summary on metacognition in relation to math teaching and learning?
Check out this guide featuring practical examples, tips and strategies to successfully embed metacognition across your school to accelerate math growth.
Privacy Overview
Want to create or adapt books like this? Learn more about how Pressbooks supports open publishing practices.
5 Teaching Mathematics Through Problem Solving
Janet Stramel

In his book “How to Solve It,” George Pólya (1945) said, “One of the most important tasks of the teacher is to help his students. This task is not quite easy; it demands time, practice, devotion, and sound principles. The student should acquire as much experience of independent work as possible. But if he is left alone with his problem without any help, he may make no progress at all. If the teacher helps too much, nothing is left to the student. The teacher should help, but not too much and not too little, so that the student shall have a reasonable share of the work.” (page 1)
What is a problem in mathematics? A problem is “any task or activity for which the students have no prescribed or memorized rules or methods, nor is there a perception by students that there is a specific ‘correct’ solution method” (Hiebert, et. al., 1997). Problem solving in mathematics is one of the most important topics to teach; learning to problem solve helps students develop a sense of solving real-life problems and apply mathematics to real world situations. It is also used for a deeper understanding of mathematical concepts. Learning “math facts” is not enough; students must also learn how to use these facts to develop their thinking skills.
According to NCTM (2010), the term “problem solving” refers to mathematical tasks that have the potential to provide intellectual challenges for enhancing students’ mathematical understanding and development. When you first hear “problem solving,” what do you think about? Story problems or word problems? Story problems may be limited to and not “problematic” enough. For example, you may ask students to find the area of a rectangle, given the length and width. This type of problem is an exercise in computation and can be completed mindlessly without understanding the concept of area. Worthwhile problems includes problems that are truly problematic and have the potential to provide contexts for students’ mathematical development.
There are three ways to solve problems: teaching for problem solving, teaching about problem solving, and teaching through problem solving.
Teaching for problem solving begins with learning a skill. For example, students are learning how to multiply a two-digit number by a one-digit number, and the story problems you select are multiplication problems. Be sure when you are teaching for problem solving, you select or develop tasks that can promote the development of mathematical understanding.
Teaching about problem solving begins with suggested strategies to solve a problem. For example, “draw a picture,” “make a table,” etc. You may see posters in teachers’ classrooms of the “Problem Solving Method” such as: 1) Read the problem, 2) Devise a plan, 3) Solve the problem, and 4) Check your work. There is little or no evidence that students’ problem-solving abilities are improved when teaching about problem solving. Students will see a word problem as a separate endeavor and focus on the steps to follow rather than the mathematics. In addition, students will tend to use trial and error instead of focusing on sense making.
Teaching through problem solving focuses students’ attention on ideas and sense making and develops mathematical practices. Teaching through problem solving also develops a student’s confidence and builds on their strengths. It allows for collaboration among students and engages students in their own learning.
Consider the following worthwhile-problem criteria developed by Lappan and Phillips (1998):
- The problem has important, useful mathematics embedded in it.
- The problem requires high-level thinking and problem solving.
- The problem contributes to the conceptual development of students.
- The problem creates an opportunity for the teacher to assess what his or her students are learning and where they are experiencing difficulty.
- The problem can be approached by students in multiple ways using different solution strategies.
- The problem has various solutions or allows different decisions or positions to be taken and defended.
- The problem encourages student engagement and discourse.
- The problem connects to other important mathematical ideas.
- The problem promotes the skillful use of mathematics.
- The problem provides an opportunity to practice important skills.
Of course, not every problem will include all of the above. Sometimes, you will choose a problem because your students need an opportunity to practice a certain skill.
Key features of a good mathematics problem includes:
- It must begin where the students are mathematically.
- The feature of the problem must be the mathematics that students are to learn.
- It must require justifications and explanations for both answers and methods of solving.

Problem solving is not a neat and orderly process. Think about needlework. On the front side, it is neat and perfect and pretty.

But look at the b ack.
It is messy and full of knots and loops. Problem solving in mathematics is also like this and we need to help our students be “messy” with problem solving; they need to go through those knots and loops and learn how to solve problems with the teacher’s guidance.
When you teach through problem solving , your students are focused on ideas and sense-making and they develop confidence in mathematics!
Mathematics Tasks and Activities that Promote Teaching through Problem Solving

Choosing the Right Task
Selecting activities and/or tasks is the most significant decision teachers make that will affect students’ learning. Consider the following questions:
- Teachers must do the activity first. What is problematic about the activity? What will you need to do BEFORE the activity and AFTER the activity? Additionally, think how your students would do the activity.
- What mathematical ideas will the activity develop? Are there connections to other related mathematics topics, or other content areas?
- Can the activity accomplish your learning objective/goals?

Low Floor High Ceiling Tasks
By definition, a “ low floor/high ceiling task ” is a mathematical activity where everyone in the group can begin and then work on at their own level of engagement. Low Floor High Ceiling Tasks are activities that everyone can begin and work on based on their own level, and have many possibilities for students to do more challenging mathematics. One gauge of knowing whether an activity is a Low Floor High Ceiling Task is when the work on the problems becomes more important than the answer itself, and leads to rich mathematical discourse [Hover: ways of representing, thinking, talking, agreeing, and disagreeing; the way ideas are exchanged and what the ideas entail; and as being shaped by the tasks in which students engage as well as by the nature of the learning environment].
The strengths of using Low Floor High Ceiling Tasks:
- Allows students to show what they can do, not what they can’t.
- Provides differentiation to all students.
- Promotes a positive classroom environment.
- Advances a growth mindset in students
- Aligns with the Standards for Mathematical Practice
Examples of some Low Floor High Ceiling Tasks can be found at the following sites:
- YouCubed – under grades choose Low Floor High Ceiling
- NRICH Creating a Low Threshold High Ceiling Classroom
- Inside Mathematics Problems of the Month
Math in 3-Acts
Math in 3-Acts was developed by Dan Meyer to spark an interest in and engage students in thought-provoking mathematical inquiry. Math in 3-Acts is a whole-group mathematics task consisting of three distinct parts:
Act One is about noticing and wondering. The teacher shares with students an image, video, or other situation that is engaging and perplexing. Students then generate questions about the situation.
In Act Two , the teacher offers some information for the students to use as they find the solutions to the problem.
Act Three is the “reveal.” Students share their thinking as well as their solutions.
“Math in 3 Acts” is a fun way to engage your students, there is a low entry point that gives students confidence, there are multiple paths to a solution, and it encourages students to work in groups to solve the problem. Some examples of Math in 3-Acts can be found at the following websites:
- Dan Meyer’s Three-Act Math Tasks
- Graham Fletcher3-Act Tasks ]
- Math in 3-Acts: Real World Math Problems to Make Math Contextual, Visual and Concrete
Number Talks
Number talks are brief, 5-15 minute discussions that focus on student solutions for a mental math computation problem. Students share their different mental math processes aloud while the teacher records their thinking visually on a chart or board. In addition, students learn from each other’s strategies as they question, critique, or build on the strategies that are shared.. To use a “number talk,” you would include the following steps:
- The teacher presents a problem for students to solve mentally.
- Provide adequate “ wait time .”
- The teacher calls on a students and asks, “What were you thinking?” and “Explain your thinking.”
- For each student who volunteers to share their strategy, write their thinking on the board. Make sure to accurately record their thinking; do not correct their responses.
- Invite students to question each other about their strategies, compare and contrast the strategies, and ask for clarification about strategies that are confusing.
“Number Talks” can be used as an introduction, a warm up to a lesson, or an extension. Some examples of Number Talks can be found at the following websites:
- Inside Mathematics Number Talks
- Number Talks Build Numerical Reasoning

Saying “This is Easy”
“This is easy.” Three little words that can have a big impact on students. What may be “easy” for one person, may be more “difficult” for someone else. And saying “this is easy” defeats the purpose of a growth mindset classroom, where students are comfortable making mistakes.
When the teacher says, “this is easy,” students may think,
- “Everyone else understands and I don’t. I can’t do this!”
- Students may just give up and surrender the mathematics to their classmates.
- Students may shut down.
Instead, you and your students could say the following:
- “I think I can do this.”
- “I have an idea I want to try.”
- “I’ve seen this kind of problem before.”
Tracy Zager wrote a short article, “This is easy”: The Little Phrase That Causes Big Problems” that can give you more information. Read Tracy Zager’s article here.
Using “Worksheets”
Do you want your students to memorize concepts, or do you want them to understand and apply the mathematics for different situations?
What is a “worksheet” in mathematics? It is a paper and pencil assignment when no other materials are used. A worksheet does not allow your students to use hands-on materials/manipulatives [Hover: physical objects that are used as teaching tools to engage students in the hands-on learning of mathematics]; and worksheets are many times “naked number” with no context. And a worksheet should not be used to enhance a hands-on activity.
Students need time to explore and manipulate materials in order to learn the mathematics concept. Worksheets are just a test of rote memory. Students need to develop those higher-order thinking skills, and worksheets will not allow them to do that.
One productive belief from the NCTM publication, Principles to Action (2014), states, “Students at all grade levels can benefit from the use of physical and virtual manipulative materials to provide visual models of a range of mathematical ideas.”
You may need an “activity sheet,” a “graphic organizer,” etc. as you plan your mathematics activities/lessons, but be sure to include hands-on manipulatives. Using manipulatives can
- Provide your students a bridge between the concrete and abstract
- Serve as models that support students’ thinking
- Provide another representation
- Support student engagement
- Give students ownership of their own learning.
Adapted from “ The Top 5 Reasons for Using Manipulatives in the Classroom ”.
any task or activity for which the students have no prescribed or memorized rules or methods, nor is there a perception by students that there is a specific ‘correct’ solution method
should be intriguing and contain a level of challenge that invites speculation and hard work, and directs students to investigate important mathematical ideas and ways of thinking toward the learning
involves teaching a skill so that a student can later solve a story problem
when we teach students how to problem solve
teaching mathematics content through real contexts, problems, situations, and models
a mathematical activity where everyone in the group can begin and then work on at their own level of engagement
20 seconds to 2 minutes for students to make sense of questions
Mathematics Methods for Early Childhood Copyright © 2021 by Janet Stramel is licensed under a Creative Commons Attribution 4.0 International License , except where otherwise noted.
Share This Book
Problem solving in mathematics education: tracing its foundations and current research-practice trends
- Original Paper
- Open access
- Published: 30 April 2024
Cite this article
You have full access to this open access article

- Manuel Santos-Trigo ORCID: orcid.org/0000-0002-7144-2098 1
937 Accesses
1 Altmetric
Explore all metrics
In tracing recent research trends and directions in mathematical problem-solving, it is argued that advances in mathematics practices occur and take place around two intertwined activities, mathematics problem formulation and ways to approach and solve those problems. In this context, a problematizing principle emerges as central activity to organize mathematics curriculum proposals and ways to structure problem-solving learning environments. Subjects’ use of concrete, abstract, symbolic, or digital tools not only influences the ways to pose and pursue mathematical problems; but also shapes the type of representation, exploration, and reasoning they engage to work and solve problems. Problem-solving foundations that privilege learners’ development of habits of mathematical practices that involve an inquiry method to formulate conjectures, to look for different ways to represent and approach problems, and to support and communicate results shed light on directions of current research trends and the relevance of rethinking curriculum proposals and extending problem-solving environments in terms of teachers/students’ consistent use of digital tools and online developments.
Similar content being viewed by others

Word problems in mathematics education: a survey
Research on early childhood mathematics teaching and learning.
A systematic review of TPACK research in primary mathematics education
Avoid common mistakes on your manuscript.
1 Introduction and rationale
Mathematical problem solving has been a prominent theme and research area in the mathematics education agenda during the last four decades. Problem-solving perspectives have influenced and shaped mathematics curriculum proposals and ways to support learning environments worldwide (Törner et al., 2007 ; Toh et al., 2023 ). Various disciplinary communities have identified and contributed to connect problem-solving approaches with the students’ learning, construction, and application of mathematical knowledge. The mathematics community recognizes that the formulation and resolution of problems are central activities in the development of the discipline (Halmos, 1980 , Polya, 1945 ). Indeed, the identification and presentation of lists of unsolved mathematical problems have been a tradition that has inspired the mathematics community to approach mathematical problems and to generate mathematical knowledge (Hilbert, 1902 ; Devlin, 2002 ). Thus, mathematical problems, results, and solution attempts provide information regarding what areas and contents were studied at different times during the development of the discipline (Santos-Trigo, 2020a , b ). Cai et al. ( 2023 ) stated that “ …[E]ngaging learners in the activity of problem posing reflects a potentially strong link to the discipline of mathematics” (p. 5). Thurston ( 1994 ) recognized that understanding and applying a mathematical concept implies analysing, coordinating, and integrating diverse meanings (geometric, visual, intuitive, and formal definition) associated with such concept and ways to carry out corresponding procedures and operations in problematic situations.
The centrality of problem-solving in mathematicians’ own work and in their teaching, is incontrovertible. Problem-solving is also a central topic for mathematics educators, who have developed conceptual frameworks to formulate general ideas about problem-solving (as opposed to the specific ideas needed for solving specific problems) (Fried, 2014 ; p.17).
That is, the mathematics education community is interested in analysing and documenting the students’ cognitive and social behaviours to understand and develop mathematical knowledge and problem-solving competencies. “…the idea of understanding how mathematicians treat and solve problems, and then implementing this understanding in instruction design, was pivotal in mathematics education research and practice” (Koichu, 2014 ). In addition, other disciplines such as psychology, cognitive science or artificial intelligence have provided tools and methods to delve into learners’ ways to understand mathematical concepts and to work on problem situations. Thus, members of various communities have often worked in collaboration to identify and relate relevant aspects of mathematical practices with the design and implementation of learning scenarios that foster and enhance students’ mathematical thinking and the development of problem-solving competencies.
2 Methods and procedures
Research focus, themes, and inquiry methods in the mathematical problem-solving agenda have varied and been influenced and shaped by theoretical and methodological developments of mathematics education as a discipline (English & Kirshner, 2016 ; Liljedahl & Cai, 2021 ). Further, research designs and methods used in cognitive, social, and computational fields have influenced the ways in which mathematical problem-solving research are framed. An overarching question to capture shifts and foundations in problem-solving developments was: How has mathematical problem-solving research agenda varied and evolved in terms of ways to frame, pose, and pursue research questions? In addressing this question, it was important to identify and contrast the structure and organization around some published problem-solving reviews (Lester, 1994 ; Törner et al., 2007 ; Rott et al., 2021 ; Liljedahl & Cai, 2021 ; Toh et al., 2023 ) to shed light on a possible route to connect seminal developments in the field with current research trends and perspectives in mathematical problem-solving developments. The goal was to identify common problem-solving principles that have provided a rational and foundations to support recent problem-solving approaches for learners to construct mathematical knowledge and to develop problem-solving competencies. The criteria to select the set of published peer-reviewed studies, to consider in this review, involved choosing articles published in indexed journals (ZDM-Mathematics Education, Educational Studies in Mathematics, Mathematical Thinking and Learning, Journal of Mathematical Behavior, and Journal for Research in Mathematics Education); contributions that appear in International Handbooks in Mathematics Education; and chapters published in recent mathematical problem-solving books. The initial search included 205 publications whose number was reduced to 55, all published in English, based on reviewing their abstracts and conclusions. Around 100 of the initial selection appeared in the references of an ongoing weekly mathematical problem-solving doctoral seminar that has been implemented during the last six years in our department. In addition, some well-known authors in the field were asked to identify their most representative publications to include in the review list. Here, some suggestions were received, but at the end the list of contributions, that appears in the references section, was chosen based on my vision and experience in the field. The goal was to identify main issues or dimensions to frame and analyse recent research trends and perspectives in mathematical problem-solving developments. Thus, seminal reviews in the field (Schoenfeld, 1992 ; Lester, 1994 ; Törner et al., 2007 ) provided directions on ways to structure and select the questions used to analyse the selected contributions. Table 1 shows chosen issues that resemble features of an adjusted framework that Lester ( 1994 ) proposed to organize, summarize, and analyse problem-solving developments in terms of research emphasis (themes and research questions), methodologies (research designs and methods), and achieved results that the problem-solving community addressed during the 1970–1994 period. Furthermore, relevant shifts in the mathematical problem-solving agenda could be identified and explained in terms of what the global mathematics education and other disciplines pursue at different periods.
It is important to mention that the content and structure of this paper involve a narrative synthesis of selected articles that includes contributions related to mathematical problem-solving foundations and those that address recent developments published in the last 9 years that involve the use of digital technologies. Table 1 shows themes, issues, and overarching questions that were used to delve into problem-solving developments.
To contextualize the current state of art in the field, it is important to revisit problem-solving principles and tenets that provide foundations and a rationale to centre and support the design and implementation of learning environments around problem-solving activities (Santos-Trigo, 2020a , b ). The identification of mathematical problem-solving foundations also implies acknowledging what terms, concepts, and language or discourse that the problem-solving community has used to refer to and frame problem-solving approaches. For example, routine and nonroutine tasks, heuristic and metacognitive strategies, students’ beliefs, mathematical thinking and practices, resources, orientations, etc. are common terms used to explain, foster, and characterize students’ problem-solving behaviours and performances. Recently, the consistent use of digital technologies in educational tasks has extended the problem-solving language to include terms such as subjects’ tool appropriation, dynamic models, dragging or moving orderly objects, tracing loci, visual or empirical solution, ChatGPT prompts, etc.
3 On mathematical problem-solving foundations and the problematizing principle
There might be different ways to interpret and implement a problem-solving approach for students to understand concepts and to solve problems (Törner, Schoenfeld, & Reiss, 2007 ; Toh et al., 2023 ); nevertheless, there are common principles or tenets that distinguish and support a problem-solving teaching/learning environment. A salient feature in any problem-solving approach to learn mathematics is a conceptualization of the discipline that privileges and enhance the students’ development of mathematical practices or reasoning habits of mathematical thinking (Cuoco, et, al., 1996 ; Dick & Hollebrands, 2011 ; Schoenfeld, 2022 ). In this context, students need to conceptualize and think of their own learning as a set of dilemmas that are represented, explored, and solved in terms of mathematical resources and strategies (Santos-Trigo, 2023 ; Hiebert et al., 1996 ).
Furthermore, students’ problem-solving experiences and behaviours reflect and become a way of thinking that is consistent with mathematics practices and is manifested in terms of the activities they engage throughout all problem-solving phases. Thus, they privilege the development of mathematics habits such as to always look for different ways to model and explore mathematical problems, to formulate conjectures, and to search for arguments to support them, share problem solutions, defend their ideas, and to develop a proper language to communicate results. In terms of connecting ways of developing mathematical knowledge and the design of learning environments to develop mathematical thinking and problem-solving competencies, Polya ( 1945 ) identifies an inquiry approach for students to understand, make sense, and apply mathematical concepts. He illustrated the importance for students to pose and pursue different questions around four intertwined problem-solving phases: Understanding and making sense of the problem statement (what is the problem about? What data are provided? What is asked to find? etc.), the design of a solution plan (how the problem can be approached? ), the implementation of such plan (how the plan can be achieved? ), and the looking-back phase that involves reviewing the solution process (data used, checking the involved operations, consistency of units, and partial and global solution), generalizing the solution methods and posing new problems. Indeed, the looking-back phase involves the formulation of new or related problems (Toh et al., 2023 ). “For Pólya, mathematics was about inquiry; it was about sense making; it was about understanding how and why mathematical ideas fit together the ways they do” (cited in Schoenfeld, 2020 , p. 1167).
Likewise, the Nobel laureate I. I. Rabi mentioned that, when he came home from school, “while other mothers asked their kids ‘ Did you learn anything today ?’ [my mother] would say, ‘ Izzy, did you ask a good question today ?’” (Berger, 2014 , p.67).
Thus, the problematizing principle is key for students to engage in mathematical problem-solving activities, and it gets activated by an inquiry or inquisitive method that is expressed in terms of questions that students pose and pursue to delve into concepts meaning, representations, explorations, operations, and to work on mathematical tasks (Santos-Trigo, 2020a , b ).
4 The importance of mathematical tasks and the role of tools in problem-solving perspectives
In a problem-solving approach, learners develop a way of thinking to work on different types of tasks that involve a variety of context and aims (Cai & Hwang, 2023 ). A task might require students to formulate a problem from given information, to estimate how much water a family spend in one year, to prove a geometry theorem, to model genetic sequences or to understand the interplay between climate and geography. In this process, students identify mathematical resources, concepts, and strategies to model and explore partial and global solutions, and ways to extend solution methods and results. Furthermore, mathematical tasks or problems are essential for students to engage in mathematical practice and to develop problem-solving competencies. Task statements should be situated in different contexts including realistic, authentic, or mathematical domains, and prompts or questions to solve or respond or even provide information or data for students to formulate and solve their own problems (problem posing). Current events or problematic situations such as climate change, immigration, or pandemics not only are part of individuals concerns; but also, a challenge for teachers and students to model and analyze those complex problems through mathematics and others disciplines knowledge (English, 2023 ). Santos-Trigo ( 2019 ) proposed a framework to transform exercises or routine textbook problems into a series of nonroutine tasks in which students have an opportunity to dynamically model, explore, and extend, the initial problem. Here, the use of technology becomes important to explore the behavior of some elements within the model to find objects’ mathematical relationships. That is, students work on tasks in such a way that even routine problems become a starting point for them to engage in mathematical reflection to extend the initial nature of the task (Santos-Trigo & Reyes-Martínez, 2019 ). Recently, the emergence of tools such as the ChatGPT has confirmed the importance for learners to problematize situations, including complex problems, in terms of providing prompts or inputs that the tool processes and answers. Here, students analyze the tool’ responses and assess its pertinence to work and solve the task. Indeed, a way to use ChatGPT involves that students understand or make sense of the problem statement and pose questions (inputs or prompts) to ask the tool for concept information or ways to approach or solve the task. Then, students analyze the relevance, viability, and consistency of the tool’s answer and introduce new inputs to continue with the solution process or to look for another way to approach the task. Based on the ChatGPT output or task solution, students could always ask whether the tool can provide other ways to solve the task.
5 Main problem-solving research themes and results
In this section the focus will be on identifying certain problem-solving developments that have permeated recent directions of the field. One relates to the importance of extending research designs to analyse and characterize learners’ problem-solving process to work on different types of tasks. Another development involves ways in which theoretical advances in mathematics education have shaped the mathematical problem-solving research agenda and the extent to which regional or national educational systems or traditions influence the developments of conceptual frameworks in the field and ways to implement problem-solving activities within the corresponding system. Finally, research results in the field have provided directions to design and implement curriculum proposals around the world and these proposals have evolved in terms of both content structure and classroom dynamics including the use of digital technologies. Santos-Trigo ( 2023 ) stated that the teachers and students’ systematic use of digital technologies not only expands their ways of reasoning and solving mathematical problems; but also opens new research areas that aim to analyse the integration of several digital tools in curriculum proposals and learning scenarios. The focus of this review will be on presenting problem-solving directions and results in the last 9 years; however, it became relevant to identify and review what principles and tenets provided bases or foundations to support and define current research trends and directions in the field. That is, accumulated research that has contributed to advance and expand the problem-solving research agenda included shifts in the tools used to delve into learners’ problem approaches, the development of conceptual frameworks to explain and characterize students’ mathematical thinking, the tools used to work on mathematical tasks (from paper and pencil, ruler and compass or semiotic tools to digital apps), and in the design of curriculum proposals and the implementation of problem-solving learning scenarios.
5.1 Relevant shifts in problem-solving developments and results
Questions used to analyse important developments in the field include: What research designs and tools are used to foster and analyse learners’ problem-solving performances? How have conceptual frameworks evolved to pose and frame research questions in the field? How have accumulated research results in the field been used to support curriculum proposals and their implementation?
5.1.1 Methodological and research paradigms
Research designs in problem-solving studies have gradually moved from quantitative or statistical paradigms to qualitative perspectives that involve data collection from different sources such as task-based interviews, fieldnotes from observations, students’ written reports, etc. to analyse students’ problem-solving approaches and performances. Trustworthiness of results included triangulating and interpreting data sources from students’ videotapes transcriptions, outside observer notes, class observations, etc. (Stake, 2000 ). Hence, the work of Krutestkii ( 1976 ) was seminal in providing tools to delve into the students’ thinking while solving mathematical tasks. His research program aimed to study the nature and structure of children’ mathematical abilities. His methodological approach involved the use of student’s task-based interviews, teachers, and mathematicians’ questionaries to explore the nature of mathematical abilities, the analysis of eminent mathematicians and physicists regarding their nature and emergence of their talents and case studies of gifted children in mathematics. A major contribution of his research was the variety of mathematical tasks used to explore and analyse the mathematical abilities of school children. Recently, the mathematical problem-posing agenda has been revisited to advance conceptual frameworks to enhance the students’ formulation of problems to learn concepts and to develop problem-solving competencies (Cai et al., 2023 ). In general, the initial qualitative research tendency privileged case studies where individual students were asked to work on mathematical tasks to document their problem-solving performances. Later, research designs include the students’ participation in small groups and the analysis of students’ collaboration with the entire group (Brady et al., 2023 ). Bricolage frameworks that share tenets and information from different fields have become a powerful tool for researchers to understand complex people’ problem-solving proficiency (Lester, 2005 ; English, 2023 ).
5.1.2 Theoretical developments in mathematics education
In mathematics education, the constructivism perspective became relevant to orient and support research programs. Specifically, the recognition that students construct mathematical concepts and ideas through active participation as a part of a learning community that fosters and values what they bring into the classroom (eliciting students’ understanding) and sharing and discussing with peers their ways to work on mathematical activities. Further, it was recognized that students’ learning of mathematics takes place within a sociocultural environment (situated learning) that promotes the students’ interaction in small groups, pairs, and whole group discussions. Thus, problem-solving environments transited from teachers being a main figure to organize learning activities and to model problem-solving behaviours to being centred on students’ active participation to work on a variety of mathematical tasks as a part of a learning community (Lester & Cai, 2016 ). English ( 2023 ) proposed A STEM-based problem-solving framework that addresses the importance of a multidisciplinary approach and experiences to work on complex problems. Here, students develop a system of inquiry that integrates critical thinking, mathematical modelling, and a creative and innovative approach to deal with problematic situations situated in contexts beyond school problems. The STEM-based problem-solving framework enhances and favours the students’ development of multidisciplinary thinking to formulate and approach challenging problematic situations. To this end, they need to problematize information to characterize local and global problems and to collaboratively work on feasible approaches and solutions. It integrates 21st century skills that include an inquiry problem-solving approach to develop and exhibit critical thinking, creativity, and innovative solutions.
5.1.3 Countries or regional education traditions and their influence on the problem-solving agenda
The emergence of problem-solving frameworks takes place within an educational and socio-cultural context that provides conditions for their development and dissemination, but also limitations in their applications inside the mathematics education community. Brady et al. ( 2023 ) pointed out that:
…shifts in the theoretical frameworks of mathematics education researchers favored a widening of the view on problem solving from information-processing theories toward sociocultural theories that encouraged a conception of problem-solving as situated cognition unfolding within a community of practice (p. 34).
In addition, regional or national educational systems and research traditions also shape the problem-solving research and practice agenda. For example, in France, problem-solving approaches and research are framed in terms of two relevant theoretical and practical frameworks: Theory of Didactic Situation and the Anthropological Theory of Didactics (Artigue & Houdement, 2007 ). While, in the Netherlands, problem-solving approaches are situated within the theory of Realistic Mathematics that encourages and supports the students’ construction of meaning of concepts and methods in terms of modelling real-life and mathematical situations (Doorman et al., 2007 ). Ding et al. ( 2022 ) stated that the Chinese educational system refers to problem solving as an instructional goal and an approach to learn mathematics. Here, students deal with different types of problem-solving activities that include finding multiple solutions to one problem, one solution to multiple problems, and one problem multiple changes. Thus, ‘teaching with variation’ is emphasized in Chinese instruction in terms of “variations in solutions, presentations, and conditions/conclusions” (p. 482). Cai and Rott ( 2023 ) proposed a general problem-posing process model that distinguishes four problem-posing phases: Orientation (understanding the situation and what is required or is asked to pose); Connection that involves finding out or generating ideas and strategies to pose problems in different ways such as varying the given situation, or posing new problems; Generation refers to making the posed problem visible for others to understand it; and Reflection involves reflecting on her/his own process to pose the problem including ways to improve problem statements. The challenge in this model is to make explicit how the use of digital technologies can contribute to providing conditions for students to engage in all phases around problem- posing process.
5.1.4 Curriculum proposals and problem-solving teaching/learning scenarios
In the USA, the Common Core State Mathematics Standards curriculum proposal (CCSMS) identifies problem solving as a process standard that supports core mathematical practices that involve reasoning and proof, communication, representation, and connections. Thus, making sense of problems and persevering in solving them, reasoning abstractly and quantitatively, constructing viable arguments and critiquing the reasoning of others, modelling with mathematics, etc. are essential activities for students to develop mathematics proficiency and problem-solving approaches (Schoenfeld, 2023 ). In Singapore, the curriculum proposal identifies problem solving as the centre of its curriculum framework that relates its development with the study of concepts, skills, processes, attitudes, and metacognition (Lee et al., 2019 ). Recently, educational systems have begun to reform curriculum proposals to relate what the use of digital technologies demands in terms of selecting and structuring mathematical contents and ways to extend instructional settings (Engelbrecht & Borba, 2023 ). Indeed, Engelbrecht et al. ( 2023 ) identify what they call a classroom in movement or a distributed classroom - that transforms traditional cubic spaces to study the discipline into a movable setting that might combine remote and face-to-face students work.
It is argued that previous results in mathematical problem-solving research not only have contributed to recognize what is relevant and what common tenets distinguish and support problem-solving approaches; but also have provided bases to identify and pursue current problem-solving developments and directions. Hence, the consistent and coordinated use of several digital technologies and online developments (teaching and learning platforms) has opened new routes for learners to represent, explore, and work on mathematical problems; and to engage them in mathematical discussions beyond formal class settings. How does the students’ use of digital technologies expand the ways they reason and solve mathematical problems? What changes in classroom environments and physical settings are needed to recognize and include students’ face-to-face and remote work? (Engelbrecht et al., 2023 ).
In the next sections, the goal is to characterize the extent to which the consistent use of digital technologies and online developments provides affordances to restructure mathematical curriculum proposals and classrooms or learning settings and to enhance and expand students’ mathematical reasoning.
6 Current mathematical problem-solving trends and developments: the use of digital technologies
Although the use of technologies has been a recurrent theme in research studies, curriculum proposals, and teaching practices in mathematics education; during the COVID-pandemic lockdown, all teachers and students relied on digital technologies to work on mathematical tasks. At different phases, they developed and implemented not only novel paths to present, discuss, and approach teaching/learning activities; but also, ways to monitor and assess students’ problem-solving performances. When schools returned to teachers and students’ face-to-face activities, some questions emerged: What adjustments or changes in school practices are needed to consider and integrate those learning experiences that students developed during the social confinement? What digital tools should teachers and students use to work on mathematical tasks? How should teaching/learning practices reconcile students remote and face-to-face work? To address these questions, recent studies that involve ways to integrate technology in educational practices were reviewed, and their main themes and findings are organized and problematized to shed light on what the use of digital technologies contributes to frame and support learning environments.
6.1 The use of technology to reconceptualize students mathematical learning
There are different studies that document the importance and ways in which the students’ use of tools such as CAS or Excel offers an opportunity for them to think of concepts and problems in terms of different representations to transit from intuitive, visual, or graphic to formal or analytical reasoning (Arcavi et al., 2017 ). Others digital technologies, such as a Dynamic Geometry System Footnote 1 DGS, provide affordances for students to dynamically represent and explore mathematical problems. In students’ use of digital technologies, the problematizing principle becomes relevant to transform the tool into an instrument to work on mathematical tasks. Santos-Trigo ( 2019 ) provides examples where students rely on GeoGebra affordances to reconstruct figures that are given in problem statements; to transform routine problem into an investigation task; to model and explore tasks that involve variational reasoning; and to construct dynamic configurations to formulate and support mathematical relations. In this process, students not only exhibit diverse problem-solving strategies; but also, identify and integrate and use different concepts and resources that are studied in algebra, geometry, and calculus. That is, the use of technology provides an opportunity for students to integrate and connect knowledge from diverse areas or domains. For instance, Sinclair and Ferrara ( 2023 ) used the multi-touch application (TouchCounts) for children to work on mathematical challenging tasks.
6.2 The use of digital technologies to design a didactic route
There is indication, that the use of digital technologies offers different paths for students to learn mathematics (Leung & Bolite-Frant, 2015 ; Leung & Baccaglini-Frank, 2017 ). For instance, in the construction of a dynamic model of a problem, they are required to think of concepts and information embedded in the problem in terms of geometric representation or meaning. Thus, focusing on ways for students to represent and explore concepts geometrically could be the departure point to understand concepts and to solve mathematical problems. In addition, students can explore problems’ dynamic models (dragging schemes) in terms of visual, empirical, and graphic representations to initially identify relations that become relevant to approach and solve the problems. Thus, tool affordances become relevant for students to detect patterns, to formulate conjectures and to transit from empirical to formal argumentation to support problem solutions (Pittalis & Drijvers, 2023 ). Engelbrecht and Borba ( 2023 ) recognized that the prominent use of digital technologies in school mathematics has produced pedagogical shifts in teaching and learning practices to “encourage more active students learning, foster greater engagement, and provide more flexible access to learning’ (p. 1). Multiple use technologies such as internet, communication apps (ZOOM, Teams, Google Meet, etc.) become essential tools for teachers and students to present, communicate, and share information or to collaborate with peers. While tools used to represent, explore, and delve into concepts and to work and solve mathematical problems (Dynamic Geometry Systems, Wolframalpha, etc.) expand the students’ ways of reasoning and solving problems. Both types of technologies are not only important for teachers and students to continue working on school tasks beyond formal settings, but they also provide students with an opportunity to consult online resources such as Wikipedia or KhanAcademy to review or extend their concepts understanding, to analyse solved problems, and to contrast their teachers’ explanation of themes or concepts with those provided in learning platforms.
6.3 Students’ access to mathematics learning
Nowadays, cell phones are essential tools for people or students to interact or to approach diverse tasks and an educational challenge is how teachers/students can use them to work on mathematical tasks. During the COVID-19 social confinement, students relied on communication apps not only to interact with their teachers during class lectures; but also, to keep discussing tasks with peers beyond formal class meetings. That is, students realized that with the use of technology they could expand their learning space to include sharing and discussing ideas and problem solutions with peers beyond class sessions, consulting online learning platforms or material to review or extend their concepts understanding, and to watch videos to contrast experts’ concepts explanations and those provided by their teachers. In this perspective, the use of digital technologies increases the students’ access to different resources and the ways to work on mathematical tasks. Thus, available digital developments seem to extend the students collaborative work in addition to class activities. Furthermore, the flipped classroom model seems to offer certain advantages for students to learn the discipline and this model needs to be analysed in terms of what curriculum changes and ways to assess or monitor students learning are needed in its design and implementation (Cevikbas & Kaiser, 2022 ).
6.4 Changes in curriculum and mathematical assessment
It is recognized that the continuous development and availability of digital technologies is not only altering the ways in which individuals interact and face daily activities; but is also transforming educational practices and settings. Likewise, people’s concerns about multiple events or global problems such climate change, immigration, educational access, renewable resources, or racial conflicts or wars are themes that permeate the educational arena. Thus, curriculum reforms should address ways to connect students’ education with the analysis of these complex problems. English ( 2023 ) stated that:
The ill-defined problems of today, coupled with unexpected disruptions across all walks of life, demand advanced problem-solving by all citizens. The need to update outmoded forms of problem solving, which fail to take into account increasing global challenges, has never been greater (p.5).
In this perspective, mathematics curriculum needs to be structured around essential contents and habits of mathematical thinking for students to understand and make sense of real-world events that lead them to formulate, represent, and deal with a variety of problem situations. “Educators now increasingly seek to emphasise the practical applications of mathematics, such as modelling real-life scenarios and understanding statistical data (Engelbrecht & Borba, 2023 , p. 7). For instance, during the pandemic it was important to problematize the available data to follow, analyze and predict its spread behavior and to propose health measures to reduce people contagion. Thus, exponential functions, graphics, and their interpretations, data analysis, etc. were important mathematics content to understand the pandemic phenomena. Drijvers and Sinclair ( 2023 ) recognized that features of computational thinking share common grounds with mathematical thinking in terms of problem-solving activities that privilege model construction, the use of algorithms, abstraction processes and generalization of results. Thus, “a further integration of computational thinking in the mathematics curriculum is desirable”. In terms of ways to assess and monitor students’ learning, the idea is that with the use of a digital tool (digital wall or log), students could organize, structure, register, and monitor their individual and group work and learning experiences. That is, they could periodically report and share what difficulties they face to understand concepts or to work on a task, what questions they posed, what sources consult, etc. The information that appears in the digital wall is shared within the group and the teacher and students can provide feedback or propose new ideas or solutions (Santos-Trigo et al., 2022 ).
6.5 The integration of technologies and the emergence of conceptual frameworks
Institutions worldwide, in general, are integrating the use of different technologies in their educational practices, and they face the challenge to reconcile previous pandemic models and post confinement learning scenarios. “A pedagogical reason for using technology is to empower learners with extended or amplified abilities to acquire knowledge…technology can empower their cognitive abilities to reason in novice ways (Leung, 2011 , p. 327). Drijvers and Sinclair ( 2023 ) proposed a five-dimensional framework to delve into the rationale and purposes for the mathematics education community to integrate the use of digital technologies in mathematical teaching environments and students learning. The five interrelated categories address issues regarding how teachers and students’ use of digital technology contributes to reconceptualize and improve mathematics learning; to understand and explain how students’ mathematics learning develops; to design environments for mathematics learning; to foster and provide equitable access to mathematics learning; and to change mathematics curricula and teaching and assessment practices (Drijvers & Sinclair, 2023 ). Schoenfeld ( 2022 ) stated that “The challenge is to create robust learning environments that support every student in developing not only the knowledge and practices that underlie effective mathematical thinking, but that help them develop the sense of agency to engage in sense making” (p. 764). Højsted et al. ( 2022 ) argue about the importance of adjusting theoretical frameworks to explicitly integrate the use of digital technologies such as DGS and Computer Algebra Systems (CAS) in teaching practices. They referred to the Danish “Competencies and Mathematical Learning framework” (KOM) that gets articulated through tenets associated with the Theory of Instrumental Orchestration (TIO) and the notion of Justification Mediation (JM). In general terms, the idea is that learners get explicitly involved in a tool’ appropriation process that transforms the artifact into an instrument to understand concepts and to solve mathematical problems. That is, learners’ tool appropriation involves the development of cognitive schemata to rely on technology affordances to work on mathematical tasks. Koichu et al. ( 2022 ) pointed out that the incorporation of problem-solving approaches in instruction should be seen as a specific case of implementing innovation. To this end, they proposed a framework of problem-solving implementation chain that involves “a sequence of actions and interactions beginning with the development of a PS resource by researchers, which teachers then engage with in professional development (PD), and finally, teachers and students make use of in classrooms” (p. 4). In this case, problem-solving resources include the design of problematic situations (tasks) to engage students in mathematical discussions to make sense of problem statements or to ask them to pose a task.
7 Reflections and concluding remarks
Throughout different periods, the research and practice mathematical problem-solving agenda has contributed significantly to understand not only essentials in mathematical practices; but also, the development of conceptual frameworks to explain and document subjects’ cognitive, social, and affective behaviours to understand mathematical concepts and to develop problem-solving competencies. Leikin and Guberman ( 2023 ) pointed out that “…problem-solving is an effective didactical tool that allows pupils to mobilize their existing knowledge, construct new mathematical connections between known concepts and properties, and construct new knowledge in the process of overcoming challenges embedded in the problems” (p. 325). The study of people cognitive functioning to develop multidisciplinary knowledge and to solve problems involves documenting ways in which individuals make decisions regarding ways to organize their subject or disciplinary learning (how to interact with teachers or experts and peers; what material to consult, what tools to use, how to monitor their own learning, etc.) and to engage in disciplinary practices to achieve their learning goals. Both strategic and tactic decisions shape teachers and students’ ways to work on mathematical tasks. Kahneman ( 2011 ) shed light on how human beings make decisions to deal with questions and problematic situations. He argues that individuals rely on two systems to make decisions and engage in thinking processes; system one (fast thinking) that involves automatic, emotional, instinctive reasoning and system two (slow thinking) that includes logical, deliberative, effortful, or conscious reasoning. In educational tasks, the idea is that teachers and students develop experiences based on the construction and activation of system two. Thus, how teachers/students decide what tools or digital developments to use to work on mathematical problems becomes a relevant issue to address in the mathematics education agenda. Recent and consistent developments and the availability of digital technologies open novel paths for teachers and students to represent, explore, and approach mathematical tasks and, provide different tools to extend students and teachers’ mathematical discussions beyond classroom settings. In this perspective, it becomes important to discuss what changes the systematic use of digital technologies bring to the mathematics contents and to the ways to frame mathematical instruction. For example, the use of a Dynamic Geometry System to model and explore calculus, geometry or algebra classic problems dynamically not only offer students an opportunity to connect foundational concepts such as rate of change or the perpendicular bisector concept to geometrically study variational phenomena or conic sections; but also, to engage them in problem-posing activities (Santos-Trigo et al., 2021 ). Thus, teachers need to experience themselves different ways to use digital technologies to work on mathematical tasks and to identify instructional paths for students to internalize the use of digital apps as an instrument to understand concepts and to pose and formulate mathematical problems. Specifically, curriculum proposal should be structured around the development of foundational concepts and problem-solving strategies to formulate and pursue complex problems such as those involving climate changes, wealth distribution, immigration, pollution, mobility, connectivity, etc. To formulate and approach these problems, students need to develop a multidisciplinary thinking and rely on different tools to represent, explore, and share and continuously report partial solutions. To this end, they are encouraged to work with peers and groups as a part of learning community that fosters and values collective problem solutions. Finding multiple paths to solve problems becomes important for students to develop creative and innovative problem solutions (Leikin & Guberman, 2023 ). In this perspective, learning environments should provide conditions for students to transform digital applications in problem-solving tools to work on problematic situations. Online students’ assignments become an important component to structure and organize students and teachers’ face-to-face interactions. Likewise, the use of technology can also provide a tool for students to register and monitor their work and learning experiences. A digital wall or a problem-solving digital notebook (Santos-Trigo et al., 2022 ) could be introduced for students to register and monitor their learning experiences. Here, Students are asked to record on a weekly basis their work, questions, comments, and ideas that include: Questions they pose to understand concepts and problem statements; online resources and platforms they consult to contextualize problems and review and extend their understanding of involved concepts; concepts and strategies used to solve problems through different approaches; the Identification of other problems that can be solved with the methods that were used to solve the problem; digital technologies and online resources used to work on and solve the problem; dynamic models used to solve the problem and strategies used to identify and explore mathematical relations (dragging objects, measuring object attributes, tracing loci, using sliders, etc.; the formulation of new related problems including possible extensions for the initial problem; discussion of solutions of some new problems; and short recorded video presentation of their work and problem solutions. That is, the digital wall becomes an space for learners to share their work and to contrast and reflect on their peers work including extending their problem-solving approaches based on their teachers feedback and peers’ ideas or solutions.
The term Dynamic Geometry System is used, instead of Dynamic Geometry Environment or Dynamic Geometry Software, to emphasize that the app or tool interface encompasses a system of affordances that combines the construction of dynamic models, the use of Computer Algebra Systems and the use spreadsheet programs.
Arcavi, A., Drijvers, P., & Stacy, K. (2017). The learning and teaching of algebra. Ideas, insights, and activities . NY: Routledge. ISBN 9780415743723.
Artigue, M., & Houdement, C. (2007). Problem solving in France: Didactic and curricular perspectives. ZDM Int J Math Educ , 39 (5–6), 365–382. https://doi.org/10.1007/s11858-007-0048-x .
Article Google Scholar
Berger, W. (2014). A more beautiful question . Bloomsbury Publishing. Kindle Edition.
Brady, C., Ramírez, P., & Lesh, R. (2023). Problem posing and modeling: Confronting the dilemma of rigor or relevance. In T. L. Toh et al. (Eds.), Problem Posing and Problem Solving in Mathematics Education, pp: 33–50, Singapore: Springer. https://doi.org/10.1007/978-981-99-7205-0_3 .
Cai, J., & Hwang, S. (2023). Making mathematics challenging through problem posing in classroom. In R. Leikin (Ed.), Mathematical Challenges For All , Research in Mathematics Education, Springer: Switzerland, pp. 115–145, https://doi.org/10.1007/978-3-031-18868-8_7 .
Cai, J., & Rott, B. (2023). On understanding mathematical problem-posing processes. ZDM – Mathematics Education , 56 , 61–71. https://doi.org/10.1007/s11858-023-01536-w .
Cai, J., Hwang, S., & Melville, M. (2023). Mathematical problem-posing research: Thirty years of advances building on the publication of on mathematical problem solving. In J. Cai et al. (Eds.), Research Studies on Learning and Teaching of Mathematics, Research in Mathematics Education, Springer: Switzerland, pp: 1–25. https://doi.org/10.1007/978-3-031-35459-5_1 .
Cevikbas, M., & Kaiser, G. (2022). Can flipped classroom pedagogy offer promising perspectives for mathematics education on pan- demic-related issues? A systematic literature review. ZDM – Math- ematics Education . https://doi.org/10.1007/s11858-022-01388-w .
Cuoco, A., Goldenberg, E. P., & Mark, J. (1996). Habits of mind: An organizing principle for mathematics curricula. Journal of Mathematical Behavior , 15 , 375–402.
Devlin, K. (2002). The millennium problems. The seven greatest unsolved mathematical puzzles of our time . Granta.
Dick, T. P., & Hollebrands, K. F. (2011). Focus in high school mathematics: Technology to support reasoning and sense making . National Council of Teachers of Mathematics, NCTM: Reston Va. ISBN 978-0-87353-641-7.
Ding, M., Wu, Y., Liu, Q., & Cai, J. (2022). Mathematics learning in Chinese contexts. ZDM -Mathematics Education , 54 , 577–496. https://doi.org/10.1007/s11858-022-01385-z .
Doorman, M., Drijvers, P., Dekker, T., Van den Heuvel- Panhuizen, M., de Lange, J., & Wijers, M. (2007). Problem solving as a challenge for mathematics education in the Netherlands. ZDM Int J Math Educ , 39 (5–6), 405–418. https://doi.org/10.1007/s11858-007-0043-2 .
Drijvers, P., & Sinclair, N. (2023). The role of digital technologies in mathematics education: Purposes and perspectives. ZDM-Mathematics Education . https://doi.org/10.1007/s11858-023-01535-x .
Engelbrecht, J., & Borba, M. C. (2023). Recent developments in using digital technology in mathematics education. ZDM -Mathematics Education . https://doi.org/10.1007/s11858-023-01530-2 .
Engelbrecht, J., Borba, M. C., & Kaiser, G. (2023). Will we ever teach mathematics again in the way we used to before the pandemic? ZDM– Mathematics Education , 55 , 1–16. https://doi.org/10.1007/s11858-022-01460-5 .
English, L. D. (2023). Ways of thinking in STEM-based problem solving. ZDM -Mathematics Education . https://doi.org/10.1007/s11858-023-01474-7 .
English, L. D., & Kirshner, D. (Eds.). (2016). Handbook of international research in mathematics education . NY. ISBN: 978-0-203-44894-6 (ebk). https://www.routledge.com/Handbook-of-International-Research-in-Mathematics-Education/English-Kirshner/p/book/9780415832045
Fried, M. N. (2014). Mathematics & mathematics education: Searching for common ground. In M.N. Fried, T. Dreyfus (Eds.), Mathematics & Mathematics Education: Searching for 3 Common Ground , Advances in Mathematics Education, pp: 3–22. https://doi.org/10.1007/978-94-007-7473-5_1 . NY: Springer.
Halmos, P. (1980). The heart of mathematics. American Mathematical Monthly , 87 (7), 519–524.
Hiebert, J., Carpenter, T. P., Fennema, E., Fuson, K., Human, P., Murray, H., et al. (1996). Problem solving as a basis for reform in curriculum and instruction: The case of mathematics. Educational Researcher , 25 (4), 12–21.
Hilbert, D. (1902). Mathematical problems. Bulletin of the American Mathematical Society , 8 , 437–479.
Højsted, I. H., Geranius, E., & Jankvist, U. T. (2022). Teachers’ facilitation of students’ mathematical reasoning in a dynamic geometry environment: An analysis through three lenses. In U. T. Jankvist, & E. Geraniou (Eds.), Mathematical competencies in the Digital era (pp. 271–292). Springer. https://doi.org/10.1007/978-3-031-10141-0_15 .
Kahneman, D. (2011). Thinking, fast and slow . Farrar, Straus and Giroux.
Koichu, B. (2014). Problem solving in mathematics and in mathematics education. In M.N. Fried, T. Dreyfus (Eds.), Mathematics & Mathematics Education: Searching for 113 Common Ground , Advances in Mathematics Education, pp: 113–135. Dordrecht: Springer. https://doi.org/10.1007/978-94-007-7473-5_8 .
Koichu, B., Cooper, J., & Widder, M. (2022). Implementation of problem solving in school: From intended to experienced. Implementation and Replication Studies in Mathematics Education , 2 (1), 76–106. https://doi.org/10.1163/26670127-bja10004 .
Krutestkii, V. A. (1976). The psychology of mathematical abilities in school children . University of Chicago Press, Chicago. ISBN: 0-226-45492-4.
Lee, N. H., Ng, W. L., & Lim, L. G. P. (2019). The intended school mathematics curriculum. In T. L. Toh et al. (Eds.), Mathematics Education in Singapore , Mathematics Education – An Asian Perspective, pp: 35–53. https://doi.org/10.1007/978-981-13-3573-0_3 .
Leikin, R., & Guberman, R. (2023). Creativity and challenge: Task complexity as a function of insight and multiplicity of solutions. R. Leikin (Ed.), Mathematical Challenges For All , Research in Mathematics Education, pp: 325–342. https://doi.org/10.1007/978-3-031-18868-8_17 .
Lester, F. K. Jr. (1994). Musing about mathematical problem-solving research: 1970–1994. Journal for Research in Mathematics Education , 25 (6), 660–675.
Lester, F. K. Jr. (2005). On the theoretical, conceptual, and philosophical foundation for research in mathematics education. Zdm Mathematics Education , 37 (6), 457–467. https://doi.org/10.1007/BF02655854 .
Lester, F. K. Jr., & Cai, J. (2016). Can mathematical problem solving be taught? Preliminary answers from 30 years of research. In P. Felmer, et al. (Eds.), Posing and solving Mathematical problems, Research in Mathematics Education (pp. 117–135). Springer. https://doi.org/10.1007/978-3-319-28023-3_8 .
Leung, A. (2011). An epistemic model of task design in dynamic geometry environment. Zdm , 43 , 325–336. https://doi.org/10.1007/s11858-011-0329-2 .
Leung, A., & Baccaglini-Frank, A. (Eds.). (2017). (Eds.). Digital Technologies in Designing Mathematics Education Tasks, Mathematics Education in the Digital Era 8, https://doi.org/10.1007/978-3-319-43423-0_1 .
Leung, A., & Bolite-Frant, J. (2015). Designing mathematics tasks: The role of tools. In A. Watson, & M. Ohtani (Eds.), Task design in mathematics education (pp. 191–225). New ICMI Study Series. https://doi.org/10.1007/978-3-319-09629-2_6 .
Liljedahl, P., & Cai, J. (2021). Empirical research on problem solving and problem pos- ing: A look at the state of the art. ZDM — Mathematics Education , 53 (4), 723–735. https://doi.org/10.1007/s11858-021-01291-w .
Pittalis, M., & Drijvers, P. (2023). Embodied instrumentation in a dynamic geometry environment: Eleven-year‐old students’ dragging schemes. Educational Studies in Mathematics , 113 , 181–205. https://doi.org/10.1007/s10649-023-10222-3 .
Pólya, G. (1945).; 2nd edition, 1957). How to solve it . Princeton University Press.
Rott, B., Specht, B., & Knipping, C. (2021). A descritive phase model of problem-solving processes. ZDM -Mathematics Education , 53 , 737–752. https://doi.org/10.1007/s11858-021-01244-3 .
Santos-Trigo, M. (2019). Mathematical Problem Solving and the use of digital technologies. In P. Liljedahl and M. Santos-Trigo (Eds.). Mathematical Problem Solving. ICME 13 Monographs , ISBN 978-3-030-10471-9, ISBN 978-3-030-10472-6 (eBook), Springer Nature Switzerland AG. Pp. 63–89 https://doi.org/10.1007/978-3-030-10472-6_4 .
Santos-Trigo, M. (2020a). Problem-solving in mathematics education. In S. Lerman (Ed.), Encyclopedia of mathematics education (pp. 686–693). Springer. https://doi.org/10.1007/978-3-030-15789-0 .
Santos-Trigo, M. (2020b). Prospective and practicing teachers and the use of digital technologies in mathematical problem-solving approaches. In S. Llinares and O. Chapman (Eds.), International handbook of mathematics teacher education , vol 2, pp: 163–195. Boston: Brill Sense, ISBN 978-90-04-41896-7.
Santos-Trigo, M. (Ed.). (2023). Trends and developments of mathematical problem-solving research to update and support the use of digital technologies in post-confinement learning spaces. InT. L. Toh (Eds.), Problem Posing and Problem Solving in Mathematics Education , pp: 7–32. Springer Nature Singapore. https://doi.org/10.1007/978-981-99-7205-0_2 .
Santos-Trigo, M., & Reyes-Martínez, I. (2019). High school prospective teachers’ problem-solving reasoning that involves the coordinated use of digital technologies. International Journal of Mathematical Education in Science and Technology , 50 (2), 182–201. https://doi.org/10.1080/0020739X.2018.1489075 .
Santos-Trigo, M., Barrera-Mora, F., & Camacho-Machín, M. (2021). Teachers’ use of technology affordances to contextualize and dynamically enrich and extend mathematical problem-solving strategies. Mathematics , 9 (8), 793. https://doi.org/10.3390/math9080793 .
Santos-Trigo, M., Reyes-Martínez, I., & Gómez-Arciga, A. (2022). A conceptual framework to structure remote learning scenarios: A digital wall as a reflective tool for students to develop mathematics problem-solving competencies. Int J Learning Technology , 27–52. https://doi.org/10.1504/IJLT.2022.123686 .
Schoenfeld, A. H. (1992). Learning to think mathematically: Problem solving, metacognition, and sense making in mathematics. In D. A. Grows (Ed.), Handbook of research on mathematics teaching and learning (pp. 334–370). Macmillan.
Schoenfeld, A. H. (2020). Mathematical practices, in theory and practice. ZDM Mathematics Education, 52, pp: 1163–1175. https://doi.org/10.1007/s11858-020-01162-w .
Schoenfeld, A. H. (2022). Why are learning and teaching mathematics so difficult? In M. Danesi (Ed.), Handbook of cognitive mathematics (pp. 1–35). Switzerland. https://doi.org/10.1007/978-3-030-44982-7_10-1%23DOI .
Schoenfeld, A. H. (2023). A theory of teaching. In A. K. Praetorius, & C. Y. Charalambous (Eds.), Theorizing teaching (pp. 159–187). Springer. https://doi.org/10.1007/978-3-031-25613-4_6 .
Sinclair, N., & Ferrara, F. (2023). Towards a Socio-material Reframing of Mathematically Challenging Tasks. In R. Leikin (Ed.), Mathematical Challenges For All , Research in Mathematics Education, pp: 307–323. https://doi.org/10.1007/978-3-031-18868-8_16 .
Stake, R. E. (2000). Case studies. In N. K. Denzin, & Y. S. Lincoln (Eds.), Handbook of qualitative research (pp. 435–454). Sage.
Thurston, P. W. (1994). On proof and progress in mathematics. Bull Amer Math Soc , 30 (2), 161–177.
Toh, T. L., Santos-Trigo, M., Chua, P. H., Abdullah, N. A., & Zhang, D. (Eds.). (2023). Problem posing and problem solving in mathematics education: Internationa research and practice trends . Springer Nature Singpore. https://doi.org/10.1007/978-981-99-7205-0 .
Törner, G., Schoenfeld, A. H., & Reiss, K. M. (Eds.). (2007). Problem solving around the world: Summing up the state of the art [Special Issue]. ZDM — Mathematics Education , 39 (5–6). https://doi.org/10.1007/s11858-007-0053-0 .
Download references
Author information
Authors and affiliations.
Centre for Research and Advanced Studies, Mathematics Education Department, Mexico City, Mexico
Manuel Santos-Trigo
You can also search for this author in PubMed Google Scholar
Corresponding author
Correspondence to Manuel Santos-Trigo .
Additional information
Publisher’s note.
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons licence and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this licence, visit http://creativecommons.org/licenses/by/4.0/ .
Reprints and permissions
About this article
Santos-Trigo, M. Problem solving in mathematics education: tracing its foundations and current research-practice trends. ZDM Mathematics Education (2024). https://doi.org/10.1007/s11858-024-01578-8
Download citation
Accepted : 18 April 2024
Published : 30 April 2024
DOI : https://doi.org/10.1007/s11858-024-01578-8
Share this article
Anyone you share the following link with will be able to read this content:
Sorry, a shareable link is not currently available for this article.
Provided by the Springer Nature SharedIt content-sharing initiative
- Mathematical problem solving
- Conceptual frameworks
- Digital and semiotic tools-
- Mathematical reasoning
- Mathematics education developments
- Digital technologies
- Find a journal
- Publish with us
- Track your research
Math Problem Solving Strategies
In these lessons, we will learn some math problem solving strategies for example, Verbal Model (or Logical Reasoning), Algebraic Model, Block Model (or Singapore Math), Guess & Check Model and Find a Pattern Model.
Related Pages Solving Word Problems Using Block Models Heuristic Approach to Problem-Solving Algebra Lessons
Problem Solving Strategies
The strategies used in solving word problems:
- What do you know?
- What do you need to know?
- Draw a diagram/picture
Solution Strategies Label Variables Verbal Model or Logical Reasoning Algebraic Model - Translate Verbal Model to Algebraic Model Solve and Check.
Solving Word Problems
Step 1: Identify (What is being asked?) Step 2: Strategize Step 3: Write the equation(s) Step 4: Answer the question Step 5: Check
Problem Solving Strategy: Guess And Check
Using the guess and check problem solving strategy to help solve math word problems.
Example: Jamie spent $40 for an outfit. She paid for the items using $10, $5 and $1 bills. If she gave the clerk 10 bills in all, how many of each bill did she use?
Problem Solving : Make A Table And Look For A Pattern
- Identify - What is the question?
- Plan - What strategy will I use to solve the problem?
- Solve - Carry out your plan.
- Verify - Does my answer make sense?
Example: Marcus ran a lemonade stand for 5 days. On the first day, he made $5. Every day after that he made $2 more than the previous day. How much money did Marcus made in all after 5 days?
Find A Pattern Model (Intermediate)
In this lesson, we will look at some intermediate examples of Find a Pattern method of problem-solving strategy.
Example: The figure shows a series of rectangles where each rectangle is bounded by 10 dots. a) How many dots are required for 7 rectangles? b) If the figure has 73 dots, how many rectangles would there be?
| Rectangles | Pattern | Total dots |
| 1 | 10 | 10 |
| 2 | 10 + 7 | 17 |
| 3 | 10 + 14 | 24 |
| 4 | 10 + 21 | 31 |
| 5 | 10 + 28 | 38 |
| 6 | 10 + 35 | 45 |
| 7 | 10 + 42 | 52 |
| 8 | 10 + 49 | 59 |
| 9 | 10 + 56 | 66 |
| 10 | 10 + 63 | 73 |
a) The number of dots required for 7 rectangles is 52.
b) If the figure has 73 dots, there would be 10 rectangles.
Example: Each triangle in the figure below has 3 dots. Study the pattern and find the number of dots for 7 layers of triangles.
| Layers | Pattern | Total dots |
| 1 | 3 | 3 |
| 2 | 3 + 3 | 6 |
| 3 | 3 + 3 + 4 | 10 |
| 4 | 3 + 3 + 4 + 5 | 15 |
| 5 | 3 + 3 + 4 + 5 + 6 | 21 |
| 6 | 3 + 3 + 4 + 5 + 6 + 7 | 28 |
| 7 | 3 + 3 + 4 + 5 + 6 + 7 + 8 | 36 |
The number of dots for 7 layers of triangles is 36.
Example: The table below shows numbers placed into groups I, II, III, IV, V and VI. In which groups would the following numbers belong? a) 25 b) 46 c) 269
| I | 1 | 7 | 13 | 19 | 25 |
| II | 2 | 8 | 14 | 20 | 26 |
| III | 3 | 9 | 15 | 21 | 27 |
| IV | 4 | 10 | 16 | 22 | |
| V | 5 | 11 | 17 | 23 | |
| VI | 6 | 12 | 18 | 24 |
Solution: The pattern is: The remainder when the number is divided by 6 determines the group. a) 25 ÷ 6 = 4 remainder 1 (Group I) b) 46 ÷ 6 = 7 remainder 4 (Group IV) c) 269 ÷ 6 = 44 remainder 5 (Group V)
Example: The following figures were formed using matchsticks.
a) Based on the above series of figures, complete the table below.
| Number of squares | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
| Number of triangles | 4 | 6 | 8 | 10 | ||||
| Number of matchsticks | 12 | 19 | 26 | 33 |
b) How many triangles are there if the figure in the series has 9 squares?
c) How many matchsticks would be used in the figure in the series with 11 squares?
| Number of squares | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
| Number of triangles | 4 | 6 | 8 | 10 | 12 | 14 | 16 | 18 |
| Number of matchsticks | 12 | 19 | 26 | 33 | 40 | 47 | 54 | 61 |
b) The pattern is +2 for each additional square. 18 + 2 = 20 If the figure in the series has 9 squares, there would be 20 triangles.
c) The pattern is + 7 for each additional square 61 + (3 x 7) = 82 If the figure in the series has 11 squares, there would be 82 matchsticks.
Example: Seven ex-schoolmates had a gathering. Each one of them shook hands with all others once. How many handshakes were there?
| A | B | C | D | E | F | G | |
| A | |||||||
| B | ● | ||||||
| C | ● | ● | |||||
| D | ● | ● | ● | ||||
| E | ● | ● | ● | ● | |||
| F | ● | ● | ● | ● | ● | ||
| G | ● | ● | ● | ● | ● | ● | |
| HS | 6 | 5 | 4 | 3 | 2 | 1 |
Total = 6 + 5 + 4 + 3 + 2 + 1 = 21 handshakes.
The following video shows more examples of using problem solving strategies and models. Question 1: Approximate your average speed given some information Question 2: The table shows the number of seats in each of the first four rows in an auditorium. The remaining ten rows follow the same pattern. Find the number of seats in the last row. Question 3: You are hanging three pictures in the wall of your home that is 16 feet wide. The width of your pictures are 2, 3 and 4 feet. You want space between your pictures to be the same and the space to the left and right to be 6 inches more than between the pictures. How would you place the pictures?
The following are some other examples of problem solving strategies.
Explore it/Act it/Try it (EAT) Method (Basic) Explore it/Act it/Try it (EAT) Method (Intermediate) Explore it/Act it/Try it (EAT) Method (Advanced)
Finding A Pattern (Basic) Finding A Pattern (Intermediate) Finding A Pattern (Advanced)

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.
Problem solving
At its roots, problem solving is exactly what it sounds like, the process of solving problems. However, problem solving methods permeate the studies of mathematics , science , and technology . The human processes involved in problem solving are often studied by cognitive scientists .
What is Problem Solving?
by Richard Rusczyk
I was invited to the Math Olympiad Summer Program (MOP) in the 10th grade. I went to MOP certain that I must really be good at math… In my five weeks at MOP, I encountered over sixty problems on various tests. I didn’t solve a single one. That’s right – I was 0-for-60+. I came away no longer confident that I was good at math. I assumed that most of the other kids did better at MOP because they knew more tricks than I did. My formula sheets were pretty thorough, but perhaps they were missing something. By the end of MOP, I had learned a somewhat unsettling truth. The others knew fewer tricks than I did, not more. They didn’t even have formula sheets!
At another contest later that summer, a younger student, Alex, from another school asked me for my formula sheets. In my local and state circles, students’ formula sheets were the source of knowledge, the source of power that fueled the top students and the top schools. They were studied, memorized, revered. But most of all, they were not shared. But when Alex asked for my formula sheets I remembered my experience at MOP and I realized that formula sheets are not really math. Memorizing formulas is no more mathematics than memorizing dates is history or memorizing spelling words is literature. I gave him the formula sheets. (Alex must later have learned also that the formula sheets were fool’s gold – he became a Rhodes scholar.)
The difference between MOP and many of these state and local contests I participated in was the difference between problem solving and what many people call mathematics. For these people, math is a series of tricks to use on a series of specific problems. Trick A is for Problem A, Trick B for Problem B, and so on. In this vein, school can become a routine of ‘learn tricks for a week – use tricks on a test – forget most tricks quickly.’ The tricks get forgotten quickly primarily because there are so many of them, and also because the students don’t see how these ‘tricks’ are just extensions of a few basic principles.
I had painfully learned at MOP that true mathematics is not a process of memorizing formulas and applying them to problems tailor-made for those formulas. Instead, the successful mathematician possesses fewer tools, but knows how to apply them to a much broader range of problems. We use the term “problem solving” to distinguish this approach to mathematics from the ‘memorize-use-forget’ approach.
After MOP I relearned math throughout high school. I was unaware that I was learning much more. When I got to Princeton I enrolled in organic chemistry. There were over 200 students in the course, and we quickly separated into two groups. One group understood that all we would be taught could largely be derived from a very small number of basic principles. We loved the class – it was a year long exploration of where these fundamental concepts could take us. The other, much larger, group saw each new destination not as the result of a path from the building blocks, but as yet another place whose coordinates had to be memorized if ever they were to visit again. Almost to a student, the difference between those in the happy group and those in the struggling group was how they learned mathematics. The class seemingly involved no math at all, but those who took a memorization approach to math were doomed to do it again in chemistry. The skills the problem solvers developed in math transferred, and these students flourished.
We use math to teach problem solving because it is the most fundamental logical discipline. Not only is it the foundation upon which sciences are built, it is the clearest way to learn and understand how to develop a rigorous logical argument. There are no loopholes, there are no half-truths. The language of mathematics is precise, as is ‘right’ and ‘wrong’ (or ‘proven’ and ‘unproven’). Success and failure are immediate and indisputable; there isn’t room for subjectivity. This is not to say that those who cannot do math cannot solve problems. There are many paths to strong problem solving skills. Mathematics is the shortest.
Problem solving is crucial in mathematics education because it transcends mathematics. By developing problem solving skills, we learn not only how to tackle math problems, but also how to logically work our way through any problems we may face. The memorizer can only solve problems he has encountered already, but the problem solver can solve problems she’s never seen before. The problem solver is flexible; she can diversify. Above all, she can create.
There are four main steps in successful problem solving — not just in math or even computer science, but also in everyday life (even figuring out how to beat your rival in a video game).
- Identify the Problem : The very first step in problem solving is to identify the problem. Problem solving is harder if one doesn’t know what “problem” to solve! This is often seen in engineering and math by reading the problem statement.
- Make a Game Plan : The next step is to have a plan on what to do. In other words, just rushing in won’t usually go through. Know what skills, properties, and information would be useful in aiding your decisions. Don’t forget about backups in case Plan A fails.
- Solve the Problem : As Rusczyk often says, sometimes, you just have to do something. A problem can’t be solved by just staring at it!
- Reflect on Actions : Skilled problem solvers look back at their work. In math, it usually means figuring out if an answer is reasonable. In engineering (and other areas), problem solvers can look back and see what went right and what went wrong, so they can tackle a similar problem better next time.
Something appears to not have loaded correctly.
Click to refresh .
Mathematics Through Problem Solving
What Is A ‘Problem-Solving Approach’?

- interactions between students/students and teacher/students (Van Zoest et al., 1994)
- mathematical dialogue and consensus between students (Van Zoest et al., 1994)
- teachers providing just enough information to establish background/intent of the problem, and students clarifing, interpreting, and attempting to construct one or more solution processes (Cobb et al., 1991)
- teachers accepting right/wrong answers in a non-evaluative way (Cobb et al., 1991)
- teachers guiding, coaching, asking insightful questions and sharing in the process of solving problems (Lester et al., 1994)
- teachers knowing when it is appropriate to intervene, and when to step back and let the pupils make their own way (Lester et al., 1994)
- A further characteristic is that a problem-solving approach can be used to encourage students to make generalisations about rules and concepts, a process which is central to mathematics (Evan and Lappin, 1994).
Schoenfeld (in Olkin and Schoenfeld, 1994, p.43) described the way in which the use of problem solving in his teaching has changed since the 1970s:
My early problem-solving courses focused on problems amenable to solutions by Polya-type heuristics: draw a diagram, examine special cases or analogies, specialize, generalize, and so on. Over the years the courses evolved to the point where they focused less on heuristics per se and more on introducing students to fundamental ideas: the importance of mathematical reasoning and proof…, for example, and of sustained mathematical investigations (where my problems served as starting points for serious explorations, rather than tasks to be completed).
Schoenfeld also suggested that a good problem should be one which can be extended to lead to mathematical explorations and generalisations. He described three characteristics of mathematical thinking:
- valuing the processes of mathematization and abstraction and having the predilection to apply them
- developing competence with the tools of the trade and using those tools in the service of the goal of understanding structure – mathematical sense-making (Schoenfeld, 1994, p.60).
- As Cobb et al. (1991) suggested, the purpose for engaging in problem solving is not just to solve specific problems, but to ‘encourage the interiorization and reorganization of the involved schemes as a result of the activity’ (p.187). Not only does this approach develop students’ confidence in their own ability to think mathematically (Schifter and Fosnot, 1993), it is a vehicle for students to construct, evaluate and refine their own theories about mathematics and the theories of others (NCTM, 1989). Because it has become so predominant a requirement of teaching, it is important to consider the processes themselves in more detail.
The Role of Problem Solving in Teaching Mathematics as a Process
Problem solving is an important component of mathematics education because it is the single vehicle which seems to be able to achieve at school level all three of the values of mathematics listed at the outset of this article: functional, logical and aesthetic. Let us consider how problem solving is a useful medium for each of these.
It has already been pointed out that mathematics is an essential discipline because of its practical role to the individual and society. Through a problem-solving approach, this aspect of mathematics can be developed. Presenting a problem and developing the skills needed to solve that problem is more motivational than teaching the skills without a context. Such motivation gives problem solving special value as a vehicle for learning new concepts and skills or the reinforcement of skills already acquired (Stanic and Kilpatrick, 1989, NCTM, 1989). Approaching mathematics through problem solving can create a context which simulates real life and therefore justifies the mathematics rather than treating it as an end in itself. The National Council of Teachers of Mathematics (NCTM, 1980) recommended that problem solving be the focus of mathematics teaching because, they say, it encompasses skills and functions which are an important part of everyday life. Furthermore it can help people to adapt to changes and unexpected problems in their careers and other aspects of their lives. More recently the Council endorsed this recommendation (NCTM, 1989) with the statement that problem solving should underly all aspects of mathematics teaching in order to give students experience of the power of mathematics in the world around them. They see problem solving as a vehicle for students to construct, evaluate and refine their own theories about mathematics and the theories of others.
According to Resnick (1987) a problem-solving approach contributes to the practical use of mathematics by helping people to develop the facility to be adaptable when, for instance, technology breaks down. It can thus also help people to transfer into new work environments at this time when most are likely to be faced with several career changes during a working lifetime (NCTM, 1989). Resnick expressed the belief that ‘school should focus its efforts on preparing people to be good adaptive learners, so that they can perform effectively when situations are unpredictable and task demands change’ (p.18). Cockcroft (1982) also advocated problem solving as a means of developing mathematical thinking as a tool for daily living, saying that problem-solving ability lies ‘at the heart of mathematics’ (p.73) because it is the means by which mathematics can be applied to a variety of unfamiliar situations.
Problem solving is, however, more than a vehicle for teaching and reinforcing mathematical knowledge and helping to meet everyday challenges. It is also a skill which can enhance logical reasoning. Individuals can no longer function optimally in society by just knowing the rules to follow to obtain a correct answer. They also need to be able to decide through a process of logical deduction what algorithm, if any, a situation requires, and sometimes need to be able to develop their own rules in a situation where an algorithm cannot be directly applied. For these reasons problem solving can be developed as a valuable skill in itself, a way of thinking (NCTM, 1989), rather than just as the means to an end of finding the correct answer.
Many writers have emphasised the importance of problem solving as a means of developing the logical thinking aspect of mathematics. ‘If education fails to contribute to the development of the intelligence, it is obviously incomplete. Yet intelligence is essentially the ability to solve problems: everyday problems, personal problems … ‘(Polya, 1980, p.1). Modern definitions of intelligence (Gardner, 1985) talk about practical intelligence which enables ‘the individual to resolve genuine problems or difficulties that he or she encounters’ (p.60) and also encourages the individual to find or create problems ‘thereby laying the groundwork for the acquisition of new knowledge’ (p.85). As was pointed out earlier, standard mathematics, with the emphasis on the acquisition of knowledge, does not necessarily cater for these needs. Resnick (1987) described the discrepancies which exist between the algorithmic approaches taught in schools and the ‘invented’ strategies which most people use in the workforce in order to solve practical problems which do not always fit neatly into a taught algorithm. As she says, most people have developed ‘rules of thumb’ for calculating, for example, quantities, discounts or the amount of change they should give, and these rarely involve standard algorithms. Training in problem-solving techniques equips people more readily with the ability to adapt to such situations.
A further reason why a problem-solving approach is valuable is as an aesthetic form. Problem solving allows the student to experience a range of emotions associated with various stages in the solution process. Mathematicians who successfully solve problems say that the experience of having done so contributes to an appreciation for the ‘power and beauty of mathematics’ (NCTM, 1989, p.77), the “joy of banging your head against a mathematical wall, and then discovering that there might be ways of either going around or over that wall” (Olkin and Schoenfeld, 1994, p.43). They also speak of the willingness or even desire to engage with a task for a length of time which causes the task to cease being a ‘puzzle’ and allows it to become a problem. However, although it is this engagement which initially motivates the solver to pursue a problem, it is still necessary for certain techniques to be available for the involvement to continue successfully. Hence more needs to be understood about what these techniques are and how they can best be made available.
In the past decade it has been suggested that problem-solving techniques can be made available most effectively through making problem solving the focus of the mathematics curriculum. Although mathematical problems have traditionally been a part of the mathematics curriculum, it has been only comparatively recently that problem solving has come to be regarded as an important medium for teaching and learning mathematics (Stanic and Kilpatrick, 1989). In the past problem solving had a place in the mathematics classroom, but it was usually used in a token way as a starting point to obtain a single correct answer, usually by following a single ‘correct’ procedure. More recently, however, professional organisations such as the National Council of Teachers of Mathematics (NCTM, 1980 and 1989) have recommended that the mathematics curriculum should be organized around problem solving, focusing on:
- developing skills and the ability to apply these skills to unfamiliar situations
- gathering, organising, interpreting and communicating information
- formulating key questions, analyzing and conceptualizing problems, defining problems and goals, discovering patterns and similarities, seeking out appropriate data, experimenting, transferring skills and strategies to new situations
- developing curiosity, confidence and open-mindedness (NCTM, 1980, pp.2-3).
One of the aims of teaching through problem solving is to encourage students to refine and build onto their own processes over a period of time as their experiences allow them to discard some ideas and become aware of further possibilities (Carpenter, 1989). As well as developing knowledge, the students are also developing an understanding of when it is appropriate to use particular strategies. Through using this approach the emphasis is on making the students more responsible for their own learning rather than letting them feel that the algorithms they use are the inventions of some external and unknown ‘expert’. There is considerable importance placed on exploratory activities, observation and discovery, and trial and error. Students need to develop their own theories, test them, test the theories of others, discard them if they are not consistent, and try something else (NCTM, 1989). Students can become even more involved in problem solving by formulating and solving their own problems, or by rewriting problems in their own words in order to facilitate understanding. It is of particular importance to note that they are encouraged to discuss the processes which they are undertaking, in order to improve understanding, gain new insights into the problem and communicate their ideas (Thompson, 1985, Stacey and Groves, 1985).
It has been suggested in this chapter that there are many reasons why a problem-solving approach can contribute significantly to the outcomes of a mathematics education. Not only is it a vehicle for developing logical thinking, it can provide students with a context for learning mathematical knowledge, it can enhance transfer of skills to unfamiliar situations and it is an aesthetic form in itself. A problem-solving approach can provide a vehicle for students to construct their own ideas about mathematics and to take responsibility for their own learning. There is little doubt that the mathematics program can be enhanced by the establishment of an environment in which students are exposed to teaching via problem solving, as opposed to more traditional models of teaching about problem solving. The challenge for teachers, at all levels, is to develop the process of mathematical thinking alongside the knowledge and to seek opportunities to present even routine mathematics tasks in problem-solving contexts.
Example #1 – Mathematical Treasure Hunt
Objective – The objective of this activity is to encourage students to apply their problem-solving skills while having fun exploring mathematical concepts in a real-world context.
Materials Needed
Paper and pencils for each student Treasure map (could be a printed map or drawn by hand) Clues (math-related questions or puzzles) Optional: Small prizes or rewards for completing the treasure hunt Instructions:
Introduction (5 minutes)
Begin by introducing the activity to the students. Explain that they will be going on a mathematical treasure hunt where they will solve math problems to uncover hidden clues leading them to the treasure. Emphasize that this activity will require their problem-solving skills and teamwork.
Setting Up the Treasure Hunt (10 minutes)
Prepare a treasure map with different locations marked on it. These locations could be scattered around the classroom, school, or any other designated area. Hide clues at each location that will lead the students to the next destination.
Creating Clues (15 minutes)
Create math-related clues or puzzles that the students will need to solve to uncover the next location on the treasure map. The clues should be age-appropriate and aligned with the students’ math skills. For example:
Solve the following addition problem to reveal the next clue: 15 + 27 – 9 = ?
Count the number of chairs in the classroom and multiply by 3 to find the next location.
Find the area of the square-shaped rug in the library to unlock the next clue.
Starting the Treasure Hunt (5 minutes)
Divide the students into small groups or pairs, depending on the class size. Provide each group with a treasure map and the first clue. Explain the rules of the treasure hunt and encourage students to work together to solve the clues.
Exploring and Solving Clues (30 minutes)
Allow the students to begin the treasure hunt. As they solve each clue, they will uncover the location of the next clue on the treasure map. Encourage them to discuss and collaborate on the solutions to the math problems. Circulate around the room to provide assistance and guidance as needed.
Finding the Treasure (10 minutes)
Once the students have solved all the clues and reached the final location on the treasure map, they will discover the hidden treasure.
Congratulate them on their problem-solving skills and teamwork. You can optionally reward the students with small prizes or certificates for completing the treasure hunt successfully.
Reflection and Discussion (10 minutes)
After the treasure hunt, gather the students together for a brief reflection and discussion. Ask them about their favorite part of the activity, the challenges they faced, and what they learned from solving the math problems. Encourage them to share their strategies and insights with the class.
Extension Ideas
Create themed treasure hunts based on specific mathematical concepts such as geometry, fractions, or measurement.
Invite students to design their own treasure hunts for their classmates, incorporating math problems and creative clues.
Integrate technology by using QR codes or digital maps to lead students to each clue location.
By engaging students in a fun and interactive math problem-solving activity like the “Mathematical Treasure Hunt,” educators can foster a positive attitude towards mathematics while strengthening students’ critical thinking and collaboration skills.
Example #2 – Math Maze Adventure
Objective – The objective of this activity is to challenge students’ problem-solving abilities while navigating through a maze filled with math-related obstacles and puzzles.
Large maze layout (could be drawn on a poster board or printed) Dice Game tokens or markers for each student Math problem cards (with varying difficulty levels) Stopwatch or timer Optional: Prizes or rewards for completing the maze within a certain time limit
Instructions
Begin by introducing the “Math Maze Adventure” to the students. Explain that they will embark on a thrilling journey through a maze filled with mathematical challenges that they must overcome using their problem-solving skills.
Setting Up the Maze (10 minutes)
Create a large maze layout on a poster board or print one from a maze generator website. Designate a starting point and an endpoint within the maze. Place obstacles and challenges throughout the maze, such as math problems, riddles, or puzzles.
Preparing Math Problem Cards (15 minutes)
Create a set of math problem cards with varying difficulty levels. These problems could involve arithmetic operations, geometry concepts, fractions, or any other relevant math topics. Write each problem on a separate card and mix them up.
Starting the Adventure (5 minutes)
Divide the students into small groups or pairs, depending on the class size. Provide each group with a game token or marker to represent their position in the maze. Explain the rules of the game and how to navigate through the maze.
Navigating the Maze (30 minutes)
Start the timer and allow the students to begin their “Math Maze Adventure.” They will roll the dice to determine how many spaces they can move in the maze. When they land on a space with a math problem, they must draw a problem card and solve it correctly to proceed.
Solving Math Problems (30 minutes)
As students encounter math problems in the maze, they will work together to solve them. Encourage them to discuss strategies, share ideas, and check each other’s work. If they solve the problem correctly, they can continue moving through the maze. If not, they must stay in place until they solve it.
Reaching the Endpoint (10 minutes)
The goal of the “Math Maze Adventure” is to reach the endpoint of the maze within a certain time limit. Students must use their problem-solving skills and teamwork to overcome obstacles and challenges along the way. If they reach the endpoint before time runs out, they win the game!
After completing the maze, gather the students together for a reflection and discussion. Ask them about their experience navigating through the maze, the math problems they encountered, and the strategies they used to solve them. Encourage them to share their insights and lessons learned.
Create multiple versions of the maze with different layouts and levels of difficulty to provide ongoing challenges for students.
Integrate storytelling elements into the maze adventure, with each space representing a different part of the story that unfolds as students progress.
Incorporate technology by using a digital maze app or online platform to create and navigate through virtual mazes with math challenges.
The “Math Maze Adventure” offers an exciting and interactive way for students to practice their problem-solving skills while embarking on a thrilling journey through a maze filled with mathematical challenges. Through teamwork, critical thinking, and perseverance, students will navigate their way to success!
Carpenter, T. P. (1989). ‘Teaching as problem solving’. In R.I.Charles and E.A. Silver (Eds), The Teaching and Assessing of Mathematical Problem Solving, (pp.187-202). USA: National Council of Teachers of Mathematics.
Clarke, D. and McDonough, A. (1989). ‘The problems of the problem solving classroom’, The Australian Mathematics Teacher, 45, 3, 20-24.
Cobb, P., Wood, T. and Yackel, E. (1991). ‘A constructivist approach to second grade mathematics’. In von Glaserfield, E. (Ed.), Radical Constructivism in Mathematics Education, pp. 157-176. Dordrecht, The Netherlands: Kluwer Academic Publishers.
Cockcroft, W.H. (Ed.) (1982). Mathematics Counts. Report of the Committee of Inquiry into the Teaching of Mathematics in Schools, London: Her Majesty’s Stationery Office.
Evan, R. and Lappin, G. (1994). ‘Constructing meaningful understanding of mathematics content’, in Aichele, D. and Coxford, A. (Eds.) Professional Development for Teachers of Mathematics , pp. 128-143. Reston, Virginia: NCTM.
Gardner, Howard (1985). Frames of Mind. N.Y: Basic Books.
Lester, F.K.Jr., Masingila, J.O., Mau, S.T., Lambdin, D.V., dos Santon, V.M. and Raymond, A.M. (1994). ‘Learning how to teach via problem solving’. in Aichele, D. and Coxford, A. (Eds.) Professional Development for Teachers of Mathematics , pp. 152-166. Reston, Virginia: NCTM.
National Council of Teachers of Mathematics (NCTM) (1980). An Agenda for Action: Recommendations for School Mathematics of the 1980s, Reston, Virginia: NCTM.
National Council of Teachers of Mathematics (NCTM) (1989). Curriculum and Evaluation Standards for School Mathematics, Reston, Virginia: NCTM.
Olkin, I. & Schoenfeld, A. (1994). A discussion of Bruce Reznick’s chapter. In A. Schoenfeld (Ed.). Mathematical Thinking and Problem Solving. (pp. 39-51). Hillsdale, NJ: Lawrence Erlbaum Associates.
Polya, G. (1980). ‘On solving mathematical problems in high school’. In S. Krulik (Ed). Problem Solving in School Mathematics, (pp.1-2). Reston, Virginia: NCTM.
Resnick, L. B. (1987). ‘Learning in school and out’, Educational Researcher, 16, 13-20..
Romberg, T. (1994). Classroom instruction that fosters mathematical thinking and problem solving: connections between theory and practice. In A. Schoenfeld (Ed.). Mathematical Thinking and Problem Solving. (pp. 287-304). Hillsdale, NJ: Lawrence Erlbaum Associates.
Schifter, D. and Fosnot, C. (1993). Reconstructing Mathematics Education. NY: Teachers College Press.
Schoenfeld, A. (1994). Reflections on doing and teaching mathematics. In A. Schoenfeld (Ed.). Mathematical Thinking and Problem Solving. (pp. 53-69). Hillsdale, NJ: Lawrence Erlbaum Associates.
Stacey, K. and Groves, S. (1985). Strategies for Problem Solving, Melbourne, Victoria: VICTRACC.
Stanic, G. and Kilpatrick, J. (1989). ‘Historical perspectives on problem solving in the mathematics curriculum’. In R.I. Charles and E.A. Silver (Eds), The Teaching and Assessing of Mathematical Problem Solving, (pp.1-22). USA: National Council of Teachers of Mathematics.
Swafford, J.O. (1995). ‘Teacher preparation’. in Carl, I.M. (Ed.) Prospects for School Mathematics , pp. 157-174. Reston, Virginia: NCTM.
Thompson, P. W. (1985). ‘Experience, problem solving, and learning mathematics: considerations in developing mathematics curricula’. In E.A. Silver (Ed.), Teaching and Learning Mathematical Problem Solving: Multiple Research Perspectives, (pp.189-236). Hillsdale, N.J: Lawrence Erlbaum.
Van Zoest, L., Jones, G. and Thornton, C. (1994). ‘Beliefs about mathematics teaching held by pre-service teachers involved in a first grade mentorship program’. Mathematics Education Research Journal. 6(1): 37-55.
Related Article on Teaching Values | Other Articles
Problem Solving in Mathematics
- Math Tutorials
- Pre Algebra & Algebra
- Exponential Decay
- Worksheets By Grade
The main reason for learning about math is to become a better problem solver in all aspects of life. Many problems are multistep and require some type of systematic approach. There are a couple of things you need to do when solving problems. Ask yourself exactly what type of information is being asked for: Is it one of addition, subtraction, multiplication , or division? Then determine all the information that is being given to you in the question.
Mathematician George Pólya’s book, “ How to Solve It: A New Aspect of Mathematical Method ,” written in 1957, is a great guide to have on hand. The ideas below, which provide you with general steps or strategies to solve math problems, are similar to those expressed in Pólya’s book and should help you untangle even the most complicated math problem.
Use Established Procedures
Learning how to solve problems in mathematics is knowing what to look for. Math problems often require established procedures and knowing what procedure to apply. To create procedures, you have to be familiar with the problem situation and be able to collect the appropriate information, identify a strategy or strategies, and use the strategy appropriately.
Problem-solving requires practice. When deciding on methods or procedures to use to solve problems, the first thing you will do is look for clues, which is one of the most important skills in solving problems in mathematics. If you begin to solve problems by looking for clue words, you will find that these words often indicate an operation.
Look for Clue Words
Think of yourself as a math detective. The first thing to do when you encounter a math problem is to look for clue words. This is one of the most important skills you can develop. If you begin to solve problems by looking for clue words, you will find that those words often indicate an operation.
Common clue words for addition problems:
Common clue words for subtraction problems:
- How much more
Common clue words for multiplication problems:
Common clue words for division problems:
Although clue words will vary a bit from problem to problem, you'll soon learn to recognize which words mean what in order to perform the correct operation.
Read the Problem Carefully
This, of course, means looking for clue words as outlined in the previous section. Once you’ve identified your clue words, highlight or underline them. This will let you know what kind of problem you’re dealing with. Then do the following:
- Ask yourself if you've seen a problem similar to this one. If so, what is similar about it?
- What did you need to do in that instance?
- What facts are you given about this problem?
- What facts do you still need to find out about this problem?
Develop a Plan and Review Your Work
Based on what you discovered by reading the problem carefully and identifying similar problems you’ve encountered before, you can then:
- Define your problem-solving strategy or strategies. This might mean identifying patterns, using known formulas, using sketches, and even guessing and checking.
- If your strategy doesn't work, it may lead you to an ah-ha moment and to a strategy that does work.
If it seems like you’ve solved the problem, ask yourself the following:
- Does your solution seem probable?
- Does it answer the initial question?
- Did you answer using the language in the question?
- Did you answer using the same units?
If you feel confident that the answer is “yes” to all questions, consider your problem solved.
Tips and Hints
Some key questions to consider as you approach the problem may be:
- What are the keywords in the problem?
- Do I need a data visual, such as a diagram, list, table, chart, or graph?
- Is there a formula or equation that I'll need? If so, which one?
- Will I need to use a calculator? Is there a pattern I can use or follow?
Read the problem carefully, and decide on a method to solve the problem. Once you've finished working the problem, check your work and ensure that your answer makes sense and that you've used the same terms and or units in your answer.
- Converting Cubic Meters to Liters
- 2nd Grade Math Word Problems
- 2020-21 Common Application Essay Option 4—Solving a Problem
- The Horse Problem: A Math Challenge
- How to Use Math Journals in Class
- The Frayer Model for Math
- Algorithms in Mathematics and Beyond
- "Grandpa's Rubik's Cube"—Sample Common Application Essay, Option #4
- Critical Thinking Definition, Skills, and Examples
- Math Stumper: Use Two Squares to Make Separate Pens for Nine Pigs
- Graphic Organizers in Math
- College Interview Tips: "Tell Me About a Challenge You Overcame"
- Christmas Word Problem Worksheets
- Solving Problems Involving Distance, Rate, and Time
- Study Tips for Math Homework and Math Tests
- Innovative Ways to Teach Math
Center for Teaching
Teaching problem solving.
Print Version
Tips and Techniques
Expert vs. novice problem solvers, communicate.
- Have students identify specific problems, difficulties, or confusions . Don’t waste time working through problems that students already understand.
- If students are unable to articulate their concerns, determine where they are having trouble by asking them to identify the specific concepts or principles associated with the problem.
- In a one-on-one tutoring session, ask the student to work his/her problem out loud . This slows down the thinking process, making it more accurate and allowing you to access understanding.
- When working with larger groups you can ask students to provide a written “two-column solution.” Have students write up their solution to a problem by putting all their calculations in one column and all of their reasoning (in complete sentences) in the other column. This helps them to think critically about their own problem solving and helps you to more easily identify where they may be having problems. Two-Column Solution (Math) Two-Column Solution (Physics)
Encourage Independence
- Model the problem solving process rather than just giving students the answer. As you work through the problem, consider how a novice might struggle with the concepts and make your thinking clear
- Have students work through problems on their own. Ask directing questions or give helpful suggestions, but provide only minimal assistance and only when needed to overcome obstacles.
- Don’t fear group work ! Students can frequently help each other, and talking about a problem helps them think more critically about the steps needed to solve the problem. Additionally, group work helps students realize that problems often have multiple solution strategies, some that might be more effective than others
Be sensitive
- Frequently, when working problems, students are unsure of themselves. This lack of confidence may hamper their learning. It is important to recognize this when students come to us for help, and to give each student some feeling of mastery. Do this by providing positive reinforcement to let students know when they have mastered a new concept or skill.
Encourage Thoroughness and Patience
- Try to communicate that the process is more important than the answer so that the student learns that it is OK to not have an instant solution. This is learned through your acceptance of his/her pace of doing things, through your refusal to let anxiety pressure you into giving the right answer, and through your example of problem solving through a step-by step process.
Experts (teachers) in a particular field are often so fluent in solving problems from that field that they can find it difficult to articulate the problem solving principles and strategies they use to novices (students) in their field because these principles and strategies are second nature to the expert. To teach students problem solving skills, a teacher should be aware of principles and strategies of good problem solving in his or her discipline .
The mathematician George Polya captured the problem solving principles and strategies he used in his discipline in the book How to Solve It: A New Aspect of Mathematical Method (Princeton University Press, 1957). The book includes a summary of Polya’s problem solving heuristic as well as advice on the teaching of problem solving.

Teaching Guides
- Online Course Development Resources
- Principles & Frameworks
- Pedagogies & Strategies
- Reflecting & Assessing
- Challenges & Opportunities
- Populations & Contexts
Quick Links
- Services for Departments and Schools
- Examples of Online Instructional Modules
You are using an outdated browser. Please upgrade your browser to improve your experience.
Math Problem Solving Strategies That Make Students Say “I Get It!”

Even students who are quick with math facts can get stuck when it comes to problem solving.
As soon as a concept is translated to a word problem, or a simple mathematical sentence contains an unknown, they’re stumped.
That’s because problem solving requires us to consciously choose the strategies most appropriate for the problem at hand . And not all students have this metacognitive ability.
But you can teach these strategies for problem solving. You just need to know what they are.
We’ve compiled them here divided into four categories:
Strategies for understanding a problem
Strategies for solving the problem, strategies for working out, strategies for checking the solution.
Get to know these strategies and then model them explicitly to your students. Next time they dive into a rich problem, they’ll be filling up their working out paper faster than ever!
Before students can solve a problem, they need to know what it’s asking them. This is often the first hurdle with word problems that don’t specify a particular mathematical operation.
Encourage your students to:
Read and reread the question
They say they’ve read it, but have they really ? Sometimes students will skip ahead as soon as they’ve noticed one familiar piece of information or give up trying to understand it if the problem doesn’t make sense at first glance.
Teach students to interpret a question by using self-monitoring strategies such as:
- Rereading a question more slowly if it doesn’t make sense the first time
- Asking for help
- Highlighting or underlining important pieces of information.
Identify important and extraneous information
John is collecting money for his friend Ari’s birthday. He starts with $5 of his own, then Marcus gives him another $5. How much does he have now?
As adults looking at the above problem, we can instantly look past the names and the birthday scenario to see a simple addition problem. Students, however, can struggle to determine what’s relevant in the information that’s been given to them.
Teach students to sort and sift the information in a problem to find what’s relevant. A good way to do this is to have them swap out pieces of information to see if the solution changes. If changing names, items or scenarios has no impact on the end result, they’ll realize that it doesn’t need to be a point of focus while solving the problem.
Schema approach
This is a math intervention strategy that can make problem solving easier for all students, regardless of ability.
Compare different word problems of the same type and construct a formula, or mathematical sentence stem, that applies to them all. For example, a simple subtraction problems could be expressed as:
[Number/Quantity A] with [Number/Quantity B] removed becomes [end result].
This is the underlying procedure or schema students are being asked to use. Once they have a list of schema for different mathematical operations (addition, multiplication and so on), they can take turns to apply them to an unfamiliar word problem and see which one fits.
Struggling students often believe math is something you either do automatically or don’t do at all. But that’s not true. Help your students understand that they have a choice of problem-solving strategies to use, and if one doesn’t work, they can try another.
Here are four common strategies students can use for problem solving.
Visualizing
Visualizing an abstract problem often makes it easier to solve. Students could draw a picture or simply draw tally marks on a piece of working out paper.
Encourage visualization by modeling it on the whiteboard and providing graphic organizers that have space for students to draw before they write down the final number.
Guess and check
Show students how to make an educated guess and then plug this answer back into the original problem. If it doesn’t work, they can adjust their initial guess higher or lower accordingly.
Find a pattern
To find patterns, show students how to extract and list all the relevant facts in a problem so they can be easily compared. If they find a pattern, they’ll be able to locate the missing piece of information.
Work backward
Working backward is useful if students are tasked with finding an unknown number in a problem or mathematical sentence. For example, if the problem is 8 + x = 12, students can find x by:
- Starting with 12
- Taking the 8 from the 12
- Being left with 4
- Checking that 4 works when used instead of x
Now students have understood the problem and formulated a strategy, it’s time to put it into practice. But if they just launch in and do it, they might make it harder for themselves. Show them how to work through a problem effectively by:

Documenting working out
Model the process of writing down every step you take to complete a math problem and provide working out paper when students are solving a problem. This will allow students to keep track of their thoughts and pick up errors before they reach a final solution.
Check along the way
Checking work as you go is another crucial self-monitoring strategy for math learners. Model it to them with think aloud questions such as:
- Does that last step look right?
- Does this follow on from the step I took before?
- Have I done any ‘smaller’ sums within the bigger problem that need checking?
Students often make the mistake of thinking that speed is everything in math — so they’ll rush to get an answer down and move on without checking.
But checking is important too. It allows them to pinpoint areas of difficulty as they come up, and it enables them to tackle more complex problems that require multiple checks before arriving at a final answer.
Here are some checking strategies you can promote:
Check with a partner
Comparing answers with a peer leads is a more reflective process than just receiving a tick from the teacher. If students have two different answers, encourage them to talk about how they arrived at them and compare working out methods. They’ll figure out exactly where they went wrong, and what they got right.
Reread the problem with your solution
Most of the time, students will be able to tell whether or not their answer is correct by putting it back into the initial problem. If it doesn’t work or it just ‘looks wrong’, it’s time to go back and fix it up.
Fixing mistakes
Show students how to backtrack through their working out to find the exact point where they made a mistake. Emphasize that they can’t do this if they haven’t written down everything in the first place — so a single answer with no working out isn’t as impressive as they might think!
Need more help developing problem solving skills?
Read up on how to set a problem solving and reasoning activity or explore Mathseeds and Mathletics, our award winning online math programs. They’ve got over 900 teacher tested problem solving activities between them!
Get access to 900+ unique problem solving activities
You might like....
Login to your account
Change password, your password must have 8 characters or more and contain 3 of the following:.
- a lower case character,
- an upper case character,
- a special character
Password Changed Successfully
Your password has been changed
Create a new account
Can't sign in? Forgot your password?
Enter your email address below and we will send you the reset instructions
If the address matches an existing account you will receive an email with instructions to reset your password
Request Username
Can't sign in? Forgot your username?
Enter your email address below and we will send you your username
If the address matches an existing account you will receive an email with instructions to retrieve your username

- Institutional Access
Cookies Notification
Our site uses Javascript to enchance its usability. You can disable your ad blocker or whitelist our website www.worldscientific.com to view the full content.
Select your blocker:, adblock plus instructions.
- Click the AdBlock Plus icon in the extension bar
- Click the blue power button
- Click refresh
Adblock Instructions
- Click the AdBlock icon
- Click "Don't run on pages on this site"
uBlock Origin Instructions
- Click on the uBlock Origin icon in the extension bar
- Click on the big, blue power button
- Refresh the web page
uBlock Instructions
- Click on the uBlock icon in the extension bar
Adguard Instructions
- Click on the Adguard icon in the extension bar
- Click on the toggle next to the "Protection on this website" text
Brave Instructions
- Click on the orange lion icon to the right of the address bar
- Click the toggle on the top right, shifting from "Up" to "Down
Adremover Instructions
- Click on the AdRemover icon in the extension bar
- Click the "Don’t run on pages on this domain" button
- Click "Exclude"
Adblock Genesis Instructions
- Click on the Adblock Genesis icon in the extension bar
- Click on the button that says "Whitelist Website"
Super Adblocker Instructions
- Click on the Super Adblocker icon in the extension bar
- Click on the "Don’t run on pages on this domain" button
- Click the "Exclude" button on the pop-up
Ultrablock Instructions
- Click on the UltraBlock icon in the extension bar
- Click on the "Disable UltraBlock for ‘domain name here’" button
Ad Aware Instructions
- Click on the AdAware icon in the extension bar
- Click on the large orange power button
Ghostery Instructions
- Click on the Ghostery icon in the extension bar
- Click on the "Trust Site" button
Firefox Tracking Protection Instructions
- Click on the shield icon on the left side of the address bar
- Click on the toggle that says "Enhanced Tracking protection is ON for this site"
Duck Duck Go Instructions
- Click on the DuckDuckGo icon in the extension bar
- Click on the toggle next to the words "Site Privacy Protection"
Privacy Badger Instructions
- Click on the Privacy Badger icon in the extension bar
- Click on the button that says "Disable Privacy Badger for this site"
Disconnect Instructions
- Click on the Disconnect icon in the extension bar
- Click the button that says "Whitelist Site"
Opera Instructions
- Click on the blue shield icon on the right side of the address bar
- Click the toggle next to "Ads are blocked on this site"
System Upgrade on Tue, May 28th, 2024 at 2am (EDT)

Problem Solving in Mathematics and Beyond: Volume 1
Problem-solving strategies in mathematics.
- By (author):
- Alfred S Posamentier ( Mercy College New York, USA ) and
- Stephen Krulik ( Temple University, USA )
- Add to favorites
- Download Citations
- Track Citations
- Recommend to Library
- Description
- Supplementary
"This is a wonderful book, filled with problems and witty solutions, addressed to anyone interested in problem-solving. Elementary mathematics is used so that the reader can focus on the strategy. The reader will enjoy reading this book and trying to find their own strategies to arrive at the solution of the problems proposed."
This book introduces ten problem-solving strategies by first presenting the strategy and then applying it to problems in elementary mathematics. In doing so, first the common approach is shown, and then a more elegant strategy is provided. Elementary mathematics is used so that the reader can focus on the strategy and not be distracted by some more sophisticated mathematics.
Errata(s) Errata
Sample Chapter(s) Introduction (71 KB) Chapter 1: Logical Reasoning (79 KB)
Request Inspection Copy
- Logical Reasoning
- Pattern Recognition
- Working Backwards
- Adopting a Different Point of View
- Considering Extreme Cases
- Solving a Simpler Analgous Problem
- Organizing Data
- Making a Drawing or Visual Representation
- Accounting for All Possibilities
- Intelligent Guessing and Testing
FRONT MATTER
- Pages: i–xxiii
https://doi.org/10.1142/9789814651646_fmatter
- About the Authors
- Introduction
Chapter 1: Logical Reasoning
- Pages: 1–12
https://doi.org/10.1142/9789814651646_0001
It must seem rather redundant to have a chapter entirely devoted to a strategy referred to as logical reasoning. Regardless of the strategy being used to solve a problem, it would seem that some logical thinking must permeate the use of all the strategies. After all, to many people, problem solving is almost synonymous with logical reasoning, or logical thought. So, why have this chapter and why isolate this strategy…
Chapter 2: Pattern Recognition
- Pages: 13–28
https://doi.org/10.1142/9789814651646_0002
Some of the most inherent beauties of mathematics are the many patterns that occur in problems as we try to resolve them. The famous mathematician, W.W. Sawyer once said that mathematics could be thought of as a search for patterns. One of the major uses of mathematics is to predict things that occur in a regular manner. For example, how many scones will I need for 3 people? For 4 people? For 10 people? For n people…
Chapter 3: Working Backwards
- Pages: 29–43
https://doi.org/10.1142/9789814651646_0003
The very name of this strategy sounds confusing to most people. It is a very unnatural way of doing things. When most of us went to school, we were taught to solve mathematical problems in a direct, straightforward manner. And yet, working backwards is the way many real-life problems are often resolved. As a simple example, suppose you had to pick up your child from football practice at exactly 5:00 p.m. At what time should you leave? Well, let us say it take 30 minutes to get to the ballpark. We would better leave a 5-minute safety valve. Okay, then we need to leave 35 minutes earlier, or not later than 4:25 p.m. Without even thinking about it, we were working backwards! Of course, this is a very simple example of this strategy…
Chapter 4: Adopting a Different Point of View
- Pages: 45–63
https://doi.org/10.1142/9789814651646_0004
Of the many strategies that exist and that are available to us to solve mathematical problems, the one that allows us to avoid “running into a brick wall” — namely avoiding frustration — is that of approaching the problem from a different point of view. Perhaps one of the classic examples — because of its simplicity and dramatic difference in solution method — is the following. This is an example where the common method leads to a correct answer, but is cumbersome and can often provide an opportunity for some arithmetic mistakes. As an example, consider the following problem…
Chapter 5: Considering Extreme Cases
- Pages: 65–82
https://doi.org/10.1142/9789814651646_0005
In order to obtain some help with solving a particular problem, we may consider some variables at their extremes, while holding others constant. If no specificity for the variable has been prescribed, the extreme case might just provide us with some helpful insight. Most of us have used this strategy in real life, without consciously realizing that we were even doing so. We say to ourselves, “What is the worst that can happen?” — for a particular, situation up for a decision. This “worst-case scenario” is an example of using an extreme case that can sometimes help resolve a problem situation in a very clever way. Similarly, suppose you were asked to test a new product, say, laundry soap. You would have to test it in very cold water as well as very hot water — clearly a consideration of two extreme cases that makes the testing worthwhile. If it does well in these two extreme temperatures, it should do well in the temperatures in between…
Chapter 6: Solving a Simpler Analgous Problem
- Pages: 83–95
https://doi.org/10.1142/9789814651646_0006
There are problems that appear to be extremely complex when we first approach them. The numbers in the problem might be extremely large, and thus confusing or possibly distracting. Perhaps there is an excessive amount of data given, some of which may not even be necessary to solve the given problem. Even the way the problem has been presented can sometimes confuse the reader. Regardless of the reason, an excellent approach is to convert the problem into a simpler form, but as an equivalent version of the original. Change the numbers, modify the original drawing, or try another approach to simply alter the form of the problem. By solving this simplified version of the problem, the problem solver can possibly gain some insight into how to approach the original problem…
Chapter 7: Organizing Data
- Pages: 97–113
https://doi.org/10.1142/9789814651646_0007
The strategy of organizing data is one of the most important strategies, even though at first glance we might assume it to be axiomatic. That is, everyone should automatically organize the data that appears in a problem without giving it a moment's thought. It is what we often do in real life without consciously thinking about it…
Chapter 8: Making a Drawing or Visual Representation
- Pages: 115–130
https://doi.org/10.1142/9789814651646_0008
When a problem asks questions about a particular given geometric figure or drawing, it goes without saying that the drawing or visual representation is an integral part of the solution method. It is necessary, and helps to solve the problem. In that sense it is hard to imagine that some mathematicians in ancient times often derived geometric concepts without drawings — or at least they presented their geometric findings without the appropriate sketch. However, there are many problems where a drawing is not implicit in the statement of the problem; yet, actually seeing what is being considered can be of great help. Many people are visual learners; they need a picture rather than merely words to understand what is taking place. And no, it is not a case of daydreaming. Contrary to what some people believe, visualization has nothing to do with daydreaming. While daydreaming is often a waste of time, visualization is a very powerful method that can help you become more familiar with a given situation…
Chapter 9: Accounting for All Possibilities
- Pages: 131–143
https://doi.org/10.1142/9789814651646_0009
We know that organizing the data in a problem can sometimes be very revealing. When we look for a pattern, for example, carefully organized data in a list or table can aid in the search and help us discover the pattern. However, one particular type of list that is extremely important is the exhaustive list. In this kind of organized list, all the possibilities are listed in some systematic way. Somewhere included on this list will be what we are looking for. Constructing an exhaustive list enables us to examine all the possibilities in a carefully-organized manner…
Chapter 10: Intelligent Guessing and Testing
- Pages: 145–159
https://doi.org/10.1142/9789814651646_0010
Somehow, the very idea of guessing as a problem-solving strategy raises some eyebrows. Indeed, many of us can recall a teacher saying to someone who had volunteered an unusual answer, “Do you know , or are you just guessing? ” In some books, this method of guessing and testing is sometimes referred to as the method of “trial and error,” which is sometimes considered a more negative expression. The added adjective, intelligent guessing and testing should put your mind at ease, and reassure you that this is, indeed, a viable, and even an often useful strategy…
BACK MATTER
- Pages: 161–163
https://doi.org/10.1142/9789814651646_bmatter
"I recommend this book to anyone interested in furthering his or her understanding of problem-solving strategies. I would encourage those individuals to read the book, become familiar with the ten strategies, and then spend some time working through the given problems before reviewing the solutions provided because it will most likely offer some new insight and thinking into the problem."
"The problems presented here could be used by teachers to help their students develop problem-solving skills, by students interested in developing their own skills, or by anybody intrigued by mathematics."
"Many of the problems or problem types will be familiar to Gazette readers and some are quite similar to one another. They are attractively presented in a clear, unfussy manner."

Related Books

Solving Problems in Our Spatial World

How Chinese Learn Mathematics

The Magic Garden of George B and Other Logic Puzzles

Connections

Continued Fractions

Reflections

The KAM Story

Experiments in Undergraduate Mathematics
Primary mathematics standards for pre-service teachers in chile.

Venusian Casino Junkets

Number Theory

Fields Medallists' Lectures

Strategy Games to Enhance Problem-Solving Ability in Mathematics

Indian Mathematics

Algebra for Parents

Geometric Inequalities

Jim Totten's Problems of the Week

Origami with Explanations

A Central European Olympiad
Weekly dose of self-improvement
The easy 4 step problem-solving process (+ examples)
This is the 4 step problem-solving process that I taught to my students for math problems, but it works for academic and social problems as well.

Every problem may be different, but effective problem solving asks the same four questions and follows the same method.
- What’s the problem? If you don’t know exactly what the problem is, you can’t come up with possible solutions. Something is wrong. What are we going to do about this? This is the foundation and the motivation.
- What do you need to know? This is the most important part of the problem. If you don’t know exactly what the problem is, you can’t come up with possible solutions.
- What do you already know? You already know something related to the problem that will help you solve the problem. It’s not always obvious (especially in the real world), but you know (or can research) something that will help.
- What’s the relationship between the two? Here is where the heavy brainstorming happens. This is where your skills and abilities come into play. The previous steps set you up to find many potential solutions to your problem, regardless of its type.
When I used to tutor kids in math and physics , I would drill this problem-solving process into their heads. This methodology works for any problem, regardless of its complexity or difficulty. In fact, if you look at the various advances in society, you’ll see they all follow some variation of this problem-solving technique.
“The gap between understanding and misunderstanding can best be bridged by thought!” ― Ernest Agyemang Yeboah
Generally speaking, if you can’t solve the problem then your issue is step 3 or step 4; you either don’t know enough or you’re missing the connection.
Good problem solvers always believe step 3 is the issue. In this case, it’s a simple matter of learning more. Less skilled problem solvers believe step 4 is the root cause of their difficulties. In this instance, they simply believe they have limited problem-solving skills.
This is a fixed versus growth mindset and it makes a huge difference in the effort you put forth and the belief you have in yourself to make use of this step-by-step process. These two mindsets make a big difference in your learning because, at its core, learning is problem-solving.
Let’s dig deeper into the 4 steps. In this way, you can better see how to apply them to your learning journey.
Step 1: What’s the problem?
The ability to recognize a specific problem is extremely valuable.
Most people only focus on finding solutions. While a “solutions-oriented” mindset is a good thing, sometimes it pays to focus on the problem. When you focus on the problem, you often make it easier to find a viable solution to it.
When you know the exact nature of the problem, you shorten the time frame needed to find a solution. This reminds me of a story I was once told.
When does the problem-solving process start?
The process starts after you’ve identified the exact nature of the problem.
Homeowners love a well-kept lawn but hate mowing the grass.
Many companies and inventors raced to figure out a more time-efficient way to mow the lawn. Some even tried to design robots that would do the mowing. They all were chasing the solution, but only one inventor took the time to understand the root cause of the problem.
Most people figured that the problem was the labor required to maintain a lawn. The actual problem was just the opposite: maintaining a lawn was labor-intensive. The rearrangement seems trivial, but it reveals the true desire: a well-maintained lawn.
The best solution? Remove maintenance from the equation. A lawn made of artificial grass solved the problem . Hence, an application of Astroturf was discovered.
This way, the law always looked its best. Taking a few moments to apply critical thinking identified the true nature of the problem and yielded a powerful solution.
An example of choosing the right problem to work the problem-solving process on
One thing I’ve learned from tutoring high school students in math : they hate word problems.
This is because they make the student figure out the problem. Finding the solution to a math problem is already stressful. Forcing the student to also figure out what problem needs solving is another level of hell.
Word problems are not always clear about what needs to be solved. They also have the annoying habit of adding extraneous information. An ordinary math problem does not do this. For example, compare the following two problems:
What’s the height of h?
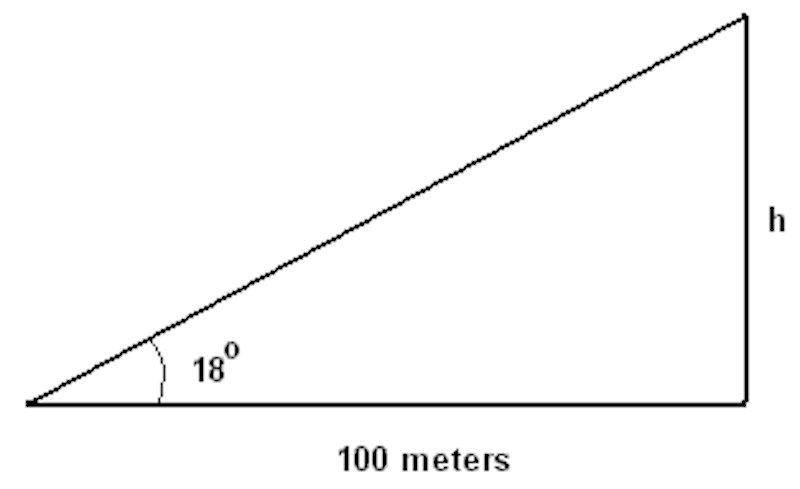
A radio station tower was built in two sections. From a point 87 feet from the base of the tower, the angle of elevation of the top of the first section is 25º, and the angle of elevation of the top of the second section is 40º. To the nearest foot, what is the height of the top section of the tower?
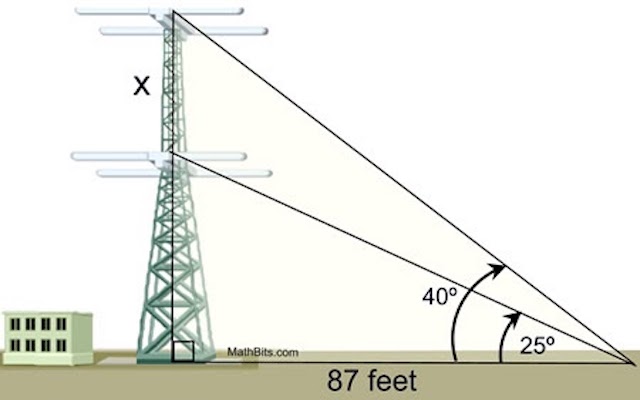
The first is a simple problem. The second is a complex problem. The end goal in both is the same.
The questions require the same knowledge (trigonometric functions), but the second is more difficult for students. Why? The second problem does not make it clear what the exact problem is. Before mathematics can even begin, you must know the problem, or else you risk solving the wrong one.
If you understand the problem, finding the solution is much easier. Understanding this, ironically, is the biggest problem for people.
Problem-solving is a universal language
Speaking of people, this method also helps settle disagreements.
When we disagree, we rarely take the time to figure out the exact issue. This happens for many reasons, but it always results in a misunderstanding. When each party is clear with their intentions, they can generate the best response.
Education systems fail when they don’t consider the problem they’re supposed to solve. Foreign language education in America is one of the best examples.
The problem is that students can’t speak the target language. It seems obvious that the solution is to have students spend most of their time speaking. Unfortunately, language classes spend a ridiculous amount of time learning grammar rules and memorizing vocabulary.
The problem is not that the students don’t know the imperfect past tense verb conjugations in Spanish. The problem is that they can’t use the language to accomplish anything. Every year, kids graduate from American high schools without the ability to speak another language, despite studying one for 4 years.
Well begun is half done
Before you begin to learn something, be sure that you understand the exact nature of the problem. This will make clear what you need to know and what you can discard. When you know the exact problem you’re tasked with solving, you save precious time and energy. Doing this increases the likelihood that you’ll succeed.
Step 2: What do you need to know?
All problems are the result of insufficient knowledge. To solve the problem, you must identify what you need to know. You must understand the cause of the problem. If you get this wrong, you won’t arrive at the correct solution.
Either you’ll solve what you thought was the problem, only to find out this wasn’t the real issue and now you’ve still got trouble or you won’t and you still have trouble. Either way, the problem persists.
If you solve a different problem than the correct one, you’ll get a solution that you can’t use. The only thing that wastes more time than an unsolved problem is solving the wrong one.
Imagine that your car won’t start. You replace the alternator, the starter, and the ignition switch. The car still doesn’t start. You’ve explored all the main solutions, so now you consider some different solutions.
Now you replace the engine, but you still can’t get it to start. Your replacements and repairs solved other problems, but not the main one: the car won’t start.
Then it turns out that all you needed was gas.
This example is a little extreme, but I hope it makes the point. For something more relatable, let’s return to the problem with language learning.
You need basic communication to navigate a foreign country you’re visiting; let’s say Mexico. When you enroll in a Spanish course, they teach you a bunch of unimportant words and phrases. You stick with it, believing it will eventually click.
When you land, you can tell everyone your name and ask for the location of the bathroom. This does not help when you need to ask for directions or tell the driver which airport terminal to drop you off at.
Finding the solution to chess problems works the same way
The book “The Amateur Mind” by IM Jeremy Silman improved my chess by teaching me how to analyze the board.
It’s only with a proper analysis of imbalances that you can make the best move. Though you may not always choose the correct line of play, the book teaches you how to recognize what you need to know . It teaches you how to identify the problem—before you create an action plan to solve it.

The problem-solving method always starts with identifying the problem or asking “What do you need to know?”. It’s only after you brainstorm this that you can move on to the next step.
Learn the method I used to earn a physics degree, learn Spanish, and win a national boxing title
- I was a terrible math student in high school who wrote off mathematics. I eventually overcame my difficulties and went on to earn a B.A. Physics with a minor in math
- I pieced together the best works on the internet to teach myself Spanish as an adult
- *I didn’t start boxing until the very old age of 22, yet I went on to win a national championship, get a high-paying amateur sponsorship, and get signed by Roc Nation Sports as a profession.
I’ve used this method to progress in mentally and physically demanding domains.
While the specifics may differ, I believe that the general methods for learning are the same in all domains.
This free e-book breaks down the most important techniques I’ve used for learning.
Step 3: What do you already know?
The only way to know if you lack knowledge is by gaining some in the first place. All advances and solutions arise from the accumulation and implementation of prior information. You must first consider what it is that you already know in the context of the problem at hand.
Isaac Newton once said, “If I have seen further, it is by standing on the shoulders of giants.” This is Newton’s way of explaining that his advancements in physics and mathematics would be impossible if it were not for previous discoveries.
Mathematics is a great place to see this idea at work. Consider the following problem:
What is the domain and range of y=(x^2)+6?
This simple algebra problem relies on you knowing a few things already. You must know:
- The definition of “domain” and “range”
- That you can never square any real number and get a negative
Once you know those things, this becomes easy to solve. This is also how we learn languages.
An example of the problem-solving process with a foreign language
Anyone interested in serious foreign language study (as opposed to a “crash course” or “survival course”) should learn the infinitive form of verbs in their target language. You can’t make progress without them because they’re the root of all conjugations. It’s only once you have a grasp of the infinitives that you can completely express yourself. Consider the problem-solving steps applied in the following example.
I know that I want to say “I don’t eat eggs” to my Mexican waiter. That’s the problem.
I don’t know how to say that, but last night I told my date “No bebo alcohol” (“I don’t drink alcohol”). I also know the infinitive for “eat” in Spanish (comer). This is what I already know.
Now I can execute the final step of problem-solving.
Step 4: What’s the relationship between the two?
I see the connection. I can use all of my problem-solving strategies and methods to solve my particular problem.
I know the infinitive for the Spanish word “drink” is “beber” . Last night, I changed it to “bebo” to express a similar idea. I should be able to do the same thing to the word for “eat”.
“No como huevos” is a pretty accurate guess.
In the math example, the same process occurs. You don’t know the answer to “What is the domain and range of y=(x^2)+6?” You only know what “domain” and “range” mean and that negatives aren’t possible when you square a real number.
A domain of all real numbers and a range of all numbers equal to and greater than six is the answer.
This is relating what you don’t know to what you already do know. The solutions appear simple, but walking through them is an excellent demonstration of the process of problem-solving.
In most cases, the solution won’t be this simple, but the process or finding it is the same. This may seem trivial, but this is a model for thinking that has served the greatest minds in history.
A recap of the 4 steps of the simple problem-solving process
- What’s the problem? There’s something wrong. There’s something amiss.
- What do you need to know? This is how to fix what’s wrong.
- What do you already know? You already know something useful that will help you find an effective solution.
- What’s the relationship between the previous two? When you use what you know to help figure out what you don’t know, there is no problem that won’t yield.
Learning is simply problem-solving. You’ll learn faster if you view it this way.
What was once complicated will become simple.
What was once convoluted will become clear.

Ed Latimore
I’m a writer, competitive chess player, Army veteran, physicist, and former professional heavyweight boxer. My work focuses on self-development, realizing your potential, and sobriety—speaking from personal experience, having overcome both poverty and addiction.
Follow me on Twitter.

Pimsleur language system review—old but still good
The Pimsleur language program offers a framework you can use to learn a language. I’ve used the program. Here are my experiences.

Pimsleur vs Duolingo: Choosing the best language learning app
Pimsleur and Duolingo are two popular language-learning apps to help you learn a new language. This guide will help you decide which app will work best for you.

My Chess.com Chess Improvement Plan
This is a plan that I’ll be implementing to improve my rating on Chess.com in the rapid and daily games. Not for OTB but could work.
Four-Step Math Problem Solving Strategies & Techniques
- Harlan Bengtson
- Categories : Help with math homework
- Tags : Homework help & study guides
Four Steps to Success
There are many possible strategies and techniques you can use to solve math problems. A useful starting point is a four step approach to math problem solving. These four steps can be summarized as follows:
- Carefully read the problem. In this careful reading, you should especially seek to clearly identify the question that is to be answered. Also, a good, general understanding of what the problem means should be sought.
- Choose a strategy to solve the problem. Some of the possible strategies will be discussed in the rest of this article.
- Carry out the problem solving strategy. If the first problem solving technique you try doesn’t work, try another.
- Check the solution. This check should make sure that you have indeed answered the question that was posed and that the answer makes sense.
Step One - Understanding the Problem
As you carefully read the problem, trying to clearly understand the meaning of the problem and the question that you must answer, here are some techniques to help.
Identify given information - Highlighting or underlining facts that are given helps to visualize what is known or given.
Identify information asked for - Highlighting the unknowns in a different color helps to keep the known information visually separate from the unknowns to be determined. Ideally this will lead to a clear identification of the question to be answered.
Look for keywords or clue words - One example of clue words is those that indicate what type of mathematical operation is needed, as follows:
Clue words indicating addition: sum, total, in all, perimeter.
Clue words indicating subtraction: difference, how much more, exceed.
Clue words for multiplication: product, total, area, times.
Clue words for division: share, distribute, quotient, average.
Draw a picture - This might also be considered part of solving the problem, but a good sketch showing given information and unknowns can be very helpful in understanding the problem.
Step Two - Choose the Right Strategy
It step one has been done well, it should ease the job of choosing among the strategies presented here for approaching the problem solving step. Here are some of the many possible math problem solving strategies.
- Look for a pattern - This might be part of understanding the problem or it might be the first part of solving the problem.
- Make an organized list - This is another means of organizing the information as part of understanding it or beginning the solution.
- Make a table - In some cases the problem information may be more suitable for putting in a table rather than in a list.
- Try to remember if you’ve done a similar problem before - If you have done a similar problem before, try to use the same approach that worked in the past for the solution.
- Guess the answer - This may seem like a haphazard approach, but if you then check whether your guess was correct, and repeat as many times as necessary until you find the right answer, it works very well. Often information from checking on whether the answer was correct helps lead you to a good next guess.
- Work backwards - Sometimes making the calculations in the reverse order works better.
Steps Three and Four - Solving the Problem and Checking the Solution
If the first two steps have been done well, then the last two steps should be easy. If the selected problem solving strategy doesn’t seem to work when you actually try it, go back to the list and try something else. Your check on the solution should show that you have actually answered the question that was asked in the problem, and to the extent possible, you should check on whether the answer makes common sense.
If you're seeing this message, it means we're having trouble loading external resources on our website.
If you're behind a web filter, please make sure that the domains *.kastatic.org and *.kasandbox.org are unblocked.
To log in and use all the features of Khan Academy, please enable JavaScript in your browser.
Unit 1: Algebra foundations
Unit 2: solving equations & inequalities, unit 3: working with units, unit 4: linear equations & graphs, unit 5: forms of linear equations, unit 6: systems of equations, unit 7: inequalities (systems & graphs), unit 8: functions, unit 9: sequences, unit 10: absolute value & piecewise functions, unit 11: exponents & radicals, unit 12: exponential growth & decay, unit 13: quadratics: multiplying & factoring, unit 14: quadratic functions & equations, unit 15: irrational numbers, unit 16: creativity in algebra.
- Solve equations and inequalities
- Simplify expressions
- Factor polynomials
- Graph equations and inequalities
- Advanced solvers
- All solvers
- Arithmetics
- Determinant
- Percentages
- Scientific Notation
- Inequalities
What can QuickMath do?
QuickMath will automatically answer the most common problems in algebra, equations and calculus faced by high-school and college students.
- The algebra section allows you to expand, factor or simplify virtually any expression you choose. It also has commands for splitting fractions into partial fractions, combining several fractions into one and cancelling common factors within a fraction.
- The equations section lets you solve an equation or system of equations. You can usually find the exact answer or, if necessary, a numerical answer to almost any accuracy you require.
- The inequalities section lets you solve an inequality or a system of inequalities for a single variable. You can also plot inequalities in two variables.
- The calculus section will carry out differentiation as well as definite and indefinite integration.
- The matrices section contains commands for the arithmetic manipulation of matrices.
- The graphs section contains commands for plotting equations and inequalities.
- The numbers section has a percentages command for explaining the most common types of percentage problems and a section for dealing with scientific notation.
Math Topics
More solvers.
- Add Fractions
- Simplify Fractions

Think back to the first problem in this chapter, the ABC Problem . What did you do to solve it? Even if you did not figure it out completely by yourself, you probably worked towards a solution and figured out some things that did not work.
Unlike exercises, there is never a simple recipe for solving a problem. You can get better and better at solving problems, both by building up your background knowledge and by simply practicing. As you solve more problems (and learn how other people solved them), you learn strategies and techniques that can be useful. But no single strategy works every time.
Pólya’s How to Solve It
George Pólya was a great champion in the field of teaching effective problem solving skills. He was born in Hungary in 1887, received his Ph.D. at the University of Budapest, and was a professor at Stanford University (among other universities). He wrote many mathematical papers along with three books, most famously, “How to Solve it.” Pólya died at the age 98 in 1985. [1]
In 1945, Pólya published the short book How to Solve It , which gave a four-step method for solving mathematical problems:
- Understand the problem.
- Devise a plan.
- Carry out the plan.
- Looking back.
This is all well and good, but how do you actually do these steps?!?! Steps 1. and 2. are particularly mysterious! How do you “make a plan?” That is where you need some tools in your toolbox, and some experience to draw upon.
Much has been written since 1945 to explain these steps in more detail, but the truth is that they are more art than science. This is where math becomes a creative endeavor (and where it becomes so much fun). We will articulate some useful problem solving strategies, but no such list will ever be complete. This is really just a start to help you on your way. The best way to become a skilled problem solver is to learn the background material well, and then to solve a lot of problems!
We have already seen one problem solving strategy, which we call “Wishful Thinking.” Do not be afraid to change the problem! Ask yourself “what if” questions:
- What if the picture was different?
- What if the numbers were simpler?
- What if I just made up some numbers?
You need to be sure to go back to the original problem at the end, but wishful thinking can be a powerful strategy for getting started.
This brings us to the most important problem solving strategy of all:
Problem Solving Strategy 2 (Try Something!). If you are really trying to solve a problem, the whole point is that you do not know what to do right out of the starting gate. You need to just try something! Put pencil to paper (or stylus to screen or chalk to board or whatever!) and try something. This is often an important step in understanding the problem; just mess around with it a bit to understand the situation and figure out what is going on.
And equally important: If what you tried first does not work, try something else! Play around with the problem until you have a feel for what is going on.
Problem 2 (Payback)
Last week, Alex borrowed money from several of his friends. He finally got paid at work, so he brought cash to school to pay back his debts. First he saw Brianna, and he gave her 1/4 of the money he had brought to school. Then Alex saw Chris and gave him 1/3 of what he had left after paying Brianna. Finally, Alex saw David and gave him 1/2 of what he had remaining. Who got the most money from Alex?
Think/Pair/Share
After you have worked on the problem on your own for a while, talk through your ideas with a partner (even if you have not solved it). What did you try? What did you figure out about the problem?
This problem lends itself to two particular strategies. Did you try either of these as you worked on the problem? If not, read about the strategy and then try it out before watching the solution.
Problem Solving Strategy 3 (Draw a Picture). Some problems are obviously about a geometric situation, and it is clear you want to draw a picture and mark down all of the given information before you try to solve it. But even for a problem that is not geometric, like this one, thinking visually can help! Can you represent something in the situation by a picture?
Draw a square to represent all of Alex’s money. Then shade 1/4 of the square — that’s what he gave away to Brianna. How can the picture help you finish the problem?
After you have worked on the problem yourself using this strategy (or if you are completely stuck), you can watch someone else’s solution.
Problem Solving Strategy 4 (Make Up Numbers). Part of what makes this problem difficult is that it is about money, but there are no numbers given. That means the numbers must not be important. So just make them up!
You can work forwards: Assume Alex had some specific amount of money when he showed up at school, say $100. Then figure out how much he gives to each person. Or you can work backwards: suppose he has some specific amount left at the end, like $10. Since he gave Chris half of what he had left, that means he had $20 before running into Chris. Now, work backwards and figure out how much each person got.
Watch the solution only after you tried this strategy for yourself.
If you use the “Make Up Numbers” strategy, it is really important to remember what the original problem was asking! You do not want to answer something like “Everyone got $10.” That is not true in the original problem; that is an artifact of the numbers you made up. So after you work everything out, be sure to re-read the problem and answer what was asked!
Problem 3 (Squares on a Chess Board)
How many squares, of any possible size, are on a 8 × 8 chess board? (The answer is not 64… It’s a lot bigger!)
Remember Pólya’s first step is to understand the problem. If you are not sure what is being asked, or why the answer is not just 64, be sure to ask someone!
Think / Pair / Share
After you have worked on the problem on your own for a while, talk through your ideas with a partner (even if you have not solved it). What did you try? What did you figure out about the problem, even if you have not solved it completely?
It is clear that you want to draw a picture for this problem, but even with the picture it can be hard to know if you have found the correct answer. The numbers get big, and it can be hard to keep track of your work. Your goal at the end is to be absolutely positive that you found the right answer. You should never ask the teacher, “Is this right?” Instead, you should declare, “Here’s my answer, and here is why I know it is correct!”
Problem Solving Strategy 5 (Try a Simpler Problem). Pólya suggested this strategy: “If you can’t solve a problem, then there is an easier problem you can solve: find it.” He also said: “If you cannot solve the proposed problem, try to solve first some related problem. Could you imagine a more accessible related problem?” In this case, an 8 × 8 chess board is pretty big. Can you solve the problem for smaller boards? Like 1 × 1? 2 × 2? 3 × 3?
Of course the ultimate goal is to solve the original problem. But working with smaller boards might give you some insight and help you devise your plan (that is Pólya’s step (2)).
Problem Solving Strategy 6 (Work Systematically). If you are working on simpler problems, it is useful to keep track of what you have figured out and what changes as the problem gets more complicated.
For example, in this problem you might keep track of how many 1 × 1 squares are on each board, how many 2 × 2 squares on are each board, how many 3 × 3 squares are on each board, and so on. You could keep track of the information in a table:
| 1 | 0 | 0 | 0 | ||
| 4 | 1 | 0 | 0 | ||
| 9 | 4 | 1 | 0 | ||
Problem Solving Strategy 7 (Use Manipulatives to Help You Investigate). Sometimes even drawing a picture may not be enough to help you investigate a problem. Having actual materials that you move around can sometimes help a lot!
For example, in this problem it can be difficult to keep track of which squares you have already counted. You might want to cut out 1 × 1 squares, 2 × 2 squares, 3 × 3 squares, and so on. You can actually move the smaller squares across the chess board in a systematic way, making sure that you count everything once and do not count anything twice.
Problem Solving Strategy 8 (Look for and Explain Patterns). Sometimes the numbers in a problem are so big, there is no way you will actually count everything up by hand. For example, if the problem in this section were about a 100 × 100 chess board, you would not want to go through counting all the squares by hand! It would be much more appealing to find a pattern in the smaller boards and then extend that pattern to solve the problem for a 100 × 100 chess board just with a calculation.
If you have not done so already, extend the table above all the way to an 8 × 8 chess board, filling in all the rows and columns. Use your table to find the total number of squares in an 8 × 8 chess board. Then:
- Describe all of the patterns you see in the table.
- Can you explain and justify any of the patterns you see? How can you be sure they will continue?
- What calculation would you do to find the total number of squares on a 100 × 100 chess board?
(We will come back to this question soon. So if you are not sure right now how to explain and justify the patterns you found, that is OK.)
Problem 4 (Broken Clock)
This clock has been broken into three pieces. If you add the numbers in each piece, the sums are consecutive numbers. ( Consecutive numbers are whole numbers that appear one after the other, such as 1, 2, 3, 4 or 13, 14, 15.)
Can you break another clock into a different number of pieces so that the sums are consecutive numbers? Assume that each piece has at least two numbers and that no number is damaged (e.g. 12 isn’t split into two digits 1 and 2.)
Remember that your first step is to understand the problem. Work out what is going on here. What are the sums of the numbers on each piece? Are they consecutive?
After you have worked on the problem on your own for a while, talk through your ideas with a partner (even if you have not solved it). What did you try? What progress have you made?
Problem Solving Strategy 9 (Find the Math, Remove the Context). Sometimes the problem has a lot of details in it that are unimportant, or at least unimportant for getting started. The goal is to find the underlying math problem, then come back to the original question and see if you can solve it using the math.
In this case, worrying about the clock and exactly how the pieces break is less important than worrying about finding consecutive numbers that sum to the correct total. Ask yourself:
- What is the sum of all the numbers on the clock’s face?
- Can I find two consecutive numbers that give the correct sum? Or four consecutive numbers? Or some other amount?
- How do I know when I am done? When should I stop looking?
Of course, solving the question about consecutive numbers is not the same as solving the original problem. You have to go back and see if the clock can actually break apart so that each piece gives you one of those consecutive numbers. Maybe you can solve the math problem, but it does not translate into solving the clock problem.
Problem Solving Strategy 10 (Check Your Assumptions). When solving problems, it is easy to limit your thinking by adding extra assumptions that are not in the problem. Be sure you ask yourself: Am I constraining my thinking too much?
In the clock problem, because the first solution has the clock broken radially (all three pieces meet at the center, so it looks like slicing a pie), many people assume that is how the clock must break. But the problem does not require the clock to break radially. It might break into pieces like this:

Were you assuming the clock would break in a specific way? Try to solve the problem now, if you have not already.
- Image of Pólya by Thane Plambeck from Palo Alto, California (Flickr) [CC BY 2.0 (http://creativecommons.org/licenses/by/2.0)], via Wikimedia Commons ↵
Mathematics for Elementary Teachers Copyright © 2018 by Michelle Manes is licensed under a Creative Commons Attribution-ShareAlike 4.0 International License , except where otherwise noted.
Share This Book
Please ensure that your password is at least 8 characters and contains each of the following:
- a special character: @$#!%*?&

Get step-by-step solutions to your math problems

Try Math Solver

Get step-by-step explanations
Graph your math problems
Practice, practice, practice

Get math help in your language

Game Central

COMMENTS
Step 1: Understanding the problem. We are given in the problem that there are 25 chickens and cows. All together there are 76 feet. Chickens have 2 feet and cows have 4 feet. We are trying to determine how many cows and how many chickens Mr. Jones has on his farm. Step 2: Devise a plan.
Here are five strategies to help students check their solutions. 1. Use the Inverse Operation. For simpler problems, a quick and easy problem solving strategy is to use the inverse operation. For example, if the operation to solve a word problem is 56 ÷ 8 = 7 students can check the answer is correct by multiplying 8 × 7.
Teaching about problem solving begins with suggested strategies to solve a problem. For example, "draw a picture," "make a table," etc. You may see posters in teachers' classrooms of the "Problem Solving Method" such as: 1) Read the problem, 2) Devise a plan, 3) Solve the problem, and 4) Check your work. There is little or no ...
1.3 Digital Technologies and Mathematical Problem Solving—Luz Manuel Santos-Trigo. Mathematical problem solving is a field of research that focuses on analysing the extent to which problem solving activities play a crucial role in learners' understanding and use of mathematical knowledge.
Research focus, themes, and inquiry methods in the mathematical problem-solving agenda have varied and been influenced and shaped by theoretical and methodological developments of mathematics education as a discipline (English & Kirshner, 2016; Liljedahl & Cai, 2021).Further, research designs and methods used in cognitive, social, and computational fields have influenced the ways in which ...
In 1945, Pólya published the short book How to Solve It, which gave a four-step method for solving mathematical problems: First, you have to understand the problem. After understanding, then make a plan. Carry out the plan. Look back on your work.
The following video shows more examples of using problem solving strategies and models. Question 2: The table shows the number of seats in each of the first four rows in an auditorium. The remaining ten rows follow the same pattern. Find the number of seats in the last row. Question 3: You are hanging three pictures in the wall of your home ...
Overview. At its roots, problem solving is exactly what it sounds like, the process of solving problems. However, problem solving methods permeate the studies of mathematics, science, and technology. The human processes involved in problem solving are often studied by cognitive scientists .
A further characteristic is that a problem-solving approach can be used to encourage students to make generalisations about rules and concepts, a process which is central to mathematics (Evan and Lappin, 1994). Schoenfeld (in Olkin and Schoenfeld, 1994, p.43) described the way in which the use of problem solving in his teaching has changed ...
Problem-solving requires practice. When deciding on methods or procedures to use to solve problems, the first thing you will do is look for clues, which is one of the most important skills in solving problems in mathematics. If you begin to solve problems by looking for clue words, you will find that these words often indicate an operation.
The mathematician George Polya captured the problem solving principles and strategies he used in his discipline in the book How to Solve It: A New Aspect of Mathematical Method(Princeton University Press, 1957). The book includes a summary of Polya's problem solving heuristic as well as advice on the teaching of problem solving. Novices in a ...
Schema approach. This is a math intervention strategy that can make problem solving easier for all students, regardless of ability. Compare different word problems of the same type and construct a formula, or mathematical sentence stem, that applies to them all. For example, a simple subtraction problems could be expressed as:
Considering Extreme Cases. Solving a Simpler Analgous Problem. Organizing Data. Making a Drawing or Visual Representation. Accounting for All Possibilities. Intelligent Guessing and Testing. Readership: Undergraduates and general public interested in Mathematics. This book introduces ten problem-solving strategies by first presenting the ...
the studies showing how strategies can improve mathematics problem solving, Koichu, Berman, and Moore (2004) aimed to promote heuristic literacy in a regular mathematics classroom. Moreover, Dewey's (1933) "How we think", Polya's (1988) problem-solving methods and the stages of Krulik and
Consider the problem-solving steps applied in the following example. I know that I want to say "I don't eat eggs" to my Mexican waiter. That's the problem. I don't know how to say that, but last night I told my date "No bebo alcohol" ("I don't drink alcohol"). I also know the infinitive for "eat" in Spanish (comer).
Solving a math problem involves first gaining a clear understanding of the problem, then choosing from among problem solving techniques or strategies, followed by actually carrying out the solution, and finally checking the solution. See this article for more information about this four-step math problem solving procedure, with several problem solving techniques presented and discussed for ...
By the end of this course, students will be able to: discover the key findings from neuroscience and cognitive psychology to illuminate the mechanisms of human learning. analyze the characteristics of experts' knowledge to shape self-regulated learning. apply Pólya's Framework for Mathematical Problem Solving to a variety of Math problems.
Unit test. Level up on all the skills in this unit and collect up to 1,000 Mastery points! This unit tackles the medium-difficulty problem solving and data analysis questions on the SAT Math test. Work through each skill, taking quizzes and the unit test to level up your mastery progress.
Learn a powerful collection of methods for working with data! AP®️ Statistics is all about collecting, displaying, summarizing, interpreting, and making inferences from data. ... Problem solving with the coordinate plane: 5th grade (Eureka Math/EngageNY) ... The Mathematics 1 course, often taught in the 9th grade, covers Linear equations ...
The Algebra 1 course, often taught in the 9th grade, covers Linear equations, inequalities, functions, and graphs; Systems of equations and inequalities; Extension of the concept of a function; Exponential models; and Quadratic equations, functions, and graphs. Khan Academy's Algebra 1 course is built to deliver a comprehensive, illuminating, engaging, and Common Core aligned experience!
QuickMath will automatically answer the most common problems in algebra, equations and calculus faced by high-school and college students. The algebra section allows you to expand, factor or simplify virtually any expression you choose. It also has commands for splitting fractions into partial fractions, combining several fractions into one and ...
Problem Solving Strategy 9 (Find the Math, Remove the Context). Sometimes the problem has a lot of details in it that are unimportant, or at least unimportant for getting started. The goal is to find the underlying math problem, then come back to the original question and see if you can solve it using the math.
Free math problem solver answers your algebra homework questions with step-by-step explanations.
Get math help in your language. Works in Spanish, Hindi, German, and more. Online math solver with free step by step solutions to algebra, calculus, and other math problems. Get help on the web or with our math app.
Online math solver with free step by step solutions to algebra, calculus, and other math problems. Get help on the web or with our math app. ... Type a math problem. Examples. Quadratic equation { x } ^ { 2 } - 4 x - 5 = 0. Trigonometry. 4 \sin \theta \cos \theta = 2 \sin \theta. Linear equation ...
Although problem-solving is a skill in its own right, a subset of seven skills can help make the process of problem-solving easier. These include analysis, communication, emotional intelligence, resilience, creativity, adaptability, and teamwork. 1. Analysis. As a manager, you'll solve each problem by assessing the situation first.